Video Lectures
Theory For Notes Making
Relative Velocity In One Dimension
Every motion is relative as it has to be observed with respect to an observer. Relative velocity is a measurement of velocity of an object with respect to other observer. It is defined as the rate of change of relative position of one object w.r.t. to another with time
If an object A is moving with velocity vector \displaystyle \overrightarrow{{{{v}_{{A,G}}}}} ( velocity Of A with respect to ground ) and an object B with velocity \displaystyle \overrightarrow{{{{v}_{{B,G}}}}} ( velocity Of B with respect to ground ), then the velocity of object A relative to object B is given as
\displaystyle \overrightarrow{{{{v}_{{BA}}}}}\,=\,\overrightarrow{{{{v}_{{BG}}}}}\,-\,\overrightarrow{{{{v}_{{AG}}}}}
This can also be written simply as \displaystyle \overrightarrow{{{{v}_{{AB}}}}}\,=\,\overrightarrow{{{{v}_{A}}}}\,-\,\overrightarrow{{{{v}_{B}}}}
The relative velocity of object B relative to object A is
\displaystyle \overrightarrow{{{{v}_{{BA}}}}}\,=\,\overrightarrow{{{{v}_{{BG}}}}}\,-\,\overrightarrow{{{{v}_{{AG}}}}}
Where,
\displaystyle {{v}_{{Ag}}}\,=relative velocity of A with respect to ground.
\displaystyle {{v}_{{Bg}}}\,=relative velocity of B with respect to ground.
\displaystyle {{v}_{{Ag}}}\, and \displaystyle {{v}_{{Bg}}}\, could be written as \displaystyle {{v}_{A}}\, and \displaystyle {{v}_{B}}\, respectively.
Now, we will discuss different cases of relative velocity.
Case I :
If \displaystyle {{v}_{B}}={{v}_{A}},\,\,{{v}_{B}}-{{v}_{A}}=0, then the two objects A and B stay at a constant distance and their position-time graphs are parallel to each other. Thus, the relative velocity \displaystyle {{v}_{{AB}}} or \displaystyle {{v}_{{BA}}} is zero.
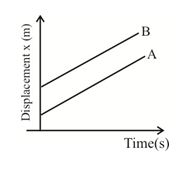
Case –II :
If \displaystyle {{v}_{A}}>{{v}_{B}},\,\,{{v}_{B}}-{{v}_{A}} is negative, then one graph is steeper than the other and they meet at a common point.
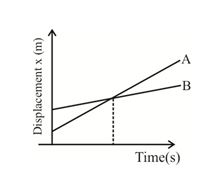
Case-III :
If \displaystyle {{v}_{A}} and \displaystyle {{v}_{B}} are of opposite signs, then the two objects meet at point. e.g., If the objects under consideration are two trains, then for a person sitting on either side, the other trans seem to go very fast.
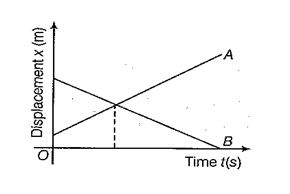
Case IV :
If both objects A and B move along parallel straight lines in the opposite direction, then relative velocity of B w.r.t. A is given as
\displaystyle {{v}_{{BA}}}={{v}_{B}}-\left( {-{{v}_{A}}} \right)
\displaystyle ={{v}_{B}}+{{v}_{A}}
and the relative velocity of A w.r.t. B is given by
\displaystyle {{v}_{{AB}}}\,=\,\left( {-{{v}_{B}}-{{v}_{A}}} \right)
Illustration
Two trains A and B of length 400m each are moving on two parallel tracks with a uniform speed of 72 \displaystyle km\,{{h}^{{-1}}} in the same direction with A ahead of B. The driver of B decides to overtake A and accelerates by 1 \displaystyle m{{s}^{{-2}}}. If after 50 s, the guard of B just brushes past the driver of A, what was the original distance between them ?
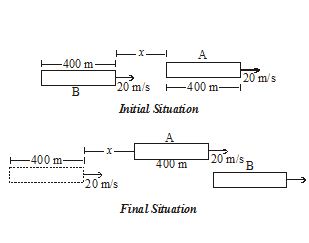
Solution
Let x = initial distance b/w A & B
w.r.t. A, B travels a distance = \displaystyle x+800\,
\displaystyle \therefore \,\,\,\,\,{{S}_{{B,A}}}\,\,=\,\,x+800
\displaystyle {{u}_{{B,\,A}}}\,\,=\,0
\displaystyle {{a}_{{B,A}}}\,\,=\,\,1\,\,m/{{s}^{2}}
\displaystyle t\,=\,50\,\,\sec
Hence applying \displaystyle {{S}_{{B,\,\,A}}}\,=\,{{u}_{{BA}}}t\,+\,\frac{1}{2}{{a}_{{B,A}}}{{t}^{2}}
\displaystyle x\,+\,800\,=\,0+\frac{1}{2}\times \,1\,\,\times \,\,{{50}^{2}}
\displaystyle \therefore \,\,\,x\,=\,450\,\,m
Illustration
Two towns A and B are connected by a regular bus service with a bus leaving in either direction energy t min. A man cycling with a speed of 20 km/h in the direction A to B notices that a bus goes past him every 18 min in the direction of his motion and every 6 min in the opposite direction. What is the period T of the bus service and with what speed (assumed constant) do the busses ply on the road l?
Solution
Let v km/h be the constant speed with which the busses plying between the towns A and B. The relative velocity of the bus (for the motion A to B) with respect to the cyclist (i.e., in the direction in which the cyclist is going = ( \displaystyle v-20) \displaystyle km{{h}^{{-1}}}. The relative velocity of the bus from B to A with respect to the cyclist ( \displaystyle v+20) km/h.
The distance travelled by the bus in time T (minutes) = vT
As per question,
\displaystyle \frac{{vT}}{{v-20}}\,=\,18\,\,or\,\,vT\,\,=\,\,18v\,\,-\,18\,\times \,20 …(i)
and \displaystyle \frac{{vT}}{{v-20}}\,=\,6\,\,or\,\,vT\,\,=\,\,6v\,\,+\,20\,\times \,6 ….(ii)
By Eqs. (i) and (ii), we get
\displaystyle 18v\,-\,18\,\times \,20\,\,=\,6v\,\,+\,20\,\,\times \,\,6
Or \displaystyle 12v\,=20\,\times \,6\,\,+\,18\,\,\times \,\,20\,=\,480
Or \displaystyle v\,=\,40\,\,km/h
Putting this value of v in Eq. (i), we get
\displaystyle 40T\,\,=\,\,18\,\,\times \,\,40-18\times \,\,20
= \displaystyle 18\,\,\times \,\,20
Or \displaystyle T\,=\,18\,\times \,\,20/40\,\,=\,9\,\,\min
Illustration
From a lift moving upward with a uniform acceleration ‘a’, a man throws a ball vertically upwards with a velocity v relative to the lift. The ball comes back to the man after a time t. Show that a + g = 2 v/t.
Solution:
Let us consider all the motion from lift frame. Then, the acceleration, displacement and velocity everything will be considered from the lift frame itself.
As the ball comes again to the man, therefore displacement from the lift frame is zero.
Again, the velocity with respect to the lift frame is v.
Similarly, the acceleration with respect the lift frame is
g – (–a) = a + g (downwards)
Now, s = ut + \displaystyle \frac{1}{2}at2
0 = vt – \displaystyle \frac{1}{2} (a+g)t2
or a + g = 2 \displaystyle \frac{v}{t}.
RELATIVE VELOCITY IN TWO DIMENSIONAL MOTION
All the motion study till now is defined with respect to a reference point of a coordinate system i.e. origin O, which is assumed to be at rest. The motion of any object, say A, w.r.t. any other moving object, say B, is termed as relative motion of object A with respect to object B.
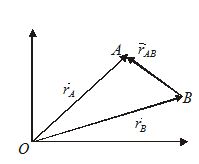
In figure the position vector of A with respect to B can be given as {{\vec{r}}_{{AB}}}={{\vec{r}}_{A}}-{{\vec{r}}_{B}}
Differentiating this equation, we get
\frac{{d{{{\vec{r}}}_{{AB}}}}}{{dt}}=\frac{{d{{{\vec{r}}}_{A}}}}{{dt}}-\frac{{d{{{\vec{r}}}_{B}}}}{{dt}}
or {{\vec{v}}_{{AB}}}={{\vec{v}}_{A}}-{{\vec{v}}_{B}}
Here {{\vec{v}}_{A}} and {{\vec{v}}_{B}} are the velocities of A and B, respectively, with respect to O and {{\vec{v}}_{{AB}}} is the velocity of A w.r.t. B
Differentiating again, we get
\frac{{d{{{\vec{v}}}_{{AB}}}}}{{dt}}=\frac{{d{{{\vec{v}}}_{A}}}}{{dt}}-\frac{{{{{\vec{v}}}_{B}}}}{{dt}}
or \displaystyle \overset{\to }{\mathop{{{{a}_{{AB}}}}}}\,=\overset{\to }{\mathop{{{{a}_{A}}}}}\,-\overset{\to }{\mathop{{{{a}_{B}}}}}\,
(i)
If an object move with uniform relative acceleration, then equations of motions are modified as
\overset{\to }{\mathop{{{{v}_{{rel}}}}}}\,=\overset{\to }{\mathop{{{{u}_{{rel}}}}}}\,+\overset{\to }{\mathop{{{{a}_{{rel}}}}}}\,t
\overset{\to }{\mathop{{{{s}_{{rel}}}}}}\,=\overset{\to }{\mathop{{{{u}_{{rel}}}}}}\,+\frac{1}{2}\overset{\to }{\mathop{{{{a}_{{rel}}}}}}\,{{t}^{2}}
{{v}_{{rel}}}^{2}-{{u}_{{rel}}}^{2}=2\overset{\to }{\mathop{{{{a}_{{rel}}}}}}\,.\overset{\to }{\mathop{{{{s}_{{rel}}}}}}\,
Rain–Man problem
If the rain is falling down with a velocity {{\vec{v}}_{{rain}}} and the man is moving on ground with velocity {{\vec{v}}_{{man}}}, the relative velocity of rain w.r.t. man {{\vec{v}}_{{rel}}} appear different than that it appears to a stationary observer.
Case-I: When rain is falling vertically down
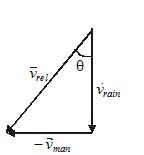
As explain above, the relative velocity of rain with respect to man is given as
{{\vec{v}}_{{rel}}}={{\vec{v}}_{{rain}}}-{{\vec{v}}_{{man}}} ={{\vec{v}}_{{rain}}}+(-{{\vec{v}}_{{man}}})
This equation is represented in figure and it suggest that
{{\vec{v}}_{{rel}}}=\sqrt{{{{v}_{{rain}}}^{2}+{{v}_{{man}}}^{2}}}
The rain appear to man falling down from the front making an angle with vertical given as
\theta ={{\tan }^{{-1}}}\left( {\frac{{{{v}_{{man}}}}}{{{{v}_{{rain}}}}}} \right)
Illustration
During a rainstorm the raindrops are observed to striking ground at an angle of 37° with the vertical. The wind speed is 4.5 m/s. Assuming that the horizontal velocity component of the raindrops is same as the speed of air, what is the vertical component of raindrops? What is their speed?
Let v= velocity of raindrops relative to earth and {{v}_{w}} wind velocity.
Then {{v}_{x}}={{v}_{w}}. = 4.5 m/s
From figure
{{v}_{y}}=\frac{{{{v}_{w}}}}{{\tan 37{}^\circ }}=6.0\,\,\mathbf{m/s} and v=\sqrt{{{{v}_{x}}^{2}+{{v}_{y}}^{2}}}=7.5\,\,\mathbf{m/s}
Illustration
A man standing on a road has to hold his umbrella at 30° with the vertical to keep the rain away. He throws the umbrella and starts running at 10 km/h. He finds that raindrops are hitting his head vertically. Find the speed of raindrops with respect to (a) the road, (b) the moving man runs is shown in the figure (b)

Here \displaystyle {{\overrightarrow{{v\,}}}_{{r,g}}}=velocity of the rain with respect to the ground
\displaystyle {{\overrightarrow{{v\,}}}_{{m,g}}}=velocity of the man with respect to the ground and
\displaystyle {{\overrightarrow{{v\,}}}_{{r,m}}}= velocity of the rain with respect to the man.
We have, \displaystyle {{\overrightarrow{{v\,}}}_{{r,g}}}={{\overrightarrow{{v\,}}}_{{r,m}}}+{{\overrightarrow{{v\,}}}_{{m,g}}} … (1)
Taking horizontal components, equation (1) gives
\displaystyle {{v}_{{r,g}}}\sin 30{}^\circ ={{v}_{{m,g}}}=10km/h
or \displaystyle {{v}_{{r,g}}}=\frac{{10\,\text{km/h}}}{{\sin 30{}^\circ }}=20\text{km/h}
Taking vertical components, equation (1) gives
\displaystyle {{v}_{{r,g}}}\cos 30{}^\circ ={{v}_{{r,\,\,m}}}
or \displaystyle {{v}_{{r,m}}}=(20\text{km/h})\frac{{\sqrt{3}}}{2}
= \displaystyle 10\sqrt{3} km/h
Objective Assignment
1.
Two cars get closer by 8 m every second while traveling in the opposite directions. They get closer by 0.8 m while traveling in the same directions. What are the speeds of the two cars?
(a) 4 m/s and 4.4 m/s (b) 4.4 m/s and 3.6 m/s
(c) 4 m/s and 3.6 m/s (d) 4 m/s and 3 m/s
Ans (b)
2.
A river is flowing from east to west at a speed of 5 m/min. A man on south bank of river, capable of swimming 10 m/min in still water, wants to swim across the river in shortest time, then he should swim
(a) due north
(b) due north-east
(c) due north-east with double the speed of river
(d) due north-west
Ans (a)
3.
The speed of a boat is 5 km/h in still water. It crosses is river of width 1.0 km along the shortest path in 15 minutes, the velocity of the river water (in km/h)
(a) 5 (b) 1 (c) 3 (d) 4
Ans(c)
4.
In previous question, the angle by which the boat should be rowed with the stream is
(a) 37° (b) 53° (c) 90° (d) 127°
Ans (d)
Subjective Assignment
Lorem ipsum dolor sit amet, consectetur adipiscing elit. Ut elit tellus, luctus nec ullamcorper mattis, pulvinar dapibus leo.