Video Lecture
Theory For Notes Making
Lorem ipsum dolor sit amet, consectetur adipiscing elit. Ut elit tellus, luctus nec ullamcorper mattis, pulvinar dapibus leo.
Objective Assignment
Q.1
The mutual inductance between a pair of coils each of N turns is M. If a current i in the first coil is brought to zero in time t, then, average emf induced in the second coil is
(a) \frac{{Mi}}{t}
(b) \frac{{NMi}}{t}
(c) \frac{{Mi}}{{Nt}}
(d) \frac{{Mt}}{{iN}}
Ans. (a)
Q.2
Two coils X and Y are placed in a circuit such that a current changes by 2 A in coil X and the magnetic flux change of 0.4 weber occur in Y. The value of mutual inductance of the coils is
(a) 0.2 henry
(b) 5 henry
(c) 0.8 henry
(d) 2 henry
Ans. (a)
Q.3
If two coils of self inductance 4H and 16H are wound on the same iron core. The coefficient of mutual inductance for them will be
(a) 8H (b) 10H (c) 20H (d) 164H
Ans. (a)
Q.4
A small square loop of wire of side l is placed inside a large square loop of wire of side
L( L >> l). The loops are co-planar and their centres coincide. The mutual inductance of the system is proportional to
(a) l/L (b) l2/L (c) L/l (d) L2/l
Ans. (b)
5.
An ideal transformer has 500 and 5000 turns in primary and secondary winding respectively. If the primary voltage is connected to a 6 V battery then the secondary voltage is
(a) 0
(b) 60 V
(c) 0.6 V
(d) 6.0 V
Ans (a)
6.
A step-down transformer transforms a supply line voltage of 2200 volt into 220 volt. The primary coil has 5000 turns. The number of turns in the secondary are
(a) 5000
(b) 550
(c) 500
(d) 450
Ans (b)
7.
A transformer
(a) transforms energy
(b) transforms frequency
(c) transforms voltage
(d) generates e.m.f.
Ans (c)
8.
In a transformer, the number of turns of primary coil and secondary coil are 5 and 4 respectively. If 240 V is applied on the primary coil, then the ratio of current in primary and secondary coil is
(a) 4 : 5
(b) 5 : 4
(c) 5 : 9
(d) 9 : 5
Ans (A)
9.
The number of turns in the primary and secondary turns of a transformer are 1000 and 3000 respectively. If 80 V AC is supplied to the primary coil of the transformer, then the potential difference per turn of the secondary coil would be
(a) 240 V
(b) 2400 V
(c) 0.24 V
(d) 0.08 V
Ans (d)
10.
A transformer has 200 windings in the primary and 400 windings in the secondary. The primary is connected to an ac supply of 110 V a current of 10 A flows in it. The voltage across the secondary and the current in it, respectively, are
(a) 55V, 20 A
(b) 440 V, 5A
(c) 220 V, 10A
(d) 220V, 5A
Ans (d)
Subjective Assignment
Q.1
How does the mutual inductance of a pair of coils change when
(i) distance between the coils is increased and
(ii) number of turns in the coils is increased ?
Q.2
Compute the mutual inductance for a given pair of coils if increase in current from 2A to 6A in of coil if increase in current from 2A to 6A in 0.1 s in one coil causes an inducted emf of 1V in the other coil.
Q.3
Two concentric circular coils, one of small radius \displaystyle {{r}_{1}} and the other of large radius \displaystyle {{r}_{2}}, such that \displaystyle {{r}_{1}}\,<\,<\,{{r}_{2}} are placed coaxially with centres coinciding. Obtain the mutual inductance of the arrangement.
Q.4
A pair of adjacent coils has a mutual inductance of 1.5 H. If the current in one coil changes from 0 to 20 A in 0.5 s, what is the change of flux linkage with the other coil?
Ans. 30 Wb
Q.5
How does the mutual inductance of a pair of coils change when
(i) distance between the coils is increased
(ii) an iron sheet is placed between the two coils?
Q.6
Define the coefficient of mutual induction. A long solenoid, of length l and radius, is enclosed coaxially within another long solenoid of length l and radius \displaystyle {{r}_{2}}\,\left( {{{r}_{2}}\,>\,{{r}_{1}}\,\,and\,\,\,l\,\,>\,>\,{{r}_{2}}} \right). Define the expression for the mutual inductance of this pair of solenoids.
Q.7
A very small circular loop, of radius \displaystyle {{r}_{1}},lies coplanarly within another circular loop of radius \displaystyle {{r}_{2}}, with its centre coincident with that of the larger loop. Derive an expression for the coefficient of mutual induction for this pair of loops.
Q.8
What do you understand by mutual Induction and Mutual Inductance. Derive an expression for the mutual inductance of two long coaxial solenoids having different radii and different number of turns.
Q.9
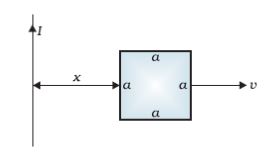
(a) Obtain an expression for the mutual inductance between a long straight wire and a square loop of side a as shown in Fig.
(b) Now assume that the straight wire carries a current of 50 A and the loop is moved to the right with a constant velocity, v = 10 m/s. Calculate the induced emf in the loop at the instant when x = 0.2 m. Take a = 0.1 m and assume that the loop has a large resistance.
Ans. \displaystyle \varepsilon \,=\,1.7\,\times \,{{10}^{{-5}}}\,v