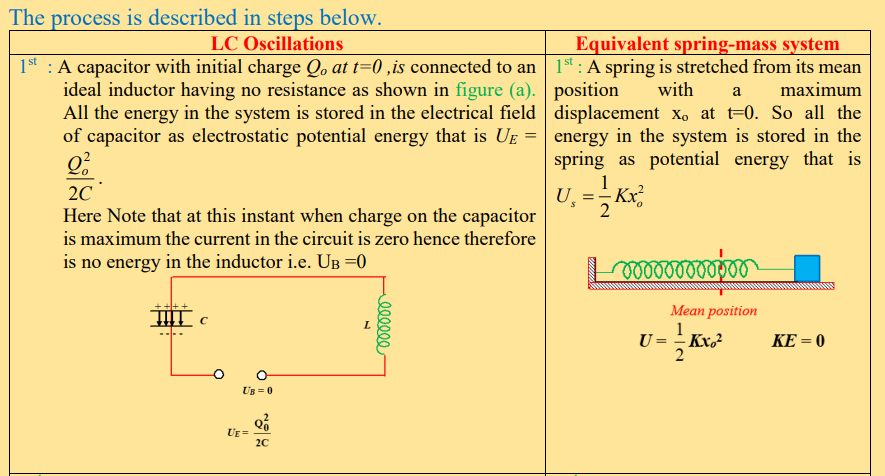
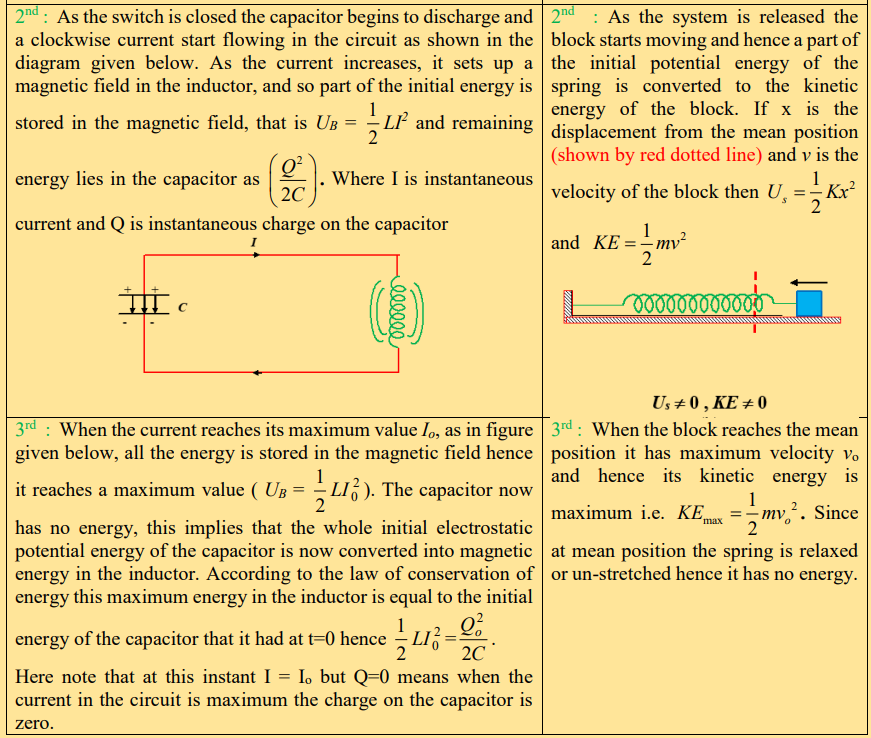
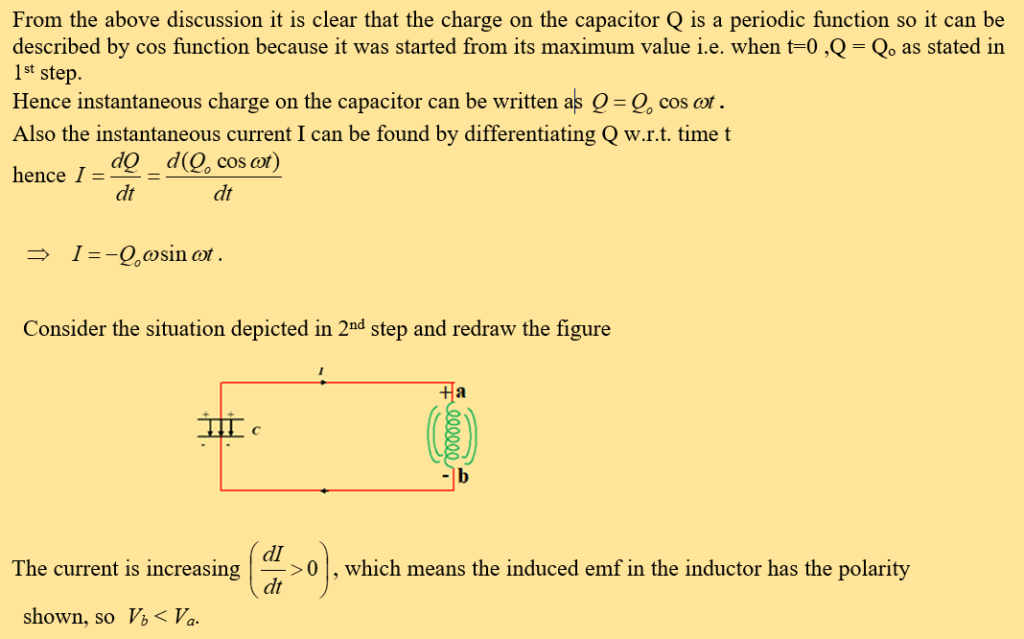
Video Lecture
Theory For Making Notes
Lorem ipsum dolor sit amet, consectetur adipiscing elit. Ut elit tellus, luctus nec ullamcorper mattis, pulvinar dapibus leo.
Practice Questions (Basic Level)
Lorem ipsum dolor sit amet, consectetur adipiscing elit. Ut elit tellus, luctus nec ullamcorper mattis, pulvinar dapibus leo.
Practice Questions (JEE Main Level)
Lorem ipsum dolor sit amet, consectetur adipiscing elit. Ut elit tellus, luctus nec ullamcorper mattis, pulvinar dapibus leo.
Practice Questions (JEE Advance Level)
Q.1
The natural frequency of the circuit shown in the figure is :
(a) \displaystyle \frac{1}{{2\pi \sqrt{{LC}}}}
(b) \displaystyle \frac{1}{{2\pi \sqrt{{2LC}}}}
(c) \displaystyle \frac{2}{{2\pi \sqrt{{LC}}}}
(d) None of these
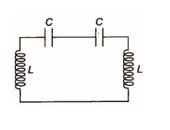
Ans. (a)
Q.2
Initially the 900mF capacitor is charged to 100V and the 100mF capacitor is uncharged in the figure shown. Then the switch S2 is closed for a time t1, after which it is opened and at the same instant switch S1 is closed for a time t2 and then opened. It is now found that the 100mF capacitor is charged to 300 V. Then the minimum possible values of the time intervals t1 and
t2 should be
(a) t1 = 0.5s, t2 = 1.05s
(b) t1 = 1.15s, t2 = 2.05s
(c) t1 = 0.15s, t2 = 0.05s
(d) t1 = 3.15s, t2 = 1.05s
Ans. (c)
Q.3
The capacitor shown in figure is initially charged to a voltage V and the circuit is then closed. The charge on C is found to oscillate with frequency w. The frequency will be doubled if
(a) The voltage V is doubled
(b) both L and C are halved
(c) both L and C are doubled
(d) both L and C are increased by factor \sqrt{2}
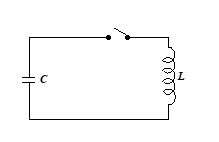
Ans (d)
4.
A capacitor is charged to a potential of V0. It is connected with an inductor through a switch S. The switch is closed at time t = 0. Which of the following statement is correct.
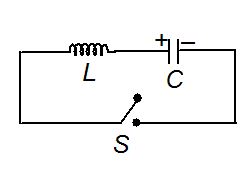
(a) maximum current in the circuit is V0 \sqrt{{LC}}
(b) potential across capacitor becomes zero for the first time at t = \displaystyle \pi \sqrt{{LC}}
(c) energy stored in the inductor at time \frac{\pi }{2}\sqrt{{LC}} is \frac{1}{4}CV_{0}^{2}
(d) maximum energy stored in the inductor is \frac{1}{2}CV_{0}^{2}.
Ans (d)
5.
A L-C circuit (inductance 0.01 H, capacity 1 \displaystyle \mu F) is connected to a variable frequency ac source. If frequency varies from 1 kHz to 2 kHz, then frequency at which the current in LC circuit is zero is at
(a) 1.2 kHz
(b) 1.4 kHz
(c) 1.6 kHz
(d) 1.8 kHz
Ans (c)
6.
Two capacitors of capacitance {{C}_{1}}and {{C}_{2}} are charged to a potential difference of {{V}_{1}} and {{V}_{2}} respectively and are connected to an inductor of inductance L as shown in the figure. Initially key k is open. Now key k is closed and current in the circuit starts increasing. When current in the circuit is maximum
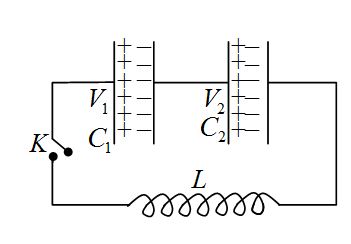
(a) charge on both the capacitors is same
(b) inducedemf in the inductor is zero
(c) potential difference across both the capacitors is half of the induced emf
(d) electrostatic potential energy stored in both the capacitors is same
Ans (d)
7.
At a moment (t = 0), when the charge on capacitor C1 is zero, the switch is closed. If I0 be the current through inductor at t = 0 ,for t> 0
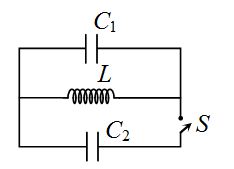
(a) maximum current through inductor equals I0/2.
(b) maximum current through inductor equals \frac{{{{C}_{1}}{{I}_{0}}}}{{{{C}_{1}}+{{C}_{2}}}}.
(c) maximum charge on {{C}_{1}}=\frac{{{{C}_{1}}{{I}_{0}}\sqrt{{L{{C}_{2}}}}}}{{{{C}_{1}}+{{C}_{2}}}}.
(d) maximum charge on {{C}_{1}}={{C}_{1}}{{I}_{0}}\sqrt{{\frac{L}{{{{C}_{1}}+{{C}_{2}}}}}}.
Ans (d)
Comprehension ( Question No.- 8 to 11 )
An inductor of inductance 2.0 mH is connected across a charged capacitor of capacitance 5 \left| {\frac{{di}}{{dt}}} \right|F and the resulting L-C circuit is set oscillating at its natural frequency. Let Q denote the instantaneous charge on the capacitor and i the current in the circuit. It is found that the maximum value of Q is 200 \displaystyle \mu C. Now answer the following questions based on the information given above:
8.
When Q = 100 \displaystyle \mu C, the value of \left| {\frac{{di}}{{dt}}} \right| is
(a) zero
(b) 104 A/s
(c) 10–4 A/s
(d) 102 A/s
Ans (b)
9.
When Q = 200 \displaystyle \mu C, the value of i is
(a) zero
(b) 2 Amp
(c) \sqrt{2}Amp
(d) \sqrt{3} Amp
Ans (a)
10.
The maximum value of i is
(a) \sqrt{3}Amp
(b) \sqrt{7}Amp
(c) 2 Amp
(d) 1 Amp
Ans (a)
11.
When is half of its maximum value, the value of |Q| is
(a) \displaystyle \sqrt{3}Coulomb
(b) \sqrt{3}\times {{10}^{{-8}}}Coulomb
(c) \sqrt{3}\times {{10}^{{-4}}}Coulomb
(d) 100 Coulomb
Ans (a)
Comprehension ( Question No.- 12 to 14 )
Two capacitors P and Q of capacitance 2C and C are connected in series with an inductor of inductance L. Initially capacitors are charge such that VB –VA = 4V0 and VC –VD = V0 (see figure). Maximum current in the circuit is Imax at t = t0
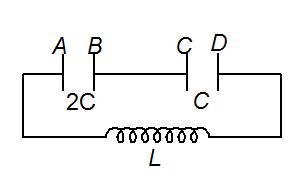
12.
Maximum current that will flow in the circuit is
(a) {{V}_{0}}\sqrt{{\frac{{6C}}{L}}}
(b) {{V}_{0}}\sqrt{{\frac{C}{L}}}
(c) \frac{{{{V}_{0}}}}{2}\sqrt{{\frac{L}{{6C}}}}
(d) \frac{{{{V}_{0}}}}{2}\sqrt{{\frac{L}{{6C}}}}
Ans. (a)
13.
Find the potential difference across capacitor P at t = t0
(a) \frac{{{{V}_{0}}}}{2}
(b) 3 V0
(c) 2V0
(d) \frac{5}{2}{{V}_{0}}
Ans. (b)
14.
Maximum energy store in inductor is
(a) \frac{1}{2}CV_{0}^{2}
(b) \frac{1}{6}C{{V}_{0}}
(c) 3CV_{0}^{2}
(d) \frac{{17}}{2}CV_{0}^{2}
Ans. (c)
Comprehension ( Question No.- 15 to 17 )
In the figure shown C1 = 1F, C2 = 2F and L = 5H. Initially C1 is charged to 50 volt and C2 to 10 Volt. Switch S is closed at time t = 0. Suppose at some instant t0 charge on C1 is 20C with the same polarities as t = 0, shown in the figure.
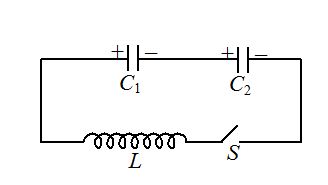
15.
Energy stored in capacitor C2 at this instant t0 will be
(a) 10 J
(b) 15 J
(c) 25 J
(d) 40 J
Ans (c)
16.
Current in the circuit at t = t0 will be
(a) 10 \sqrt{2}A
(b) 15 \sqrt{2}A
(c) 10 A
(d) 20 A
Ans (b)
17.
Maximum current in the circuit will be
(a) 4\sqrt{{30}}A
(b) 16\sqrt{2}A
(c) 20\sqrt{3}A
(d) 12\sqrt{6}A
Ans (c)