Video Lectures
Theory For Making Notes
Circular Motion:
If a particle is moving in a plane in such a way that, its distance from a fixed point is a constant then path followed by it is a circle. Such motion is known as circular motion.
Angular Position :
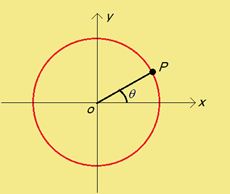
Angle between the reference axis and the position vector of a particle is the Angular position
Angular Velocity :
As the particle moves on the circle its angular position θ changes. Suppose the particle goes to a near by point in time ∆t so that θ increases to θ+∆θ.

The rate of change of angular position is called angular velocity. Thus the angular velocity is given by :
Instantaneous angular velocity \omega =\underset{{\Delta t\to 0}}{\mathop{{\lim }}}\,\frac{{\Delta \theta }}{{\Delta t}}=\frac{{d\theta }}{{dt}}
If a body completes a full circle in time period ‘T’
then \displaystyle \omega \,=\,\frac{{2\pi }}{T}\,=\,2\pi \upsilon , = frequency of revolution in rps.
Angular Velocity Vector :
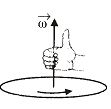
Angular speed \displaystyle \omega is the magnitude of vector called the angular velocity \displaystyle \vec{\omega } of the particle. Direction of \displaystyle \vec{\omega } can be determined from circular motion right hand rule. Curl your fingers of right hand in the sense of rotation of particle, then the extended thumb points in the direction of \displaystyle \vec{\omega }.
Uniform Circular Motion
If a particle moves along the circumference of a circle with constant speed, the motion is termed as uniform circular motion.
Here, the magnitude of the velocity vector remains constant but the direction changes continuously and therefore it is an accelerated motion. To analyze the circular motion, we choose a reference frame in the form of a xy–plane with its origin at the centre of the circle on which the particle moves.
Let R be the radius of the circle. The instantaneous position of the particle is expressed by an angle q between a radial line OP and a reference line such as OA.
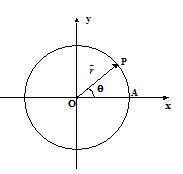
Suppose the particle starts at t = 0 from point A and reaches point P in time t. The position vector of the particle rotates by an angle q. If the distance moved along the circumference of the circle is s then
s = R θ
Differentiating with respect to time, we get
\frac{{ds}}{{dt}}=R\frac{{d\theta }}{{dt}}
The quantity \frac{{ds}}{{dt}} = v the speed of the particle at any instant.
The quantity \frac{{d\theta }}{{dt}} is called the angular speed of the particle and it is denoted by w (omega).
Thus v = Rw
Note : In vector form \displaystyle \vec{v}\,\,=\,\,\vec{\omega }\,\,\times \,\,\vec{r}
We can now write the position vector of the particle \displaystyle \vec{r}. From the figure,
\displaystyle \vec{r} = R cos q \hat{i} + R sin q \hat{j}
Differentiating with respect to time, we get
\vec{v}=\frac{{d\vec{r}}}{{dt}}=-R\sin \theta .\frac{{d\theta }}{{dt}}\hat{i}+R\cos \theta \frac{{d\theta }}{{dt}}\hat{j}
= –Rw sin q \hat{i} + Rw cos q \hat{j}
The magnitude of \left| {\vec{v}} \right|=\sqrt{{{{{\left( {-R\omega \sin \theta } \right)}}^{2}}+{{{\left( {R\omega \cos \theta } \right)}}^{2}}}} = Rw as expected.
If we take the dot product of \displaystyle \vec{v} and \displaystyle \vec{r}, it is observed to be zero. Thus the velocity vector is perpendicular to the position (radius) vector i.e. it is along the tangent to the circle. This is once again an expected result.
Next, we differentiate with respect to time to get , thus
\vec{a}=\frac{{d\vec{v}}}{{dt}}=-R\omega \cos \theta \frac{{d\theta }}{{dt}}\hat{i}-R\omega \sin \theta \frac{{d\theta }}{{dt}}\hat{j}
= -w2 \left( {R\cos \theta \,\,\hat{i}+R\sin \theta \,\,\hat{j}} \right) (Q w = \frac{{d\theta }}{{dt}})
\vec{a}=-{{\omega }^{2}}\vec{r}
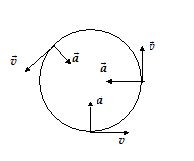
The acceleration vector has a magnitude ω2R and is oppositely directed to \displaystyle \vec{r} i.e. towards the centre of circle. This acceleration is called the centripetal acceleration. We now summarize the situation in the figure.
Note that the magnitude of centripetal acceleration can also be written as \frac{{{{v}^{2}}}}{R}
Non-Uniform Circular Motion
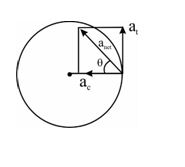
We now consider the more general case of circular motion in which the speed of the particle is not constant. In uniform circular motion, the acceleration resulted from the change in direction only of the velocity vector. Here, both the magnitude and direction of the velocity vector change and, therefore, not only the centripetal acceleration, we have another acceleration also which results from the change in magnitude of the velocity vector. This acceleration, is called the tangential acceleration, it has a magnitude equal to \frac{{dv}}{{dt}} and is directed either in the direction of \vec{v} (speed increasing) or opposite to the direction of \vec{v} (speed decreasing). Note that in this case, the magnitude of centripetal acceleration is also not a constant as it depends on v. Thus, in case of non-uniform circular motion, the total acceleration vector has two components
(i) Centripetal acceleration ac= \frac{{{{v}^{2}}}}{R} = w2R
(ii) Tangential acceleration at= \frac{{dv}}{{dt}}
Hence anet = \sqrt{{a_{c}^{2}+a_{t}^{2}}}
also tanθ =\frac{{{{a}_{t}}}}{{{{a}_{c}}}} where θ is the angle made by the net acceleration with the radius
In non uniform circular motion when the angular velocity of a body changes with time, another acceleration called angular acceleration is also considered. It can be defined as the time rate of change of angular velocity and is denoted by α.
Concept : 1.
The angular acceleration vector \displaystyle \vec{\alpha } (t) points in the direction of the change in the angular velocity vector \displaystyle \vec{\omega }\left( t \right). If speed of particle increases or decreases, the angular velocity vector also increases or decreases. Direction of angular velocity vector is always normal to plane rotation.
Therefore the angular acceleration vector \displaystyle \vec{\alpha }\left( t \right) is directed either parallel to \displaystyle \vec{\omega }\left( t \right) when the particle is speeding up along the circle, or antiparallel to \displaystyle \vec{\omega }\left( t \right) when the particle is slowing.
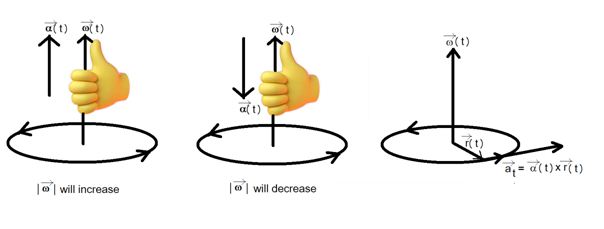
Hence \alpha =\frac{{d\omega }}{{dt}}, And at = r.α
If α is constant then
\begin{array}{l}\omega =\omega o+\alpha t,\\\theta =\omega ot+\frac{1}{2}\alpha {{t}^{2}},\\{{\omega }^{2}}=\omega {{o}^{2}}+2\alpha \theta .\end{array} \displaystyle \}These equations are called the equations of kinematics in circular motion.
\displaystyle \omega = final angular velocity.
\displaystyle {{\omega }_{0}}= Initial angular velocity
\displaystyle \theta = angular displacement
Tangential and Normal acceleration
When a particle moves along in a curvilinear path with variable speed (like non-uniform circular motion) it can be shown to have two acceleration components.

One of these components is called the tangential component at resulting from a change in magnitude of velocity vector and has a magnitude at= \frac{{dv}}{{dt}}. The second component is termed as the normal component anand its magnitude is equal to \displaystyle \frac{{{{v}^{2}}}}{r} where r is the radius of curvature of the path at that point.
Practice Questions (Basic Level)
1.
The speed of a particle revolving in a circle slows down at a rate of 2m/sec2.At some instant the magnitude of the total acceleration is 4m/sec2 and the speed is 12m/sec.The radius of the circle is
(a)21m
(b)42m
(c)10.5m
(d)48m
Ans : b
2.
A cyclist is moving on a circular path with constant speed then the direction of the net angular velocity of the wheels of the bicycle must be
(a)towards the center of the circular path
(b)towards the center of the wheel
(c)neither of a or b
(d)depends on the speed of the bicycle.
Ans : c
3.
A particle is moving along a circular path with uniform speed. Through what angle does its angular velocity change when it completes half of the circular path?
(a) 360°
(b) 180°
(c) 45°
(d) 0°
Ans (d)
4.
To enable a particle describe a circular path of constant radius with constant speed, what should be the angle between its velocity and acceleration?
(a) 180°
(b) 90°
(c) 45°
(d) 0°
Ans (b)
5.
The angular speed of the second’s hand of a clock is
(a) \frac{\mathbf{1}}{{\mathbf{60}}}\mathbf{rad/s}
(b) \frac{\mathbf{\pi }}{{\mathbf{60}}}\mathbf{rad/s}
(c) \frac{{\mathbf{\pi }}}{{\mathbf{30}}}\mathbf{rad/s}
(d) \frac{{\mathbf{360}}}{{\mathbf{60}}}\mathbf{rad/s}
Ans .(c)
6.
A particle is moving in a plane along a circular path. The angular velocity, linear velocity, angular acceleration and centripetal acceleration of the particle at any instant respectively are w, v, a and ac . Which of the following relations is not correct?
(a) \vec{\omega }\bot \vec{v}
(b) \vec{\omega }\bot \vec{\alpha }
(c) \vec{\omega }\bot {{\vec{a}}_{c}}
(d) \vec{v}\bot {{\vec{a}}_{c}}
Ans (b)
7.
The length of second’s hand in a watch is 1 cm. The change in velocity of its tip in a period of 15s is
(a) Zero
(b) \left( {\frac{\pi }{{15\sqrt{2}}}} \right)\mathbf{cm}{{\mathbf{s}}^{{-\mathbf{1}}}}
(c) \left( {\frac{\pi }{{15}}} \right)\mathbf{cm}{{\mathbf{s}}^{{-\mathbf{1}}}}
(d) \left( {\frac{{\pi \sqrt{2}}}{{15}}} \right)\mathbf{cm}{{\mathbf{s}}^{{-\mathbf{1}}}}
Ans (b)
8.
A wheel is subjected to uniform angular acceleration about its axis. Initially its angular velocity is zero. In the first two second it rotates through an angle q1. In next two second it rotates through {{\theta }_{2}}. What is the ratio \frac{{{{\theta }_{2}}}}{{{{\theta }_{1}}}}?
(a) 4
(b) 3
(c) 2
(d) 1
Ans .(b)
9.
A particle is describing a circular path of radius 10m in time interval of every 2 s. The average angular speed of the particle during 4 s is
(a) \,\,\pi \,\,\mathbf{rad}\,{{\mathbf{s}}^{{-\mathbf{1}}}}
(b) 4\,\,\pi \,\,\mathbf{rad}\,{{\mathbf{s}}^{{-\mathbf{1}}}}
(c) 2\,\,\pi \,\,\mathbf{rad}\,{{\mathbf{s}}^{{-\mathbf{1}}}}
(d) None of these
Ans (a)
10.
A body covers a semicircular path of length 5 m . The ratio of magnitude of linear velocity and angular velocity is
(a) 10 : p2
(b) 5 : p
(c) 5 : p2
(d) 10 : p
Ans (a)
11.
A particle is moving on a circle of constant radius with a constat speed of 5m/s. At any instant it is moving eastward and in 10 second, the velocity just changes it direction to northward. The radius of the path and the average acceleration in this time interval must be
(a) 10/p m , Zero
(b) 100/p m , \frac{1}{{\sqrt{2}}}\,\,\mathbf{m/}{{\mathbf{s}}^{2}} towards north–west
(c) 100/p m , \frac{1}{{\sqrt{2}}}\,\,\mathbf{m/}{{\mathbf{s}}^{\mathbf{2}}} towards north – east
(d) p/20 m , \frac{1}{2}\,\,\mathbf{m/}{{\mathbf{s}}^{\mathbf{2}}} towards north–west
Ans (b)
12.
Starting from rest, a particle rotates in a circle of radius R=\sqrt{2}\,\mathbf{m} with an angular acceleration \alpha =\frac{\pi }{4}\mathbf{rad/}{{\mathbf{s}}^{\mathbf{2}}}. The magnitude of average velocity of the particle over the time it rotates quarter circle is
(a) 1.5 m/s
(b) 2 m/s
(c) 1 m/s
(d) 1.25 m/s
Ans (c)
13.
In the figure particle is travelling in circular paths of radius 20m, with constant tangential acceleration. The velocity vectors are indicated. Find the ratio of the magnitudes of the net acceleration of particle at the final and initial point
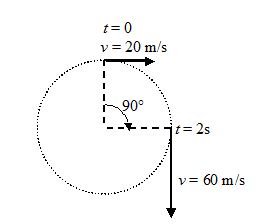
(a) \displaystyle \sqrt{{34}} m/s2
(b) \displaystyle \sqrt{{41}} m/s2
(c) \displaystyle \sqrt{{74}} m/s2
(d) \displaystyle \sqrt{{67}} m/s2
Ans (b)
14.
An object moves without friction along a concave surface.

(a)the tangential component is increasing
(b)the normal component is increasing
(c)the net acceleration is towards the center of the surface
(d)none
Ans : b
15.
A particle start from rest on a circle of radius R =20 cm with a constant tangential acceleration 5 cm/sec2 . The time in which the normal acceleration of the particle become equal to the tangential acceleration is
(a) 4 second
(b) 1 second
(c) 3 second
(d) 2 second
Ans : d
16.
A stone is dropped from the top of the building In a high wind. The wind exerts a steady horizontal force on the stone as it falls. The path of the stone is
(a) straight line
(b) parabola
(c) a circle
(d) another complicated path
Ans : a
17.
A simple pendulum is oscillating without damping . When the displacement of the Bob is less than maximum its the acceleration Vector a is correctly shown in
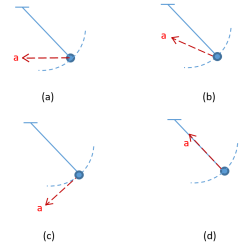
Ans : b
Practice Questions (JEE Main Level)
1.
A particle is moving in a circular path of constant radius r and its centripetal acceleration varies as a=rk2t2, where k is a constant. The power delivered to the particle by the forces acting on it is
(a)2pmk2r2t
(b) mk2r2t
(c) mk4r2t5/3
(d) 2pmk2r2
Ans. b
2.
A particle starts moving along a circular path so that its tangential acceleration \displaystyle {{a}_{t}}=a and the normal acceleration and \displaystyle {{a}_{n}}=b{{t}^{4}} , where a and b are constants. In terms of distance S travelled by the particle the radius of curvature of path is given by
(a) \displaystyle \frac{{{{a}^{3}}}}{{2bs}}
(b) \displaystyle \frac{{{{a}}}}{{2bS}}
(c) \displaystyle \frac{{{{b}^{2}}}}{{2a{{s}^{2}}}}
(d) \displaystyle \frac{{ab}}{{2s}}
Ans : a
3.
A particle of mass m rotates in a circle of radius r with a constant angular velocity w.It is viewed from a frame rotating about Z axis with a constant angular velocity wo . The centripetal force on the particle is
(a) \displaystyle m{{\omega }^{2}}r
(b) \displaystyle m\omega _{o}^{2}r
(c) \displaystyle m{{\left( {\frac{{\omega +{{\omega }_{o}}}}{2}} \right)}^{2}}r
(d)none
Ans : d
4.
A point moves along a circle with velocity v =at (a is constant). The total acceleration of the point at a time when it has traced 1/8th of the circumference is :
(a) \displaystyle \frac{v}{{8a}}
(b) \displaystyle 2a\sqrt{{4+{{\pi }^{2}}}}
(c) a
(d) \displaystyle \frac{a}{2}\sqrt{{4+{{\pi }^{2}}}}
Ans : d
5.
At t = 0 a flywheel is rotating at 50 rpm. A motor gives it a constant acceleration of 0.5 rad/s2 until it reaches 100 rpm. The motor is then disconnected. How many revolutions are completed at t = 20 s?
(a)15rev
(b)20 rev
(c)29 rev
(d) 32 rev
Ans (c)
6.
The angular position of a line of a disc of radius r = 6 cm is given by q = 10 – 5t + 4t2 rad. Find:
6(i)
the average angular velocity between 1 and 3s;
(a)10 rad/s
(b)15 rad/s
(c) 20 rad/s
(d)11 rad/s
Ans (d)
6.(ii)
the linear speed of a point on the rim at 2s;
(a)1.22 m/s
(b)1.55 m/s
(c)2.30 m/s
(d)0.66 m/s
Ans (d)
6(iii)
the radial and tangential accelerations of a point on the rim at 2s.
(a)7.26 m/s2; 5.45 m/s2
(b)7.26 m/s2; 0.48 m/s2
(c)7.26 m/s2; 2.50 m/s2
(d)7.26 m/s2; 1.48 m/s2
Ans (b)
7.
A 30 cm diameter turntable starts from rest and takes 2 s to reach its final rotation rate of
33 \frac{1}{3} rpm. Find
7(i)
the angular acceleration
(a)1.50 rad/s2
(b)1.75 rad/s2
(c)2.30 rad/s2
(d)2.20 rad/s2
Ans (b)
7(ii)
the number of revolutions completed in 5s
(a)1.50 rev
(b) 2.50 rev
(c)2.00 rev
(d)2.22 rev
Ans (d)
7(iii)
the time needed to complete 2 revolutions
(a)4.6 s
(b)2.8 s
(c)1.5 s
(d) 5.5 s
Ans (a)
7(iv)
the radial and tangential accelerations of a point on the rim at 2s.
(a) 3.65 m/s2 , 0.525 m/s2
(b) 0.25 m/s2, 0.525 m/s2
(c) 0.525 m/s2 , 0.25 m/s2
(d) 3.65 m/s20, 0.50 m/s2
Ans (a)
8.
A point mass M slides down a curved friction Less track starting from rest. The curve Obeys the equation \displaystyle y=-\frac{{{{x}^{2}}}}{2} where x ≥ 0. The Tangential acceleration of the mass is
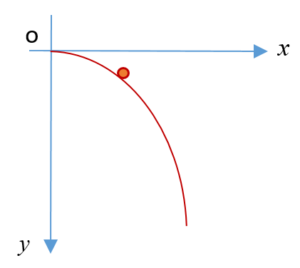
(a) g
(b) \displaystyle \frac{{gx}}{{\sqrt{{4+{{x}^{2}}}}}}
(c) g / 2
(d) \displaystyle \frac{{gx}}{{\sqrt{{1+{{x}^{2}}}}}}
Ans : d
9.
In the previous question what is the coordinates of the mass at which its tangential and normal accelerations are equal
(a) 1, -1
(b) 1/2, -1
(c) 1, -1/2
(d) -1 , -1/2
Ans : c
10.
A car is travelling with linear velocity v on a circular road of radius r. If it is increasing Its speed at the rate of ‘a’ meter / sec2 then the resultant acceleration will be:
(a) \displaystyle \frac{{{{v}^{2}}}}{r}+a
(b) \displaystyle \sqrt{{\frac{{{{v}^{2}}}}{{{{r}^{2}}}}+{{a}^{2}}}}
(c) \displaystyle \sqrt{{\frac{{{{v}^{4}}}}{{{{r}^{2}}}}+{{a}^{2}}}}
(d) \displaystyle \sqrt{{\frac{{{{v}^{2}}}}{{{{r}^{2}}}}-{{a}^{2}}}}
Ans : c
Practice Questions (JEE Advance Level)
1.
A point moves along a circle of radius r. Its velocity varies as \displaystyle v=a\sqrt{S} where a is a constant and s is the distance traveled. The angle a between the total acceleration and the velocity vector is given by
(a) \displaystyle {{\tan }^{{-1}}}\left( {\frac{r}{S}} \right)
(b) \displaystyle {{\tan }^{{-1}}}\left( {\frac{r}{2S}} \right)
(c) \displaystyle {{\tan }^{{-1}}}\left( {\frac{2S}{r}} \right)
(d) \displaystyle {{\tan }^{{-1}}}\left( {\frac{S}{r}} \right)
Ans : c
2.
A particle is moving in a circular orbit with a constant tangential acceleration. After a certain time t has elapsed after the beginning of motion the angle between total acceleration a and direction along the radius R becomes 45o. Then angular acceleration of particle must be
(a) t2
(b)1/t2
(c)t
(d) none
Ans : b
3.
A solid body rotates about a stationary axis so that its angular velocity depends upon the rotation angle f as \displaystyle \omega ={{\omega }_{o}}-a\phi where a and w0 Are positive constants. If initially i.e. at t = 0 , f = 0 then the correct relation between f and time t is given by
(a) \displaystyle \phi ={{\omega }_{o}}(1-{{e}^{{-at}}})
(b) \displaystyle \phi =\frac{{{{\omega }_{o}}}}{a}(1-{{e}^{{-at}}})
(c) \displaystyle \phi ={{\omega }_{o}}{{e}^{{-at}}}
(d) \displaystyle \phi ={{\omega }_{o}}{{e}^{{at}}}
Ans : b
4.
The winding radius of a tape on a cassette was reduced to half in 20 minute of operation . In what time the winding radius be reduced to half again?
(a) 15 minute
(b) 10 minute
(c) 5 minute
(d) 20 minute
Ans : c
5.
The equation of motion of a particle on circular path of radius 19.5m is given by s = 18t +3 t2 – 2t3 where s is the total distance covered from starting point in meters at the end of t seconds . The maximum centripetal acceleration during the motion to keep moving in the given circular path is
(a) 15 m/sec2
(b) 23 m/sec2
(c) 19.5 m/sec2
(d) 25 m/sec2
Ans : c
6.
A stunt rider on a unicycle is riding around the arena of a circus in a circle of radius R.

The radius of wheel of unicycle is r and angular velocity with which the wheel rotates is \displaystyle \omega . Then angular acceleration of wheel must be
(a) \displaystyle \frac{{{{\omega }^{2}}r}}{R}
(b) \displaystyle \frac{{{{\omega }^{2}}R}}{r}
(c) \displaystyle \frac{{\omega {{r}^{2}}}}{{{{R}^{2}}}}
(d) none.
Ans : c
7.
A particle moves in a circle of radius R. Its linear speed is given by v = k t where k is a constant. The instantaneous ratio of its radial and tangential acceleration depends on kn where n is
(a) 2
(b) 3
(c) 1
(d) 4
Ans : c
8.
A body is moving on a curved path of radius r with a speed v=ktn.(k=constant) such that the net acceleration of the body always makes 45o with the radius vector. Choose the wrong statement
(a) the graph between the radius and time is a parabola if n is equal to 1
(b) the centripetal acceleration of the body is changing with time except for n equal to 1
(c) the graph between tangential acceleration and time is a parabola for n equal to 3
(d) The motion is uniform circular motion if n is equal to zero.
Ans : d