Video Lecture
Theory For Making Notes
Electric Lines of Force
The lines of forces are purely geometrical construction which helps in visualising the nature of electric field in a region. It has no physical existence. Lines of forces are drawn in such a way that the tangent to a line of force gives the direction of resultant electric field. The density of field lines in any region is proportional to the magnitude of the electric field in that region. Field lines originate on positive charges and terminate on negative charges.
1.
There cannot be any closed line of force in an electrostatic field \displaystyle \vec{E}. Electric lines of force emerge from positive charges and terminate on negative charges (or extends to infinity).

2.
Crowded lines represent strong field, while distant represent weak field.
3.
The number of lines originating or terminating on a charge is proportional to the magnitude of the charge. In SI units, the number of electric field lines associated with a unit charge (i.e., 1 coulomb) is taken as 1/ε0. Thus, if a body encloses a charge q, total lines of force associated with it (also called flux) will be q/ε0.
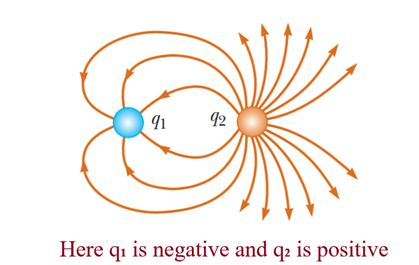
In the above figure the magnitude of q2 is more than that of q1.
4.
Lines of force can never cross each other. It is obvious, because if they cross at a point the intensity at that point will have two directions, which is absurd.
5.
Lines of force have tendency to contract longitudinally (like a stretched elastic string) and repel each other laterally. This concept, like the lines of force, is also imaginary. It helps in understanding how attraction is produced between opposite charges, and how repulsion is produced between similar charges.
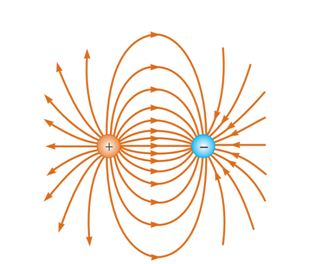
Electric field lines for two positive point-charges of equal magnitude.

Electric field lines of two similar charges placed near to each other
6.
If there is no electric field in a region of space, there will be no lines of force. This is why inside a conductor or at a neutral point (where resultant intensity is zero) there cannot be any line of force.
7.
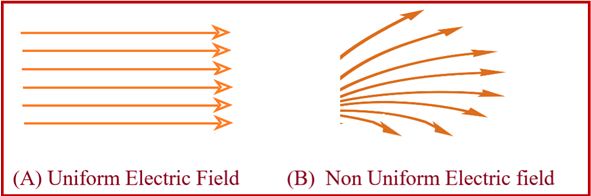
If the lines of force are equidistant straight lines, the field is uniform [Fig. (A)]. If either lines of force are not equidistant or straight lines or both, the field will be non-uniform [Fig. (B)]
Electric Flux
Consider a surface of area A placed in an electric field E.
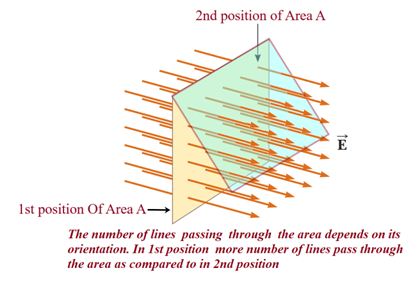
Now the number of lines that penetrate through the surface depends on three factors
(i) Strength of electric field E
(ii) Surface area A
(iii) Orientation of area with respect to the electric field .
If N is the number of electric field lines passing through the Area then
\displaystyle N\propto \vec{E} —————(i)
And \displaystyle N\propto \vec{A} —————(ii)
On combining (i) and (ii) we get
\displaystyle N\propto \vec{E}.\vec{A}
Here the term \displaystyle \vec{E}.\vec{A} is called electric flux and is denoted by \displaystyle \phi . So we can define electric flux as the dot product of electric field vector and area vector. The number of electric field lines passing through the surface area depends on the electric flux.
Hence \displaystyle \phi =\vec{E}.\vec{A}
Or \displaystyle \phi =EA\cos \theta .
Here \displaystyle A\cos \theta is the projection of area A onto a plane oriented perpendicular to the electric field as shown in the diagram given below and \displaystyle \theta is the angle between electric field vector and normal to the area.
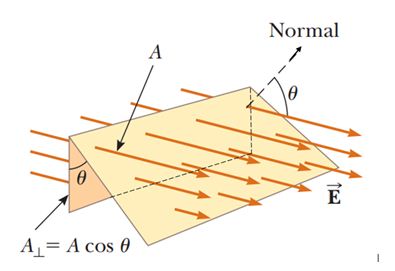
From the SI units of E and A, we see that \displaystyle \phi has units of N.m2/C. Also remember that \displaystyle \phi is a scalar quantity.
From the equation \displaystyle \phi =EA\cos \theta we can conclude that
(i)
when θ = 0 that is the normal to area is parallel to the electric field or the plane of the area is perpendicular to the field , the flux through the area is maximum
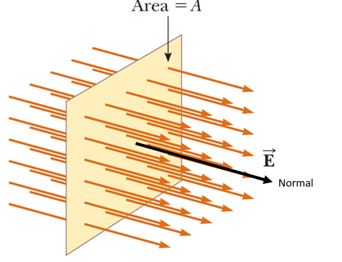
(ii)
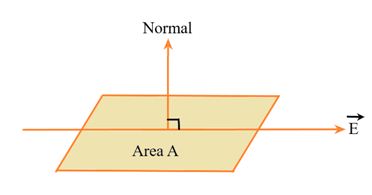
When θ =90o the flux passing through the area is zero.
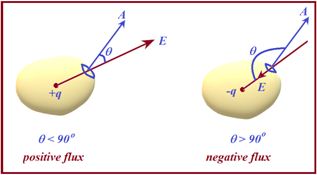
In case of closed surface the flux may be positive or negative depending on the direction of field lines and the angle between the normal and the electric field as shown in the diagram given below. In the diagram there are two close surfaces. The first one contains a positive charge and the second contains a negative charge. In the first case the angle between the normal to surface and the electric field is less than 90o. So according to formula \displaystyle \phi =EA\cos \theta , the flux is positive. whereas in the second case the flux is negative because θ is more than 90o.
Important Points
(i)
For a closed body outward flux is taken to be positive [Fig. (A)] while inward negative
[Fig. (B)]

(ii)
The electric flux is contributed by only that charge which is enclosed by the surface. If a charge is lying outside the closed surface the net electric flux passing the closed surface is zero whereas electric field is produced by all the charges whether inside on outside.
Practice Questions (Basic Level)
Q.1
The electric field in a region of space is given by = N/C. The flux of due to this field through an area 2 m2 lying in the y-z plane, in SI units, is
(a) 10
(b) 20
(c) 10 \sqrt{2}
(d) 2 \sqrt{{29}}
Ans. (a)
Q.2
Figure shows a closed surface which intersects a conducting sphere. If a positive charged is placed at the point P, the flux of the electric field through the closed surface
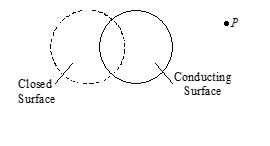
(a) will remain zero
(b) will become positive
(c) will become negative
(d) will become undefined
Ans. (b)
Q.3
In a region of space, the electric field is in the x-derection and proportional to s, i.e., =E0xî. consider an imaginary cubical volume of edge a, with its edges parallel to the axes of coordinates. The charge inside this volume is
(a) zero
(b) e0E0a3
(c) \frac{1}{{\varepsilon 0}} E0a3
(d) \frac{1}{6}e0E0a2
Ans. (b)
Q.4
In a region of space, the electric field is given by . The electric flux through a surface of area of 100 units in x-y plane is
(a) 800 units
(b) 300 units
(c) 400 units
(d) 1500 units
Ans. (b)
Q.5
A cylinder of length L and radius b has its axis coincident with the x The electric field in this region is E = 200j. Find the flux through thebottom of the cylinder.
(a)– 200 pb2
(b)– 700 pb2
(c)-400bL
(d) –100 pb2
Ans: (c)
Q.6
Two oppositely charged parallel plates have dimensions of 10x20cm and have a charge of 20mC each. Find the electric flux and electric flux density between the two plates.
(a)0.25×106 Nm2/C , 0.125×108 N/C
(b) 25×106 Nm2/C , 125×108 N/C
(c) 2.25×106 Nm2/C , 1.125×108 N/C
(d)35×106 Nm2/C , 2×108 N/C
Ans: (c)
7.
A long string with a charge of \lambda per unit length passes through an imaginary cube of edge a. The maximum flux of the electric field through the cube will be
(a) \lambda a/{{\varepsilon }_{0}}
(b) \frac{{\sqrt{2}\,\lambda a}}{{{{\varepsilon }_{0}}}}
(c) \frac{{6\lambda {{a}^{2}}}}{{{{\varepsilon }_{0}}}}
(d) \frac{{\sqrt{3}\,\lambda a}}{{{{\varepsilon }_{0}}}}
Ans (d)
Practice Questions (JEE Main Level)
Q.1
A positive charge Q is brought near an isolated metal cube.
(a) The cube becomes negatively charged.
(b) The cube becomes positively charged.
(c) The interior becomes positively charged and the surface becomes negatively charged.
(d) The interior remains charge free and the surface gets non-uniform charge distribution.
Ans. (d)
Q.2
A charge Q is placed at the mouth of a conical flask. The flux of the electric field through the flask is
(a) zero
(b) Q/e0
(c) \frac{Q}{{2\varepsilon 0}}
(d) < \frac{Q}{{2\varepsilon 0}}
Ans. (c)
Q.3
A long string with a charge of l per unit length passes through an imaginary cube of edge a. The maximum flux of the electric field through the cube will be
(a) la/e0 (b) Ö2la/e0 (c) 6la2/e0 (d) Ö3la/e0
Ans. (d)
Q.4
A thin, metallic spherical shell contains a charge Q on it. A point charge q is placed at the center of the shell and another charge q1 is placed outside it as shown in figure. All the three charges are positive. The force on the charge at the center is
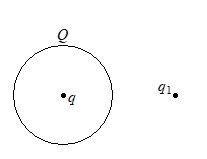
(a) towards left
(b) towards right
(c) upward
(d) zero.
Ans. (d)
Q.5
Figure shows an imaginary cube of edge L/2. A uniformly charged rod of length L moves towards left at a small but constant speed v. At t = 0, the left end just touches the center of the face of the cube opposite it. Which of the graphs shown in figure below represents the flux of the electric field through the cube as the rod goes through it?
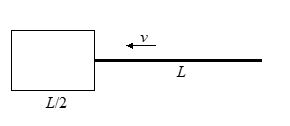

6.
A charge q is placed at the center of the open end of a cylindrical vessel. The flux of the electric field through the surface of the vessel is
(a) zero
(b) q/eo
(c) q/2eo
(d) 2q/eo
Ans. (c)
Practice Questions (JEE Advance Level)
Q.1
The electric field strength depends only on the x and y co-ordinates according to the law, E=\frac{{a(xi+yj)}}{{({{x}^{2}}+{{y}^{2}})}}, where a is a constant, i and j are the unit vectors of the x and y axes. Find the flux of E through a sphere of radius R with its centre at the origin of co-ordinates.
(a)4pak (b)2pak (c)3pak (d)6pak
Ans. (a)
Q.2
A ball of radius R is uniformly charged with the volume density r. The flux of the electric field strength vector across the ball’s section formed by the plane located at a distance r0 < R from the centre of the ball will be
(a) \frac{{\pi \rho {{r}_{0}}\left( {{{R}^{2}}-r_{0}^{2}} \right)}}{{{{\varepsilon }_{0}}}}
(b) \displaystyle \frac{{\pi \rho {{r}_{0}}\left( {{{R}^{2}}-r_{0}^{2}} \right)}}{{3{{\varepsilon }_{0}}}}
(c) \frac{{\pi \rho {{r}_{0}}\left( {{{R}^{2}}-r_{0}^{2}} \right)}}{{2{{\varepsilon }_{0}}}}
(d) zero
Ans. (b)
Q.3
A point charge Q is located on the axis of a disc of radius R at a distance a=R/\sqrt{3} from the plane of the disc. What percentage of net flux will pass through the disc?
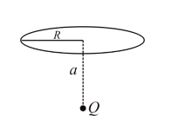
(a) 25% (b) 50%
(c) 40% (d) 20%
Ans. 25%
Q.4
An infinite, uniformly charged sheet with surface charge density s cuts through a spherical Gaussian surface of radius R at a distance x from its center, as shown in the figure. The electric flux through the Gaussian surface is
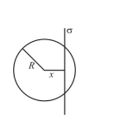
(a) \frac{{\pi {{R}^{2}}\sigma }}{{{{\varepsilon }_{0}}}}
(b) \frac{{2\pi \left( {{{R}^{2}}-{{x}^{2}}} \right)}}{{{{\varepsilon }_{0}}}}
(c) \frac{{\pi \,{{{\left( {R-x} \right)}}^{2}}\sigma }}{{{{\varepsilon }_{0}}}}
(d) \frac{{\pi \,\left( {{{R}^{2}}-{{x}^{2}}} \right)\sigma }}{{{{\varepsilon }_{0}}}}
Ans. (d)
Q.5
A hollow charged conductor has a tiny hole cut into its surface. Find the electric field in the hole If s is the surface charge density near the hole.
(a) (s/e0) ñ, where ñ is the unit vector in the outward normal direction
(b) (s/2e0) ñ, where ñ is the unit vector in the outward normal direction
(c) (3s/2e0) ñ, where ñ is the unit vector in the outward normal direction
(d) none
Ans: (b)
Q.6
If three infinite charged sheets of uniform surface charge densities s, 2s and –4s are placed as shown in figure, then find out electric field intensities at points A, B, C and D.
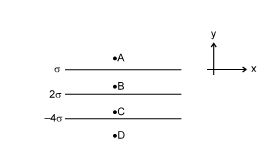
(a) {{E}_{a}}=\left( {-\frac{{\sigma {{\varepsilon }_{o}}}}{2}\hat{j}} \right)
(b) {{E}_{b}}=\left( {-\frac{{\sigma {{\varepsilon }_{o}}}}{2}\hat{j}} \right)
(c) {{E}_{c}}=\left( {-\frac{{\sigma {{\varepsilon }_{o}}}}{2}\hat{j}} \right)
(d) {{E}_{d}}=\left( {-\frac{{\sigma {{\varepsilon }_{o}}}}{2}\hat{j}} \right)
Ans:(a)
Q.7
A Uniformly charged solid non-conducting sphere of uniform volume charge density r and radius R is having a concentric spherical cavity of radius r. Find out electric field intensity at following points, as shown in the figure :
Point A ,B & C
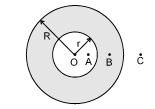
(a) \overrightarrow{{{{E}_{A}}}}\ne 0, {{\vec{E}}_{B}}=\frac{{K\frac{8}{3}\pi (O{{B}^{3}}-{{r}^{3}})\rho }}{{O{{B}^{3}}}}, {{\vec{E}}_{c}}=\frac{\rho }{{3{{\varepsilon }_{0}}}}\frac{{{{R}^{3}}-{{r}^{3}}}}{{{{{[OC]}}^{3}}}}
(b) \overrightarrow{{{{E}_{A}}}}=0, {{\vec{E}}_{B}}=\frac{{K\frac{4}{3}\pi (O{{B}^{3}}-{{r}^{3}})\rho }}{{4O{{B}^{3}}}}, {{\vec{E}}_{c}}=\frac{{4\rho }}{{3{{\varepsilon }_{0}}}}\frac{{{{R}^{3}}-{{r}^{3}}}}{{{{{[OC]}}^{3}}}}
(c) \overrightarrow{{{{E}_{A}}}}=0, {{\vec{E}}_{B}}=\frac{{K\frac{4}{3}\pi (O{{B}^{3}}-{{r}^{3}})\rho }}{{O{{B}^{3}}}}, {{\vec{E}}_{c}}=\frac{\rho }{{3{{\varepsilon }_{0}}}}\frac{{{{R}^{3}}-{{r}^{3}}}}{{{{{[OC]}}^{3}}}}
(d) none
Ans: (c)
Q.8
In above question if cavity is not concentric and centred at point P as shown in the figure. Then repeat all the steps
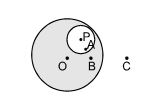
(a) \overrightarrow{{{{E}_{A}}}}=\frac{\rho }{{3{{\varepsilon }_{0}}}}\overrightarrow{{OP}}, {{\overrightarrow{E}}_{B}}=\frac{{\rho (\overrightarrow{{OB}})}}{{3{{\varepsilon }_{0}}}}+\frac{{K\left[ {\tfrac{4}{3}\pi {{r}^{3}}(-\rho )} \right]}}{{{{{[PB]}}^{3}}}}\overrightarrow{{PB}}, \overrightarrow{{{{E}_{c}}}}=\frac{{K\left[ {\tfrac{4}{3}\pi {{R}^{3}}\rho } \right]}}{{{{{[OC]}}^{3}}}}\overrightarrow{{OC}}+\frac{{K\left[ {\tfrac{4}{3}\pi {{r}^{3}}(-\rho )} \right]}}{{{{{[PC]}}^{3}}}}\overrightarrow{{PC}}
(b) \overrightarrow{{{{E}_{A}}}}=\frac{\rho }{{2{{\varepsilon }_{0}}}}\overrightarrow{{OP}}, {{\overrightarrow{E}}_{B}}=\frac{{\rho (\overrightarrow{{OB}})}}{{2{{\varepsilon }_{0}}}}+\frac{{K\left[ {\tfrac{8}{3}\pi {{r}^{3}}(-\rho )} \right]}}{{{{{[PB]}}^{3}}}}\overrightarrow{{PB}}, \overrightarrow{{{{E}_{c}}}}=\frac{{K\left[ {\tfrac{7}{3}\pi {{R}^{3}}\rho } \right]}}{{{{{[OC]}}^{3}}}}\overrightarrow{{OC}}+\frac{{K\left[ {\tfrac{4}{5}\pi {{r}^{3}}(-\rho )} \right]}}{{{{{[PC]}}^{3}}}}\overrightarrow{{PC}}
(c) \overrightarrow{{{{E}_{A}}}}=\frac{{3\rho }}{{3{{\varepsilon }_{0}}}}\overrightarrow{{OP}}, {{\overrightarrow{E}}_{B}}=\frac{{2\rho (\overrightarrow{{OB}})}}{{3{{\varepsilon }_{0}}}}+\frac{{4K\left[ {\tfrac{4}{3}\pi {{r}^{3}}(-\rho )} \right]}}{{{{{[PB]}}^{3}}}}\overrightarrow{{PB}}, \overrightarrow{{{{E}_{c}}}}=\frac{{6K\left[ {\tfrac{4}{3}\pi {{R}^{3}}\rho } \right]}}{{{{{[OC]}}^{3}}}}\overrightarrow{{OC}}+\frac{{7K\left[ {\tfrac{4}{3}\pi {{r}^{3}}(-\rho )} \right]}}{{{{{[PC]}}^{3}}}}\overrightarrow{{PC}}
(d) none
Ans: (a)
Q.9
A point charge Q is located on the axis of a disk of radius R at a distance b from the plane of the disk. Show that if one-fourth of the electric flux from the charge passes through the disk, then R = \displaystyle \sqrt{{3b}}.
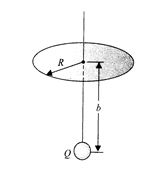
(a) \displaystyle \sqrt{b}
(b) \displaystyle \sqrt{{3b}}
(c) \displaystyle \sqrt{{2b}}
(d) \displaystyle \sqrt{{5b}}
Ans. (b)
Q.10
The electric field in a region is radially outward with magnitude \displaystyle E\,=\,ar . If a = 100 \displaystyle V{{m}^{{-2}}} and R = 0.30 m, then the value of charge contained in a sphere of radius R centred at the origin is \displaystyle W\,\times \,{{10}^{{-10}}}C. Find W.
(a) 9 (b) 3 (c) 2 (d) 15
Ans. (b)
Q.11
A ball of radius R is uniformly charged with the volume density r. The flux of the electric field strength vector across the ball’s section formed by the plane located at a distance r0 < R from the centre of the ball will be
(a) \frac{{\pi \rho {{r}_{0}}\left( {{{R}^{2}}-r_{0}^{2}} \right)}}{{{{\varepsilon }_{0}}}}
(b) \frac{{\pi \rho {{r}_{0}}\left( {{{R}^{2}}-r_{0}^{2}} \right)}}{{3{{\varepsilon }_{0}}}}
(c) \frac{{\pi \rho {{r}_{0}}\left( {{{R}^{2}}-r_{0}^{2}} \right)}}{{2{{\varepsilon }_{0}}}}
(d) zero
Ans. (b)
Q.12
The diagram shows a point charge + Q placed at the center of an imaginary hemispherical surface of radius R. A uniform electric field E has been applied in the horizontal direction. If the net flux passing through the hemispherical surface is zero then what is the magnitude of the chare + Q ?
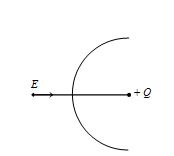
(a) 2\pi {{\varepsilon }_{0}}E{{R}^{2}}
(b) \frac{{\pi {{\varepsilon }_{0}}E{{R}^{2}}}}{2}
(c) \frac{{E{{R}^{2}}\left( {2\pi {{\varepsilon }_{0}}} \right)}}{7}
(d) \frac{{2E{{R}^{2}}}}{{{{\varepsilon }_{0}}}}
Ans. (a)
13.
An electric field line emerges from a positive point charge +q at angle \alpha to the straight line connecting it to a negative point charge -2q as shown in figure. At what angle \beta will the field line enter the charge -2q?
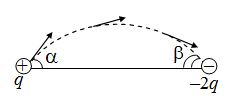
(a) \alpha
(b) 2{{\sin }^{{-1}}}\left( {\frac{1}{{\sqrt{2}}}\sin \frac{\alpha }{2}} \right)
(c) {{\sin }^{{-1}}}\left( {\frac{1}{{\sqrt{2}}}\sin \frac{\alpha }{2}} \right)
(d) {{\sin }^{{-1}}}\left( {\frac{1}{{2\sqrt{2}}}\sin \frac{\alpha }{2}} \right)
Ans (b)
14.
An electric field given by \vec{E}=4\hat{i}-3({{y}^{2}}+2)\hat{j} pierces Gaussian cube of side 1m placed at origin such that its three sides represents x, y and z axes. The net charge enclosed within the cube is
(a) 4{{\varepsilon }_{0}}
(b) 3{{\varepsilon }_{0}}
(c) 5{{\varepsilon }_{0}}
(d) zero
Ans (b)
