Video Lecture
Theory For Making Notes
WORK
The work W done by a constant force F when its point of application undergoes a displacement s is defined to be
W = F s cosθ …(1)
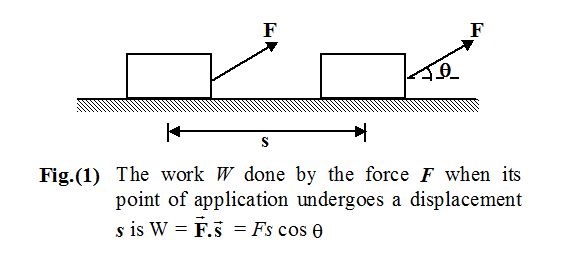
where θ is the angle between \displaystyle \vec{F} and \displaystyle \vec{s} as indicated in figure(1). Only the component of \displaystyle \vec{F} along s, that is , F cosθ, contributes to the work done. Strictly speaking, the work is done by the source or agent that applies the force. Work is a scalar quantity and its SI unit is the joule (J). From equation (1), we see that
1 J = 1 N-m
Work is also defined as the dot product of force and its displacement as given by equation (2)
W = \displaystyle \vec{F}.\vec{s}…(2)
In terms of rectangular components, the two vectors are \displaystyle \vec{F} = Fx \hat{k} + Fy \hat{j}+ Fz \hat{k}and
\displaystyle \vec{s} = Dx \hat{i} + Dy \displaystyle \hat{j} + Dz \hat{k}; hence, equation (2) may be written as
W = Fx Δx + Fy Δy + Fz Δz
The work done by a given force on a body depends only on the force, the displacement, and the angle between them. It does not depend on the velocity or the acceleration of the body, or on the presence of other forces.
Since the work is a scalar, its value also does not depend on the orientation of the coordinate axes. Since the magnitude of a displacement in a given time interval depends on the velocity of the frame of reference used to measure the displacement, the calculated work also depends on the reference frame.
Positive and Negative Work
Work done by a force may be positive or negative depending on the angle θ between the force and displacement. If the angle θ is acute (θ< 90o), then the component of force is parallel to the displacement and the work done is positive. (See figure 2 a)

If the angle θ is obtuse (θ> 90o), then the component of force is antiparallel to the displacement and the work done by force is negative (see fig.(2 b)
Zero Work Done
It is clear from the equation (1) that the work done by a force is zero when
(a) F = 0
(b) s = 0
(c) cos θ = 0 or θ = 90o
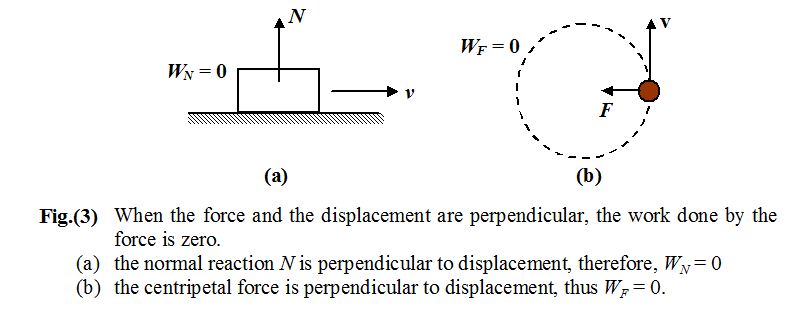
Work Done by Friction
There is a misconception that the force of friction always does negative work. In reality, the work done by friction may be zero, positive or negative depending upon the situation as shown in the figure (4).

Work Done by Gravity
Consider a block of mass m which slides down a smooth inclined plane of angle qas shown in figure(5).
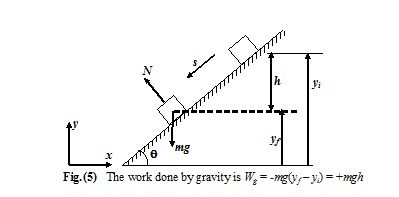
Let us assume the coordinate axes as shown in the figure, to specify the components of the two vectors – although the value of work will not depend on the orientation of the axes.
Now, the force of gravity, \displaystyle \vec{F}g = –mg \hat{j}
and the displacement is given by
\displaystyle \vec{s} = Δx \hat{i} + Δy \hat{j} + Δz \hat{k} The work done by gravity is
Wg= \displaystyle \vec{F}g . \displaystyle \vec{s} = –mg \hat{j}.(Δx \hat{i} + Δy \hat{j} + Δz \hat{k})
or Wg = –mgΔy
Since Δy = yf– yi= –h
Wg= –mg(yf– yi) = +mgh
If the block moves in the upward direction, then the work done by gravity is negative and is given by
Wg= –mgh
WORK DONE BY A VARIABLE FORCE
When the magnitude and direction of a force vary in three dimensions, it can be expressed as a function of the position vector \displaystyle \vec{F} (r), or in terms of the coordinates \displaystyle \vec{F} (x, y, z). The work done by such a force in an infinitesimal displacement ds is
dW = \displaystyle \vec{F}.d \displaystyle \vec{s}

The total work done in going from point A to point B as shown in the figure is
\displaystyle {{W}_{{A\to B}}}=\int\limits_{A}^{B}{{\vec{F}.d\vec{s}=}}\int\limits_{A}^{B}{{(F\cos \theta )ds}}
In terms of rectangular components,
\displaystyle {F} = Fx \hat{i} + Fy \hat{j} + Fz
\hat{k} and d \displaystyle {\vec{s}} = dx \displaystyle \hat{i} + dy \hat{j} + dz \hat{k}
therefore ,
WA – B= \int\limits_{{{{x}_{A}}}}^{{{{x}_{B}}}}{{{{F}_{x}}dx}}+\int\limits_{{{{y}_{A}}}}^{{{{y}_{B}}}}{{{{F}_{y}}dy}}+\int\limits_{{{{z}_{A}}}}^{{{{z}_{B}}}}{{{{F}_{z}}dz}}
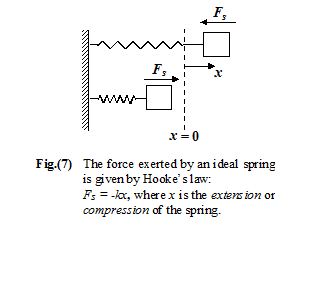
Work done by a Spring
If x be the displacement of the free end of the spring from its equilibrium position then, the magnitude of spring force is given by
Fx = -kx
The negative sign signifies that the force always opposes the extension (x> 0) or the compression (x < 0) of the spring. In other words, the force tends to restore the system to its equilibrium position
The work done by the spring force for a displacement from xito xfis given by
Ws = \int\limits_{{{{x}_{i}}}}^{{{{x}_{f}}}}{{{{F}_{s}}dx}}=-\int\limits_{{{{x}_{i}}}}^{{{{x}_{f}}}}{{kxdx}}
or Ws = -\frac{1}{2}k\left( {x_{f}^{2}-x_{i}^{2}} \right)
Note
- The work done by a spring force is negative
- The work done by the spring force only depends on the initial and final points.
- The net work done by the spring force is zero for any path that returns to the initial point.
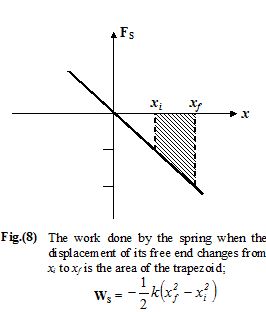
Graphically, the work done by the spring force in a displacement from xito xfis the shaded area (as shown in the figure 8)which is the difference in the areas of two triangles.
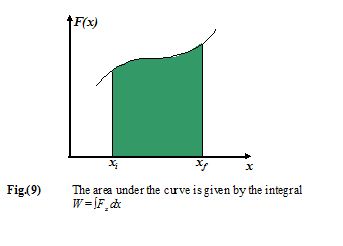
In general, the work done by a variable force F(x) from an initial point xito final point xfis given by the area under the force – displacement curve as shown in the figure (9).
Area (work) above the x – axis is taken as positive, and vice-versa.
Illustration
A box is moved over a horizontal path by applying force F = 60 N at an angle θ = 30o to the horizontal. What is the work done during the displacement of the box over a distance of 0.5 km.
Solution
By definition, W = F s cos θ
Here F = 60 N; s = 0.5 km = 500 m; θ = 30o.
W = (60)(500) cos30o = 26 kJ
Illustration
A load of mass m = 3000 kg is lifted by a winch with an acceleration a = 2 m/s2. Find the work done during the first one and a half seconds from the beginning of motion.
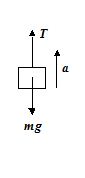
Solution
The height to which the body is lifted during the first t second is h = \frac{1}{2}a{{t}^{2}}.
The tension in the rope is given by
T = mg + ma
Work done is given by
W = Th = m(g + a) \left( {\frac{1}{2}a{{t}^{2}}} \right)
Here m = 3000kg, a = 2m/s2; g = 10m/s2; t = 1.5 s.
W = (3000)(10 + 2) \left[ {\frac{1}{2}\left( 2 \right){{{\left( {1.5} \right)}}^{2}}} \right]
or W = 81 kJ
Illustration
A block of mass 20 kg is to be raised from the bottom to the top of an incline 3 m long and 1 m off the ground at the top. Assuming the incline to be frictionless, how much work must be done by a force parallel to the incline pushing the block up? (g = 9.8 ms–2)
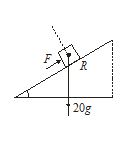
Solution
We have F = 20g sinq
and R = 20g cosq
or F=20g.\frac{1}{3}=\frac{{20g}}{3}\ \text{Newton} F=20g.\frac{1}{3}=\frac{{20g}}{3}\ \text{Newton}
Work done =Fx=\frac{{20g}}{3}.3=20\times 9.8=196\ \text{joule}.Ans.
POWER
The power delivered by an agent is the total work done by the agent divided by the total time interval
or P=\frac{{\Delta W}}{{\Delta t}}.
The instantaneous power of an agent is P=\frac{{dW}}{{dt}}
and W(\text{total work done)}=\int\limits_{{{{\mathbf{t}}_{\mathbf{1}}}}}^{{{{\mathbf{t}}_{\mathbf{2}}}}}{{Pdt.}}
We can easily find an alternative useful formula for power in terms of velocity. We have
P=\frac{{dW}}{{dt}}=\frac{{\overrightarrow{{F\,}}.\,\overrightarrow{{d\,}}x}}{{dt}}=\overrightarrow{{F\,}}.\left( {\frac{{\overrightarrow{{d\,}}x}}{{dt}}} \right)=\overrightarrow{{F\,}}.\overrightarrow{{v\,}}.
In SI the unit of power is joule per second (Js–1), which is called watt (W). The layman’s unit of power is horse power (H.P.) One horse power is equal to 746 watt.
Illustration
A block of mass 2kg is pulled up on a smooth incline of angle 30° with horizontal. If the block moves with an acceleration of 1m/s2, find the power delivered by the pulling force at a time 4 second after the motion has begun. What is the average power delivered during these four second after the motion starts?
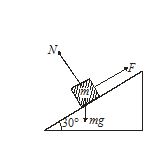
The force acting on the block are shown in figure.
Resolving forces parallel to incline,
F – mg sin θ = ma
F = mg sin q + ma
= 2 × 9.8 × sin 30° + 2 ×1
= 11.8 N
velocity = 0 + 1 × 4 = 4 m/s
Power delivered by force at t = 4 second
= Force × velocity = 11.8 × 4 = 47.2 W
The displacement during 4 second is given by the formula
v2 = u2 + 2aS
v2 = 0 + 2 × 1 × S
S = 8m
Work done in four second = Force × distance = 11.8 × 8 = 94.4J
average power delivered =Work done/time = 94.4/4 =23.6 Ans.
Practice Questions (Level-1)
Q.1
A body moves a distance of 10 m along a straight line under the action of a force of 5 N. If the work done is 25 J, the angle which the force makes with the direction of motion of the body is
(a) 0o (b) 30o (c) 60o (d) 90o
Ans. (c)
Q.2
A body of mass m is moving in a circle of radius r with a constant speed v. The force on the body is mv2/r and is directed towards the center. What is the work done by the force in moving the body half the circumference of the circle:
(a) \frac{{m{{v}^{2}}}}{r}\times \pi rac
(b) zero
(c) \frac{{m{{v}^{2}}}}{{{{r}^{2}}}}
(d) \frac{{\pi {{r}^{2}}}}{{m{{v}^{2}}}}
Ans. (b)
Q.3
A particle moves along the x–axis from x = 0 to x = 5m under the influence of a force given by F = 7 – 2x + 3x2. The work done in the process is
(a) 70 (b) 270 (c) 35 (d) 135
Ans. (d)
Q.4
A person holds a bucket of weight 60 N. He walks 10 m along the horizontal and then climbs up a vertical distance of 5 m. The work done by the man is
(a) 300 J (b) 420 J (c) 720 J (d) none of these
Ans. (a)
Q.5
An engine develops 10 kW of power. How much time will it take to lift a mass of 200 kg to a height of 40 m( g = 10m/s2)
(a) 4 s (b) 5 s (c) 8s (d) 10 s
Ans. (c)
Q.6
Under the action of a force, a 2 kg body moves such that its position x as a function of time is given by x = \frac{{{{t}^{3}}}}{3}; where x is in meters and t in seconds the work done by the force in the first two seconds is
(a) 1600 J (b) 160 J (c) 16 J (d) 1.6 J
Ans. (c)
Q.7
An electric motor creates a tension of 4500 N in a hoisting cable and reels it in at the rate of 2 m/s. What is the power of this motor
(a) 25 kW (b) 9 kW (c) 225 W (d) 9000 hp
Ans. (b)
Q.8
A particle is acted upon by a conservative force \vec{F}=\left( {7\hat{i}-6\hat{j}} \right) N (no other force is acting on the particle). Under the influence of this force particle moves from (0, 0) to (-3m, 4m), then
(a) work done by the force is 3 J
(b) work done by the force is -45 J
(c) at (0, 0) speed of the particle must be zero
(d) none of these
Ans. (b)
Q.9
Work done by force of friction
(a) can be positive
(b) can be negative
(c) can be zero
(d) all of the above
Ans. (d)
11.
A motor drives a body along a straight line with a constant force. The power P developed by the motor must vary with time t according to

Ans (d)
12.
A force \overrightarrow{F}=(5\hat{i}+3\hat{j}) Newton is applied on a particle which displaces it from its origin to the point \overrightarrow{{r\,}}=(2\hat{i}-1\hat{j})metre. The work done on the particle is
(a) – 7J
(b) +13J
(c) +7J
(d) +11J
Ans (c)
13.
A machine which is 70% efficient raises a 10 kg body through a certain distance and spends 100 J of energy. The body is then released. On reaching the ground, the kinetic energy of the body will be
(a) 50J
(b) 35J
(c) 70J
(d) 100J
Ans (c)
14.
A vehicle is moving on a straight horizontal road with a constant velocity of 10 ms–1. The engine needs to spend 4kJ of energy per second. The force on the vehicle is
(a) 0.2 kN
(b) 0.4 kN
(c) 0.6 kN
(d) 1 N
Ans (b)
15.
A train weighing 1000 ton is moving on a horizontal track with a uniform velocity of 10 ms–1. The total resistance to the motion of the train is 5 kgf per ton. If g = 10 ms–2, then the power of the engine is
(a) 200 kW
(b) 400 kW
(c) 500 kW
(d) 800 kW
Ans (c)
16.
A electric motor creates a tension of 9000 N in cable and reels it in at the rate of 2ms–1. The power of the electric motor is
(a) 18 kW
(b) 15 kW
(c) 81 W
(d) 225 W
Ans (a)
17.
A crane is used to lift 1000 kg of coal from a mine 100 m deep. The time taken by the crane is 1 hour. The efficiency of the crane is 80%. If g = 10 ms–2, then the power of the crane is
(a) 104 W
(b) 105 W
(c) \frac{{{{{10}}^{4}}}}{{36\times 8}}\text{W}
(d) \frac{{{{{10}}^{5}}}}{{36\times 8}}\text{W}
Ans (d)
Practice Questions (Level-2)
1.
A position-dependent force F=3{{x}^{2}}-2x+7 acts on a body of mass 7 kg and displace it from x = 0 m to x = 5 m. The work done on the body is x¢ joule. If both F and x are measured in SI units, the value of x¢ is
(a) 135
(b) 235
(c) 335
(d) 935
Ans (a)
2.
The F-x graph of a particle of mass 100 g is shown in figure. If the particle begins to move from rest at x = 0, its velocity at x = 12 m is
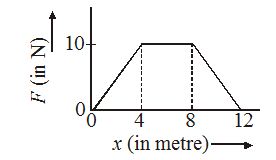
(a) 10 ms–1
(b) 20 ms–1
(c) 30 ms–1
(d) 40 ms–1
Ans (d)
3.
A plate of mass m, length b and breadth a is initially lying on a horizontal floor with length parallel to the floor and breadth perpendicular to the floor. The work done to erect it on its breadth is
(a) mg\left[ {\frac{b}{2}} \right]
(b) mg\left[ {a+\frac{b}{2}} \right]
(c) mg\left[ {\frac{{b-a}}{2}} \right]
(d) mg\left[ {\frac{{b+a}}{2}} \right]
Ans (c)
4.
Figure shows F-x graph of a particle. The work done is
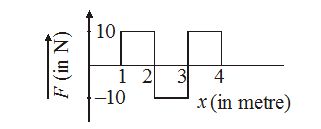
(a) 0 J
(b) 10 J
(c) 20 J
(d) 30 J
5.
A metre scale of 0.1 kg pivoted at one end is turned so that it is inclined at 30° with the horizontal in the vertical plane. The work done is [Take g = 10 ms–2]
(a) 0.05 joule
(b) 0.025 joule
(c) 0.25 joule
(d) 0.5 joule
Ans (c)
6.
The force F acting on a body moving in a circle of radius r is always perpendicular to the instantaneous velocity v. The work done by the force on the body in one complete rotation is
(a) Fv
(b) F.2pr
(c) Fr
(d) zero
Ans (d)
7.
Water is pouring down from a waterfall at the rate of 75 kg s–1 on the blades of a turbine. If the height of the ‘fall’ be 100m, then the power delivered to the turbine in nearly
(a) 95 kW
(b) 95 Kw
(c) 100 kW
(d) 0 kW
Ans (d)
8.
A uniform chain of length L and mass M overhangs a horizontal table with its two third part on the table. The friction coefficient between the table and the chain is m. The work done by the friction during the period the chain slips off the table is
(a) -\frac{1}{4}\mu MgL
(b) -\frac{2}{9}\mu MgL
(c) -\frac{4}{9}\mu MgL
(d) -\frac{6}{7}\mu MgL
Ans (b)
9.
Water is drawn from a well in a 5kg drum of capacity 55 litre by two ropes connected to the top of the drum. The linear mass density of each rope is 0.5 kgm–1. The work done in lifting water to the ground from the surface of water in the well 20 m below is [g = 10 ms–2]
(a) 1.4\times {{10}^{4}}\text{J}
(b) 1.5\times {{10}^{4}}\text{J}
(c) 9.8\times 10\times 6\text{J}
(d) 18J
Ans (a)
10.
A one-ton car moves with a constant velocity of 15 ms–1 on a rough horizontal road. The total resistance to the motion of the car is 12 % of the weight of the car. The power required to keep the car moving with the same constant velocity of 15 ms–1 is [Take g =10 ms–2]
(a) 9 kW
(b) 18 kW
(c) 24 kW
(d) 36 kW
Ans (b)
11.
A bullet of mass 10g leaves a rifle at an initial velocity of 1000 ms–1 and strikes the earth at the same level with a velocity of 500 ms–1. The work done in overcoming the resistance of air will be
(a) 500 J
(b) 375 J
(c) 3750 J
(d) 5000 J
Ans (c)
12.
A cord is used to lower vertically a block of mass M through a distance d at a constant downward acceleration g/4. Find the work done by the cord on the block.
(a) \frac{4}{9}Mgd
(b) \frac{-1}{4}Mgd
(c) \frac{1}{4}Mgd
(d) \frac{3}{8}Mgd
Ans.(c)
Comprehension Based Question ( 13 to 16)
A block of mass 3.57 kg is drawn at a constant speed a distance 4.06 m along a horizontal floor by a rope exerting a constant force of 7.68 Newton making 15° wit the horizontal. Calculate
13.
the total work done on the block,
(a) 5
(b) 20
(c) 0
(d) 1
Ans (c)
14.
the work done by the rope on the block,
(a) 0 J
(b) -30.1 J
(c) 31.5 J
(d) 30.1 J
Ans (d)
15.
the work done by friction on the block and
(a) -30.1 J
(b) 0 J
(c) 32.0 J
(d) 30.1 J
Ans (a)
16.
the coefficient of kinetic friction.
(a) 0.5
(b) 0.225
(c) 0.20
(d) 0.038
Ans (b)
Comprehension Based Question ( 17 to 19)
A body of mass 0.3 kg is taken up an inclined plane of length 10m and height 5m, and then allowed to slide down to the bottom again. The coefficient of friction between the body and the plane is 0.15. What is the
17.
Work done by the gravitational force over the round tip?
(a)1
(b)-5
(c)5
(d) Zero
Ans (d)
18.
Work done by the applied force on the upward journey?
(a) 18.5J
(b) 19.0J
(c) 17.0J
(d) 18.0J
Ans (a)
19.
Work done by the frictional force over the round trip,
(a) 9.3J
(b) –9.3J
(c) –7.6J
(d) 7.6J
Ans (C)
20.
Kinetic energy of the body at the end of the trip?
(a) 9.2J
(b) 10.9J
(c) 12.0 J
(d) 11.5 J
Ans (b)
21.
A particle moves from a point \overrightarrow{{{{r}_{1}}}}=(2\hat{i}+3\hat{j}) to another point \overrightarrow{{{{r}_{2}}}}=(3\hat{i}+2\hat{j}) during which a certain force \overrightarrow{F}=(5\hat{i}+5\hat{j}) acts on it. Calculate work down by the force on the particle during this displacement.
(a) 0
(b) 10
(c) -5
(d) 2
Ans (a)
22.
A particle moves along the x-axis from x = 0 to x = 5m under the influence of a force given by F=7-2x+3{{x}^{2}}. Calculate the work done.
(a) 100J
(b) 135J
(c) 230J
(d) 150J
Ans (b)
23.
A water pump lifts water from a level 10 m below the ground. Water is pumped at a rate of 30 kg/minute with negligible velocity. Calculate the minimum horsepower the engine should have to do this?
(a) 3.2 × 10–2 H.P
(b) 6.6 × 10–9 H.P
(c) 6.6 × 10–2 H.P
(d) 6.6 × 10–4 H.P
Ans(d)
Comprehension Based Question (24 and 25)
A man weighing 55 kg supports a body of 20 kg on his head. Calculate work done by him if he moves a distance of 20m
24.
on a horizontal road
(a) 19
(b)2
(c) Zero
(d) 5
Ans (c)
25.
upon a smooth incline of 1 in 5. Take g = 10ms–2.
(a) 5500J
(b) 3238J
(c) 5000J
(d) 3000J
Ans(d)
26.
An electron and a proton are detected in a cosmic ray experiment, the first with kinetic energy 10 keV and the second with 100 keV. Which is faster, the electron or proton? Obtain the ratio of their speeds. Take mass of electron = 9.11×10–31 kg, mass of proton= 1 .67 × 10–27 kg and 1 keV = 1.6 × 10–16J.
(a)14.83
(b)13.29
(c) 13.53
(d) 12.54
Ans (c)
Comprehension Based Question (27 to 29)
A car of mass 1000 kg accelerates uniformly fro rest to a velocity of 54 km/h in 5 second. Calculate
27.
its acceleration
(a) 3ms–2
(b)9ms–2
(c)2ms–2
(d)7ms–2
Ans (a)
28.
its gain in K.E.
(a) 1.227 ×105 J
(b) 1.125 ×105 J
(c) 1.058 ×105 J
(d) 1.5 ×102 J
Ans (b)
29.
average power of the engine during this period, neglect friction.
(a) 21000 W
(b) 22000 W
(c) 22856 W
(d) 22500 W
Ans(d)
Q.30
The relation between the displacement x of an object produced by a variable force F is represented by a graph shown in figure. If the object undergoes a displacement from x = 0 m to x = 2 m, the work done (in joule) will be approximately equal to

(a) 16 (b) 25 (c) 8 (d) 1.6
Ans. (b)
Q.31
A body is moved along a straight line by a machine delivering constant power. The distance moved by the body in time t is proportional to
(a) t1/2 (b) t3/4 (c) t3/2 (d) t2
Ans. (c)
Q.32
An object of mass m is tied to a string of length L and a variable horizontal force is applied on it which starts at zero and gradually increases until the string makes an angle q with the vertical . Work done by the force F is
(a) mgL(1 – sinq)
(b) mgL
(c) mgL(1 – cosq)
(d) mgL(1 + cosq)
Ans. (c)
Q.33
A bucket tied to a string is lowered at a constant acceleration of g/4. If the mass of the bucket is m and is lowered by a distance d, the work done by the string will be (assume the string to be massless)
(a) \frac{1}{4}mgd
(b) -\frac{3}{4}mgd
(c) -\frac{4}{3}mgd
(d) \frac{4}{3}mgd
Ans. (b)
Q.34
A body of mass m is projected at an angle q with the horizontal with an initial velocity u. The mean power of gravity over the whole time of journey is
(a) mg cos q
(b) \frac{1}{2}mg\sqrt{{u\cos \theta }}
(c) \frac{1}{2}mgu\sin \theta
(d) zero
Ans. (d)