Video Lecture
Theory For Notes Making
BIOT- SAVART LAW
The Biot-Savart law is a mathematical description of the magnetic field d \vec{B} that arises from a current I flowing along an infinitesimal path element d \vec{l}
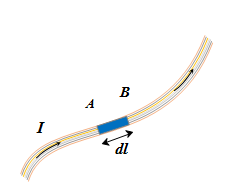
It is the product of current and length of infinitesimal segment of current carrying wire.
Current element AB =Id \vec{l}
The current element is taken as a vector quantity. Its direction is same as the direction of current.
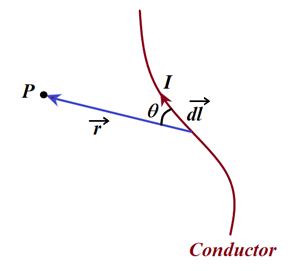
Consider a point P near a conductor carrying current I . Let there is a small length d \vec{l} of conductor whose magnetic field is d \vec{B} at point P.
The nature and magnitude of magnetic field is given by the following four points
1.
The magnetic field grows weaker as we move farther from its source. In particular, the magnitude of the magnetic field d \vec{B} is inversely proportional to the square of the distance from the current element Id \vec{l}.
2.
The larger the electric current, the larger is the magnetic field. In particular, the magnitude of the magnetic field d \vec{B} is proportional to the current I.
3.
The magnitude of the magnetic field d \vec{B} is proportional to sin q, where q is the angle between the direction of current element Id \vec{l} and vector \hat{r} that points from the current element to the point in space where dB is evaluated.
4.
The direction of the \vec{B} -is not radially away from its source as the gravitational field and the electric field are from their sources. In fact, the direction of d \vec{B} is perpendicularto both Idl and the vector \hat{r} .
These four points of the magnetic field can be combined and written mathematically as
d \vec{B} = \frac{{{{\mu }_{0}}}}{{4\pi }}\ \frac{{Id\vec{l}\ \times \ \vec{r}}}{{{{r}^{3}}}}
or d \vec{B} = \frac{{{{\mu }_{0}}}}{{4\pi }}\ \frac{{Id\vec{l}\ \times \ \hat{r}}}{{{{r}^{2}}}} …(i)
where \hat{r} is a unit vector that points from the current element to the point in space where d \vec{B} is to be evaluated. The constant mo is called the permeability of free space or the permeability constant. Its value is defined to be
μo = 4π x 10–7 T m/A
The direction of the magnetic field element dB as given by Biot Savart law is shown in the figure.
The direction of \vec{B} can be obtained from the cross product d\vec{l}\ \times \ \vec{r} given in equation (i) using right hand thumb rule.
Some important features of Biot-Savart’s law:
1.
BiotSavart’s law is applicable only to a very small length of conductor carrying current.
2.
The direction of d \vec{B} is perpendicular to both d \vec{l} and \vec{r} .
3.
If θ = 0°, i.e. point P lies on the axis of the linear conductor carrying current, then
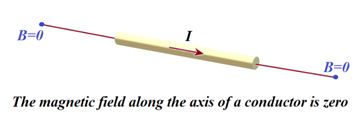
4.
If θ = 90°, i.e. the point P lies at a perpendicular position w.r.t. to current, then
dB = \frac{{{{\mu }_{0}}}}{{4\pi }}\frac{{Id\ell }}{{{{r}^{2}}}} , which is maximum.
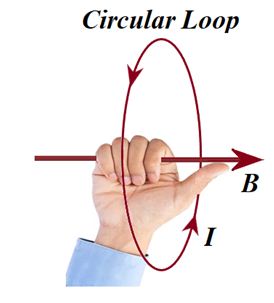
Right hand thumb rule :
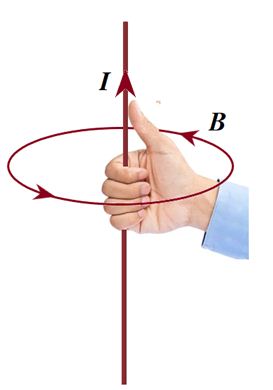
According to this rule if a straight current carrying conductor is held in the right hand such that the thumb of the hand represents the direction of current flow, then the direction of folding fingers will represent the direction of magnetic lines of force.
Right hand thumb rule of circular currents : According to this rule if the direction of current in circular conducting coil is in the direction of folding fingers of right hand, then the direction of magnetic field will be in the direction of stretched thumb
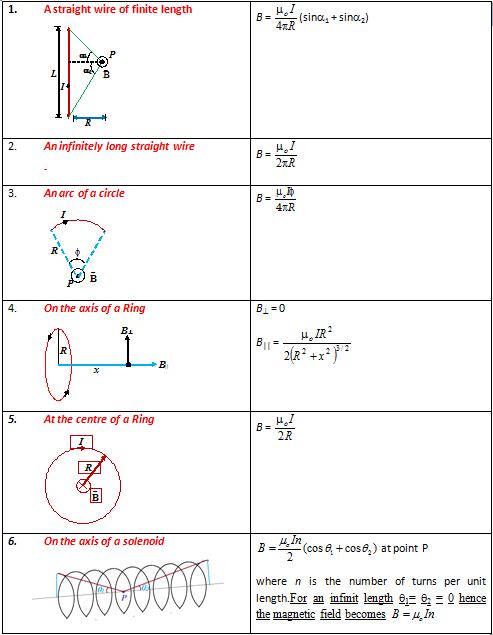
Applications Of Biot-Savart Law
Magnetic field due to different current systems
Please Note that here we are giving only the final formula of magnetic field in different cases. For detailed derivation kindly refer to the video lectures
Illustration
Two circular coils made of similar wires but of radii 20 cm and 40 cm are connected in parallel. The ratio of the magnetic field at their centres is
(1) 4 : 1
(2) 1 : 4
(3) 2 : 1
(4) 1 : 2
Solution:
B = B=\frac{{{{\mu }_{0}}}}{{4\mu }}\cdot \frac{{2\pi ni}}{r}=\frac{{{{\mu }_{0}}}}{{4\pi }}\cdot \frac{{2\pi n}}{r}\cdot \frac{V}{{2\pi mx}} (x = resistance per metre)
or B ∝ \frac{V}{{{{r}^{2}}}} (the potential difference V is same for both coils)
or B{{r}^{2}}= Constant \frac{{{{B}_{1}}}}{{{{B}_{2}}}}=\frac{{r_{2}^{2}}}{{r_{1}^{2}}}={{\left( {\frac{{40}}{{20}}} \right)}^{2}} =\frac{4}{1}
∴ (1)
Illustration
In a hydrogen atom, the electron is making 6.6 x 1015 revolutions per second in a circular orbit of radius 0.53 Å. The field of induction produced at the position of the nucleus is approximately
(1) zero
(2) 3πT
(3) 4πT
(4) 0.4πT
Solution:
i= Ne = (6.6\times {{10}^{5}}\times 1.6\times {{10}^{{-19}}})\ amp
B = \frac{{{{\mu }_{0}}}}{{4\pi }}\cdot \frac{{2\pi ni}}{r}
= {{10}^{{-7}}}\times 2\pi \times (1)\frac{{(6.6\times {{{10}}^{5}}\times 1.6\times {{{10}}^{{-19}}})}}{{0.53\times {{{10}}^{{-10}}}}} = 4π tesla
∴ (3)
Illustration
The conductor abcd carries a current of 4 A. The field at the centre O of the circular part of it is
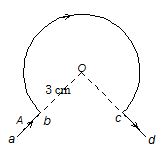
(1) \frac{{8\pi }}{3}\ \times \ {{10}^{{-5}}}\,T
(2) 2p x 10–5T
(3) \frac{{8\pi }}{3}\ \times \ {{10}^{{-4}}}\,T
(4) 2p x 10–4T
Solution:
2\pi \times {{10}^{{-5}}}T
B = \frac{{{{\mu }_{0}}}}{{4\pi }}\cdot \frac{{2\pi ni}}{r}
= 1{{0}^{{-7}}}\times \frac{{2\pi \times \frac{3}{4}\times 4}}{{3\times 1{{0}^{{-2}}}}}
∴ (2)
Illustration
A piece of wire carrying a current 6 amp. is bent in the form of a circular arc of radius 10 cm and it subtends an angle of 120° at centre. Find the flux density at the centre.
(1) 1.26 x 10–5 tesla
(2) 2.52 x 10–5 tesla
(3) 0.63 x 10–5 tesla
(4) 10–5 tesla
Solution:
Flux density due to full circular line =\ \frac{{{{\mu }_{0}}i}}{{2a}}
The arc AC is only 1/3 of the circular line
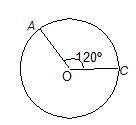
B= \frac{1}{3}\ \times \ \frac{{{{\mu }_{0}}i}}{{2a}}\ =\ \frac{{{{\mu }_{0}}i}}{{6a}}
= \frac{{4\pi \ \times \ {{{10}}^{{-7}}}\times \ 6}}{{6\ \times \ {{{10}}^{{-1}}}}}
= 4π x 10–6
= 3.14 x 4 x 10–6
= 1.26 x 10–5 tesla
∴ (1)
Illustration
A cell is connected across two points P and Q of a uniform circular conductor. Prove that the magnetic field at its center O will be zero.
Solution:
Let {{l}_{1}},\,\,{{l}_{2}} be the lengths of the two parts PRQ and PSQ of the conductor and \rho be the resistance per unit length of the conductor.

The resistance of the portion PRQ will be, {{R}_{1}}={{l}_{1}}\rho
The resistance of the portion PRQ will be {{R}_{2}}={{l}_{2}}\rho . Let {{I}_{1}},\,\,{{I}_{2}} be the current in PRQ and PSQ respectively. Since these parts are in parallel, the potential differnce across their ends will be same
{{I}_{1}}{{R}_{1}}={{I}_{2}}{{R}_{2}}
Or {{I}_{1}}{{l}_{1}}\rho ={{I}_{2}}{{l}_{2}}\rho
or {{I}_{1}}{{l}_{1}}={{I}_{2}}{{l}_{2}} …(i)
According to Biot-Savart’s law, the magnetic fields at the center O due to current through circular conductors PRQ and PSQ will be
{{B}_{1}}=\frac{{{{\mu }_{1}}}}{{4\pi }}\frac{{{{I}_{1}}{{l}_{1}}\,\sin 90{}^\circ }}{{{{r}^{2}}}}
and {{B}_{2}}=\frac{{{{\mu }_{0}}}}{{4\pi }}\frac{{{{I}_{2}}{{l}_{2}}\,\sin 90{}^\circ }}{{{{r}^{2}}}}
Since, {{I}_{1}}{{l}_{1}}={{I}_{2}}{{l}_{2}}
{{B}_{1}}={{B}_{2}}
According to right hand rule, the directions of {{B}_{1}} and {{B}_{2}} are opposite to each other, Hence, the resultant magnetic field at O will be zero.
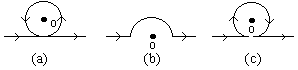
Illustration
Find the magnetic field induction at a point O if the current carrying wire has the shape shown in figure (a), (b), (c). The radius of the curved part of the wire is , the linear parts are assumed to be very long.

Solution:
(a)
Magnetic field induction at O due to current through straight wires PQ and RS is zero. The magnetic field induction at O due to current through semicircular part QR of wire is given by
{{B}_{1}}=\frac{{{{\mu }_{o}}}}{{4\pi }}\times \frac{{\pi i}}{r} (It acts \bot r to the plane of the current loop downwards).
B=0+\frac{{{{\mu }_{o}}}}{4}\times \frac{i}{r}=\frac{{{{\mu }_{o}}}}{4}\times \frac{i}{r}
(b)
Magnetic field induction at O due to current through straight portion PQ.
{{B}_{1}}=\frac{{{{\mu }_{o}}}}{{4\pi }}\times \frac{i}{r}\,(\sin 0{}^\circ +\sin 90{}^\circ )=\frac{{{{\mu }_{o}}}}{{4\pi }}\frac{i}{r} acting \bot r to loop downwards.
Magnetic field induction at O due to current through curved part QR of the wire is
{{B}_{2}}=\frac{{{{\mu }_{o}}}}{{4\pi }}\times \frac{{3\pi }}{2}\times \frac{i}{r}=\frac{{{{\mu }_{o}}}}{{4\pi }}\frac{i}{r}\times \frac{{3\pi ;}}{2} acting \bot r to loop downwards
Magnetic field induction at O due to current through straight wire RS is zero.
B={{B}_{1}}+{{B}_{2}}=\frac{{{{\mu }_{o}}}}{{4\pi }}\frac{i}{r}+\frac{{{{\mu }_{o}}}}{{4\pi }}\frac{i}{r}\times \frac{{3\pi }}{2}=\frac{{{{\mu }_{o}}}}{{4\pi }}\frac{i}{r}\left( {1+\frac{{3\pi }}{2}} \right)
(c)
In this case, the effective magnetic field induction at O due to current through the entire wire will be
B=\frac{{{{\mu }_{o}}}}{{4\pi }}\frac{i}{r}+\frac{{{{\mu }_{o}}}}{{4\pi }}\frac{{\pi i}}{r}+\frac{{{{\mu }_{o}}}}{{4\pi }}\frac{i}{r}=\frac{{{{\mu }_{o}}}}{{4\pi }}\frac{i}{r}(2+\pi )
Objective Assignment
Q.1
Two infinitely long wires carrying current i are bent, as shown in the figure. The magnetic induction at the centre C is equal to
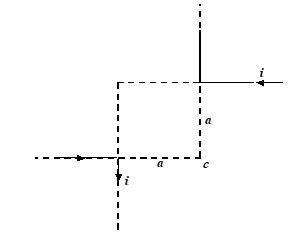
(a) zero
(b) \frac{{{{\mu }_{o}}I}}{{2\pi a}}
(c) \frac{{{{\mu }_{o}}I}}{{4\pi a}}
(d) \frac{{{{\mu }_{o}}I}}{{\pi a}}
Ans. (b)
Q.2
If the resistance of the upper half of a rigid loop is twice of that of the lower half, the magnitude of magnetic induction at the centre is equal to
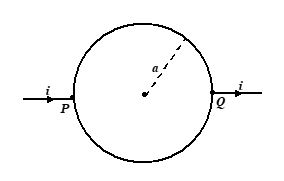
(a) zero
(b \frac{{{{\mu }_{o}}I}}{{4a}}
(c) \frac{{{{\mu}_{o}}I}}{{12a}}
(d) none of these.
Q.3
The resulting magnetic field at the point ‘O’ due to the current carrying wire shown in the figure
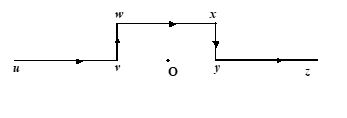
(a) points vertically upwards
(b) points vertically downwards
(c) is zero
(d) is the same as due to the segment wx alone.
Q.4.
The magnetic field at the centre of a current-carrying circular coil depends on the radius R of the coil as
(a) R (b) R2 (c) 1/R (d) 1/R2
Ans : (c)
Q.5
The value of the magnetic field at a distance x from a long straight current-carrying conductor is proportional to:
(a) x (b) x2 (c) 1/x2 (d) 1/x
Ans : (d)
Q.6
A circular coil has one turn and carries a current I. The same wire is wound into a smaller coil of 4 turns and the same current is passed through it. The field at the centre
(a) decreases to \frac{1}{4} the value
(b) is the same
(c) increases to 16 time the value
(d) decreases to 16 times the value
Ans : (c)
Q.7
A battery is connected between two points A and B on the circumference of a uniform conducting ring of radius r and resistance R. One arc AB of the ring subtends an angle q at the centre. The value of the magnetic induction at the centre due to the current in the ring is
(a) proportional to 2(180o – q)
(b) inversely proportional to r
(c) zero only if q = 180o
(d) zero for all values of q
Ans : (d)
8.
Two infinitely long, parallel wires carry equal currents of 2.5 amp in the same direction. The conductors are 5 cm apart. Calculate the field (B–field) at the point which lies
(i)
at 2.5 cm from both the wires
(a)Zero
(b)1
(c)2
(d)5
Ans (a)
(ii)
at 5 cm from both the wires
(a) {{10}^{{5}}}\,\,tesla
(b) {{10}^{{-5}}}\,\,tesla
(c) {{10}^{{-4}}}\,\,tesla
(d) {{10}^{{-2}}}\,\,tesla
Ans (b)
(iii)
10 cm apart from the first conductor towards the second conductors.
(a) 1.0\times {{10}^{{-5}}}\,\,tesla
(b) 2.5\times {{10}^{{-5}}}\,\,tesla
(c) 1.5\times {{10}^{{-5}}}\,\,tesla
(d) 3.0\times {{10}^{{-5}}}\,\,tesla
Ans (c)
9.
Four long copper wires, parallel to each other are perpendicular to the plane of the paper, their cross-sections forming a square 20 cm on edge. A 20 ampere current is set in each wire. The currents through the conductors at the corners B and C are perpendicular into the plane of the paper and those at the corners A and D are perpendicular out of the plane of the paper. What is the magnitude and the direction of the field at the center of the square?
(a) 8\times {{10}^{{-5}}}\,\,\mathbf{Tesla}
(b) 8\times {{10}^{{-5}}}\,\,\mathbf{Tesla}
(c) 8\times {{10}^{{-5}}}\,\,\mathbf{Tesla}
(d) 8\times {{10}^{{-5}}}\,\,\mathbf{Tesla}
Ans (C)
10.
Calculate the magnetic field at the center of a square 10 cm on each side when 1.5 ampere – current passes through it.
(a) 2.5\times {{10}^{{5}}}T
(b) 2.5\times {{10}^{{-5}}}T
(c) 1.7\times {{10}^{{5}}}T
(d) 1.7\times {{10}^{{-5}}}T
Ans (d)
Subjective Assignment
Q.1.
Define constant \displaystyle \mu of a medium for magnetic field. Also give its dimensional formula.
Q.2.
What is the ratio of electric and magnetic force under identical conditions.
Q.3.
What is magnetic dipole moment? Is it a scaler or vector quantity ?
Q.4.
Is magnetic induction a scalar or vector ? What are S.I. and C.G.S. units of magnetic field
Q.5.
What is dimensional formula for B? Define one tesla.
Q.6.
Calculate the magnetic field B at a distance of 10 cm from a long straight wire carrying a current of 5 A.
Q.7.
Write Biot-Savart law. Explain the symbols used.
Q.8.
Derive an expression for the magnetic field intensity at a point due to a current carrying straight wire of infinite length.
Q.9.
Derive an expression for the magnetic field at the centre of a circular current carrying coil.
Q.10.
Using Biot-Savart law, derive an expression for the magnetic field at the centre of a circular coil of radius ‘r’, having ‘N’ number of turns. Indicate the direction of the magnetic field.
Q.11.
Explain Biot—Savart law. Obtain an expression for the magnetic field at a point situated at x meter away from center of a circular coil of N turns and of r meter radius carrying a current of I ampere.
Q.12.
Find the net magnetic field at the point `O’ in each
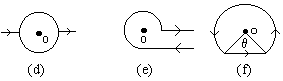

Q.13.
Find the magnetic field at the center of a ractangle whose diagonal is 16cm and the angle between the diagonals is 30 o. The current flowing is 5amp.
Q.14.
A thin disc of radius r has a charge Q distributed all over its surface.It is rotating at a speed of n rotations per sec about its own axis.Find the magnetic induction at the center of the disc
Q.15.
A thin insulating wire form a spiral of N turns in the same plane.A current I is passed through the spiral whose inner and outer radii are a and b respectively.Find the magnetic induction at the center of the spiral coil.
Q.16.
Two concentric coils of radius 10cm and 20cm are placed in the same plane in such a way that the current in the outer coil is 10A clockwise and the curent in the inner coil is 15A in the anticlockwise direction when viewed from the same place.Give magnitude of net magnetic field at the common center of the coils.
Q.17.
Two parallel conductors are placesd 50 cm apart and carrying a current of 10amp and 20 amp in the same direction. Find the net magnetic field at a point
(a) mid way between them.
(b) at a distance of 30cm from the first and 40cm from the second conductor.
Q.18.
Two circular loops of radius 10cm and 30cm are placed with their centers coinciding with each other but in mutually perpendicular planes. If they carry currents of 20amp and 10amp then find the net magnetic field at their common canter.
Q.19.
If in the previous question the planes of the two loops are making 60o with each other then find the net magnetic field again.
Q.20.
Two long straight wires P and Q are 10cm apart .The wires being perpendicular to the plane of paper. The current in P is into the plane and is 6amp.Find the direction and value of the current in the wire Q for a null point at R. Then find the magnetic induction at S & T
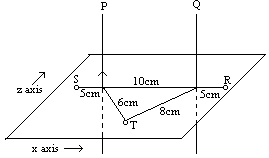
Q.21.
An electron is moving in a circular orbit of radius 1Ao with a frequency of 105 Hz. Find the magnetic field at
(a) the center of the orbit
(b) at an axial point at a distance of 2Ao from the center.
Q.22.
If a proton is fixed at a point and an electron is revolving around it in a circular path of radius 0.35A.Find the magnetic induction at the position of proton.
Q.23
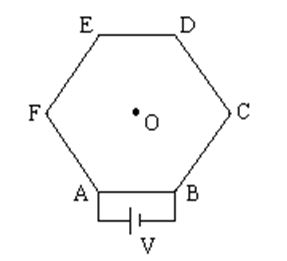
A hexagonal conducting loop abcdefa is connected ot battery of V volts at the points A and B as shown. Find the magnetic induction at the center O of the hexagon. Each side of the hexagon is of length a.
Q.24
A current I = 1.00 A circulates in a round thin-wire loop of radius R = 100 mm. Find the magnetic induction
(a) at the center of the loop;
(b) at the point lying on the axis of the loop at a distance x = 100 mm from its center
Q.25
Find the magnetic moment of a thin round loop with current if the radius of the loop is equal to R = 100 mm and magnetic induction at its center is equal to B = 6.0 \displaystyle \mu T.
Q.26
A circular coil of 200 mm diameter is made of 100 turns of thin wire and carries a current of 50 mA. Find the magnetic field induction in the center of the coil and on the coil’s axis 100 mm away from its center.
Q.27
A very long wire carrying a current I = 5.0 A is bent at right angle. Find the magnetic induction at a point lying on a perpendicular to the wire, drawn through the point of bending, at a distance I = 35 cm from it.
Q.28
A long straight conductor passes vertically through the center of a laboratory ; the direction of the current is upward. The magnetic field at a point 0.20 m from the wire is found to have magnitude \displaystyle 5.0\times {{10}^{4}}T. What is the current in the conductor.
(b) ?
Q.29
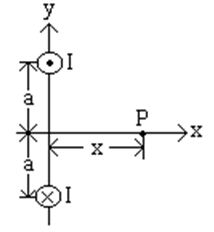
Figure is an end view of two long parallel wires perpendicular to the xy-plane, each carrying a current I. but in opposite direction.
(a) Copy the diagram, and show by vectors B field of each wire, and the resultant B field at point P.
(b) Construct a graph of the magnitude of B at any point on the x-axis.
(c) Derive an expression for the magnetic field at any point on the x axis.
(d) At what value of x be the magnetic field maximum.
Q.30
Repeat the previous problem. If the current in both wire is away from the reader.
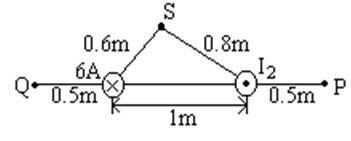
Q.31
Two long straight, parallel wires are 1.0m apart, as in Fig. The crossed wire carries a current of 6 A into the plane of the paper.
(a) What must be the magnitude and direction of the current I2 for the resultant field at point P to be zero?
(b) What is them the resultant field at Q & S ?
Q.32
Consider two parallel co-axial circular coils of equal radius R and number of turns N, carrying equal currents in the same direction and separated by a distance R. Show that the field on the axis around the mid point between the coils is uniform aver a distance small compared to R, and is given by
B = 0.72 m \displaystyle \mu NI/R
Q.33.
Write Biot-Savart expression for the magnetic field due to a current element. Calculate the force per unit length on a long straight wire carrying current of 4 A due to a parallel wire carrying 6A current. Distance between the wires = 3 cm.
[Ans. 1.6xl05Nm-1]
