Video Lecture
Theory For Making Notes
Lorem ipsum dolor sit amet, consectetur adipiscing elit. Ut elit tellus, luctus nec ullamcorper mattis, pulvinar dapibus leo.
Practice Questions (Level-1)
1.
In a head-on collision between an a-particle and a gold nucleus, the minimum distance of approach is 3.95 × 10–14 m. Calculate the energy of the a-particle.
(a) 1MeV
(b) 6MeV
(c) 2MeV
(d) 10MeV
Ans (b)
2.
In an atom, two electrons move around the nucleus in circular orbits of radii R and 4R. The ratio of the time taken by them to complete one revolution is
(a) \frac{1}{4}
(b) \frac{4}{1}
(c) \frac{8}{1}
(d) \frac{1}{8}.
Ans (d)
3.
The kinetic energy of the electron in an orbit of radius r in hydrogen atom is (e = electronic charge)
(a) \frac{{{{e}^{2}}}}{{{{r}^{2}}}}
(b) \frac{{{{e}^{2}}}}{{2r}}
(c) \frac{{{{e}^{2}}}}{r}
(d) \frac{{{{e}^{2}}}}{{2{{r}^{2}}}}.
Ans (b)
4.
A charged oil drop is suspended in a uniform electric field of 3 × 104 Vm–1, so that it neither falls nor rises. Find the charge on the drop if its mass is 9.75 × 10–15kg.
(a) 4.7 × 10–12 C
(b) 3.8 × 10–12 C
(c) 3.2 × 10–12 C
(d) 2.6 × 10–12 C
Ans (c)
5.
The angular momentum of an electron in a hydrogen atom is proportional to
(a) \frac{1}{{\sqrt{r}}}
(b) \frac{1}{r}
(c) \sqrt{r}
(d)r2.
Ans (c)
6.
One requires an energy En to remove a nucleon from a nucleus and an energy Ee to remove an electron from an atom. Then
(a) {{E}_{n}}={{E}_{e}}
(b) {{E}_{n}}<{{E}_{e}}
(c) {{E}_{n}}>{{E}_{e}}
(d) {{E}_{n}}\ge {{E}_{e}}.
Ans (c)
7.
Calculate the second excitation potential of single ionized helium.
Given e = 1.6×10–19 C, m = 9.1 10–31 kg and h = 6.62 10–34 J-s.
(a) 6.2 eV
(b) 5.4 eV
(c) 6.9 eV
(d) 7.7 eV
Ans (a)
8.
As per Bohr model, the minimum energy (in eV) required to remove an electron from the ground state of doubly ionized Li and (Z = 3) is
(a) 1.51
(b) 13.6
(c) 40.8
(d) 122.4
Ans (d)
9.
In Bohr’s model, the atomic radius of the first orbit is r0, then the radius of the third orbit is
(a) \frac{{{{r}_{0}}}}{9}
(b) {{r}_{0}}
(c) 9{{r}_{0}}
(d) 3{{r}_{0}}
Ans (c)
10.
As per Bohr model, the minimum energy (in eV) required to remove an electron from the ground state of doubly ionized Li and (Z = 3) is
(a) 1.51
(b) 13.6
(c) 40.8
(d) 122.4
Ans (d)
Practice Questions (Level-2)
1.
What is the impact parameter at which the scattering angle is 90° for Z = 79 and initial energy of the a-particle is 10 MeV?
(a)2.7 × 10–14m
(b) 0.7 × 10–14m
(c) 1.1 × 10–14m
(d) 1.8 × 10–14m
Ans (c)
2.
If the electron in H atom radiates a photon of wavelength 4860 Å, the kinetic energy of the electron
(a) decreases by 2.0 × 10–19J
(b) increases by 4.1 × 10–19J
(c) decreases by 4.1 × 10–19J
(d) increases by 8.2 × 10–19J.
Ans (c)
3.
A stream of electrons moving with a speed of 3 × 107 ms–1 is deflected on passing through an electric field of 1800 Vm–1, perpendicular to its path. If the radius of the deflected beam is 3 m, calculate the value of e/m.
(a) 1.7 × 1011 Ckg–1
(b) 0.5 × 1011 Ckg–1
(c) 2.4 × 1011 Ckg–1
(d) 3.7 × 1011 Ckg–1
Ans (a)
4.
An oil drop carrying a charge of 2e is kept stationary between two horizontal plates 2.0 cm apart when a potential difference of 12000V is applied across them. Calculate the radius of the drop. Density of oil 1200 kg m–3 and g = 9.8 ms–2.
(a) 1.2 × 10–6m
(b) 0.3 × 10–6m
(c) 2.8 × 10–6m
(d) 1.7 × 10–6m
Ans (d)
5.
The radius of Bohr’s first orbit is a0. The electron in nth orbit has a radius
(a)na0
(b) \frac{{{{a}_{0}}}}{n}
(c)n2a0
(d) \frac{{{{a}_{0}}}}{{{{n}^{2}}}}.–
Ans (c)
6.
The ratio of the speed of the electrons in the ground state of hydrogen to the speed of light in vacuum is
(a) \frac{1}{2}
(b) \frac{2}{{237}}
(c) \frac{1}{{237}}
(d) \frac{1}{{237}}
Ans (d)
7.
In terms of the Bohr’s radius a0 the radius of the second Bohr orbit of hydrogen atom is given by
(a)4 a0
(b)8 a0
(c) \sqrt{2}\,{{a}_{0}}
(d)2 a0.
Ans (a)
8.
Which of the following curves may represent the speed of electron in a hydrogen atom as a function of principal quantum number n?
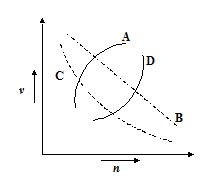
(a) A
(b) B
(c) C
(d) D
Ans (c)
9.
In hydrogen like atoms the ratio of difference of energies {{E}_{{4n}}}-{{E}_{{2n}}} and {{E}_{{2n}}}-{{E}_{n}} varies with atomic number z and principle quantum number n as
(a) \frac{{{{z}^{2}}}}{{{{n}^{2}}}}
(b) \frac{{{{z}^{4}}}}{{{{n}^{4}}}}
(c) \frac{z}{n}
(d) none of these
Ans (d)
10.
The radius of hydrogen atom in its ground state is 5.3 × 10–11 m. After collision with an electron it is found to have a radius of 21.2 × 10–11 m. What is the principal quantum number n of the final state of the atom?
(a) n = 4
(b) n = 2
(c) n = 16
(d) n = 3
Ans (b)
11.
An electron in H-atom jumps from second excited state to first excited state and then from first excited to ground state. Let the ratio of wavelength, momentum and energy of photons emitted in these two cases be a, b and c respectively. Then, choose the incorrect answer:
(a) c=\frac{1}{a}
(b) a=\frac{9}{4}
(c) b=\frac{5}{{27}}
(d) c=\frac{5}{{27}}
Ans (b)
12.
What is the ratio of the circumference of the first Bohr orbit for the electron in the hydrogen atom to the de-Broglie wavelength of electrons having the same velocity as the electron in the first Bohr orbit of the hydrogen atom?
(a) 1 : 1
(b) 1 : 2
(c) 1 : 4
(d) 2 : 1
Ans (a)
13.
In a hydrogen atom, an electron of mass m and charge e is in an orbit of radius r making n revolutions per second. If the mass of the hydrogen nucleus is M , the magnetic moment associated with the orbital motion of the electron is
(a) \frac{{\pi ne{{r}^{2}}m}}{M}
(b) \frac{{\pi ne{{r}^{2}}M}}{m}
(c) \frac{{\pi ne{{r}^{2}}m}}{{\left( {M+m} \right)}}
(d) \pi ne{{r}^{2}}
Ans (d)
14.
The hydrogen atoms in its ground state are excited by means of monochromatic radiations of wavelength 1023 Å. The number of lines which can be seen in the resulting spectrum is
(a) 1
(b) 2
(c) 3
(d) 6
Ans (c)
15.
In hydrogen atom, if potential energy of electron in ground state is assumed to be zero, then its energy in first excited state is equal to
(a) – 3.4 eV
(b) 23.8 eV
(c) 17.2 eV
(d) – 6.8 eV
Ans (b)
