Video Lecture
Theory For Making Notes
Electric Field
Electric Field is the space around a charge in which its influence (electric forces) can be experienced. In principle the electric field of a charge extends upto infinity.
In other words an electric charge influences its surroundings by creating an electric field. The second charge does not interact directly with the first, rather, it responds to whatever field it encounters.
Electric Field Intensity
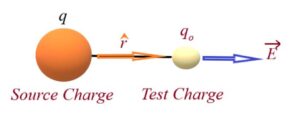
The electric field strength or intensity due to a point source charge q at any point P at a distance r from it is given by the force acting on a unit positive test charge placed at that point.
The electric field strength \vec{E} at a point is defined as the force \vec{F} per unit charge experienced by a vanishing small positive test charge q0
\displaystyle \vec{E}=\underset{{{{q}_{0}}\to 0}}{\mathop{{\lim }}}\,\frac{{\vec{F}}}{{{{q}_{0}}}}
\vec{E} is also called the electric field intensity or simply the electric field.
Note that \vec{E} at a point is equal to the force experienced by a unit positive charge placed at that point. Obviously, if \vec{E} is the electric field at any point, then the force experienced by a charge q at that point is
\vec{F}= q \vec{E}
SI unit of \displaystyle \vec{E} is Newton per coulomb (N/C), which is same as volt per metre (V/m):
unit of E\text{ }=\text{ }\frac{\text{N}}{\text{C}}\text{ }=\text{ }\frac{\text{J}}{\text{m}}\text{ }\times \text{ }\frac{\text{1}}{\text{C}}\text{ }=\text{ }\frac{\text{J}}{\text{C}}\text{ }\times \text{ }\frac{\text{1}}{\text{m}}\text{ }=\text{ }\frac{\text{V}}{\text{m}}
The dimensions of E are [MLT–3A–1].
The electric field created by a point charge q is given by
\displaystyle \vec{E} = \displaystyle \frac{{kq}}{{{{r}^{2}}}}\hat{r}
where the unit vector r has it origin at the source charge q as shown in the figure
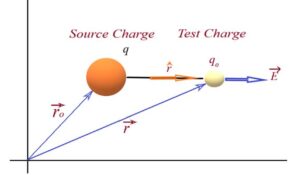
Vector form of electric field at position vector \displaystyle \vec{r} due to a point charge q placed at a point whose position vector is \displaystyle {{\vec{r}}_{0}} is given as \displaystyle \vec{E}=\frac{{kq\left( {\vec{r}-{{{\vec{r}}}_{0}}} \right)}}{{|\vec{r}-{{{\vec{r}}}_{0}}{{|}^{3}}}}
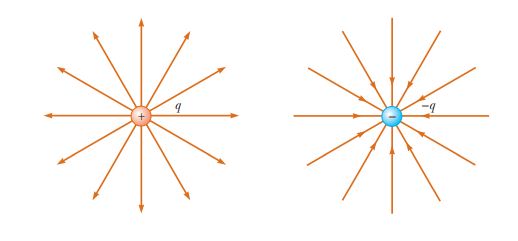
The electric field due to a charge points away from the charge if it is positive and towards it if the charge is negative as shown in the diagram given below

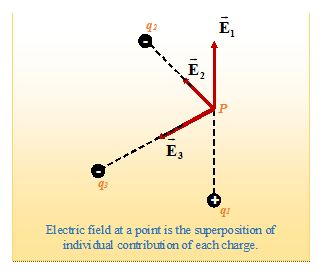
Principle of superposition
Since the principle of superposition is valid for Coulomb’s law, it is also valid for the electric field. To calculate the field strength at point due to a system of charges, we first find the individual field intensity {{\vec{E}}_{1}} due to q1, {{\vec{E}}_{2}} due to q2 and so on.
For N point charges, the resultant field intensity is the vector sum of all the individual electric fields due to different charges, so if \vec{E} is the net electric field then it is given by
\vec{E} = {{\vec{E}}_{1}} + {{\vec{E}}_{2}} + …….. + {{\vec{E}}_{N}}
Let the point charges \displaystyle {{q}_{1}},\,\,{{q}_{2}},\,\,{{q}_{3}},\,\,…… placed at the points whose position vectors are \displaystyle {{\vec{r}}_{1}},\,\,{{\vec{r}}_{2}},\,\,{{\vec{r}}_{3}},\,\,……. respectively. Then their net electric field at a point whose position vector \displaystyle \vec{r} is given as
\displaystyle \vec{E}\,\,=\,\frac{{k{{q}_{1}}\,\left( {\vec{r}-{{{\vec{r}}}_{1}}} \right)}}{{|\vec{r}-{{{\vec{r}}}_{1}}{{|}^{3}}}}\,+\,\frac{{k{{q}_{2}}\,\left( {\vec{r}-{{{\vec{r}}}_{2}}} \right)}}{{|\vec{r}-{{{\vec{r}}}_{2}}{{|}^{3}}}}\,+\,\frac{{k{{q}_{3}}\,\left( {\vec{r}-{{{\vec{r}}}_{3}}} \right)}}{{|\vec{r}-{{{\vec{r}}}_{3}}{{|}^{3}}}}+\,……..
hence \displaystyle \vec{E}\,\,=k\sum\limits_{{i=1}}^{{i=N}}{{\frac{{k{{q}_{i}}\,\left( {\vec{r}-{{{\vec{r}}}_{i}}} \right)}}{{|\vec{r}-{{{\vec{r}}}_{i}}{{|}^{3}}}}}}
this is also known as superposition principle.
Motion of a charged particle in an electric field
Let a particle of mass m, charge q be situated in an electric field of strength E, then force on the charged particle is given by \overset{\to }{\mathop{F}}\,=q.\overset{\to }{\mathop{E}}\,
The acceleration a produced by this force is \overset{\to }{\mathop{a}}\,=\frac{{\overset{\to }{\mathop{F}}\,}}{m}=\frac{{q\overset{\to }{\mathop{E}}\,}}{m} …(i)
If the particle is at rest at t = 0, then velocity of charged particle after time t is
\vec{v}=\vec{u}+\vec{a}t=0+\frac{{q\vec{E}}}{m}t
or \vec{v}=\frac{{q\vec{E}}}{m}t …(ii)
and distance traversed by the particle is \vec{s}=\vec{u}t+\frac{1}{2}\left( {\frac{{q\vec{E}}}{m}} \right){{t}^{2}}
or \vec{s}=\frac{1}{2}\left( {\frac{{q\vec{E}}}{m}} \right){{t}^{2}} …(iii)
and kinetic energy K =\frac{1}{2}m\left( {\frac{{qE}}{m}{{t}^{2}}} \right) =\frac{1}{2}m\frac{{{{q}^{2}}{{E}^{2}}}}{{{{m}^{2}}}}{{t}^{2}}
K=\frac{{{{q}^{2}}{{E}^{2}}}}{{2m}}{{t}^{2}} …(iv)
Note that the path followed by the particle depends upon the initial velocity of particle, its angle with the electric field and also type of electric field (means uniform or non uniform field)
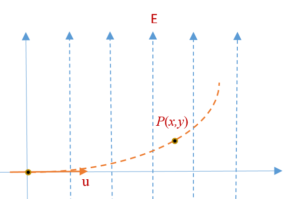
Motion of a charged particle when its initial velocity is perpendicular to a uniform electric field :
Consider a charged particle given an initial velocity along +ve x axis in a uniform electric field acting along +ve y axis.
The force on the particle is along Y-axis is given by {{\overset{\to }{\mathop{F}}\,}_{Y}}=q.\overset{\to }{\mathop{E}}\,
Acceleration of the particle along y–axis must be given by a=\frac{F}{m}
hence {{a}_{y}}= \frac{{F}_{y}}{m}=\frac{{qE}}{m}
Initial velocity is u along x – axis and it is zero along y–axis. The deflection (or displacement) after time t along y-axis can be found using S=ut+\frac{1}{2}a{{t}^{2}}
hence y=\frac{1}{2}\frac{{qE}}{m}{{t}^{2}} …(v)
As there is no acceleration along x-axis, therefore the displacement of particle in time t along x–axis is
x=ut …(vi)
Eleminating t from equation (v) and (vi), we get equation of trajectory as
y=\frac{1}{2}.\frac{{qE}}{m}{{\left( {\frac{x}{u}} \right)}^{2}}
\Rightarrow y\propto {{x}^{2}}
This shows that the path of the charged particle is a parabola.
Electric field due to a uniformly charged ring on its axis.
Let a total positive charge Q is distributed uniformly around a thin, circular, non-conducting ring of radius a. Let us calculate its electric field E at a point P along the axis of the ring, at a distance x from the centre, as shown in figure.
Consider a element charge dq on the ring and its electric field is written at the point P
As \displaystyle \vec{E}=\frac{{kdq}}{{{{r}^{2}}}}\hat{r} , where the magnitude \displaystyle dE = \frac{{k dq}}{{({{a}^{2}}+{{x}^{2}})}} . We can find its two components one along the x axis i.e. dEx the other perpendicular to x axis i.e. dE⊥
Note the symmetry. Every element dq can be paired with a similar element on the opposite side of the ring. Every component dE⊥ perpendicular to the x-axis is thus cancelled by an equal component dE⊥ in the opposite direction. In the summation process, all the perpendicular components dE⊥ add to zero. Thus, we are to add only the dE⊥ components.
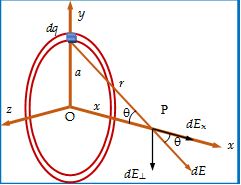
Its x-component is
\displaystyle d{{E}_{\text{x}}} = dE \text{cos} \theta = \frac{{k dq}}{{({{a}^{2}}+{{x}^{2}})}}.\frac{x}{{\sqrt{{{{a}^{2}}+{{x}^{2}}}}}}
E = Ex = \displaystyle \int{{d{{E}_{x}}}} = k\int{{\frac{{x dq}}{{{{{({{a}^{2}}+{{x}^{2}})}}^{{3/2}}}}}}}
As we integrate around the ring,
so \displaystyle \int{{dq}}=Q
Hence \displaystyle E = \frac{{k x Q}}{{{{{({{a}^{2}}+{{x}^{2}})}}^{{3/2}}}}}
Note that At centre x = 0 so Ecentre = 0
Whereas at a point on the axis such that x>>a. On neglecting a from the denominator we get E=\frac{{kQ}}{{{{x}^{2}}}}
NOTE
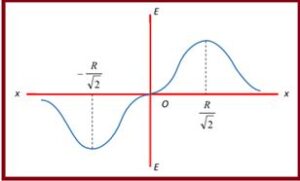
If we differentiate E with respect to x and putting dE/dx equal to zero we get x=\pm \frac{R}{{\sqrt{2}}}, It is the point where the electric field becomes maximum with magnitude {{E}_{{\max }}}=\frac{Q}{{6\sqrt{3}\pi {{\varepsilon }_{0}}{{a}^{2}}}}
Graph showing the variation of electric field of a ring with distance x
Illustration
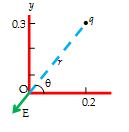
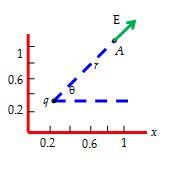
A 5.0µC point charge is placed at the point x = 0.2 cm, y = 0.3 cm. Find the magnitude of \displaystyle \overrightarrow{E} due to it (a) at the origin and (b) at point A with coordinates x = 1.0m, y = 1.0 m.
Solution
(a) The direction of \displaystyle \overrightarrow{E} at the origin is shown in figure
The distance of point O from the charge is
\displaystyle r=\sqrt{{{{{(0.2)}}^{2}}+{{{(0.3)}}^{2}}}}=0.36\text{m}
also \displaystyle \tan \theta =\frac{{0.3}}{{0.2}}=1.5
\Rightarrow \displaystyle \theta = {{tan}^{-1}}(1.5)={{56.3}^{o}}
The magnitude of electric intensity is
\displaystyle E=\frac{{kq}}{{{{r}^{2}}}}=\frac{{(9\times {{{10}}^{9}})\,\,(5\times {{{10}}^{{-6}}})}}{{{{{(0.36)}}^{2}}}}=3.46\times {{10}^{5}}\text{N}{{\text{C}}^{{-1}}}
(b) The distance between the charge q and the point A is
\displaystyle r=\sqrt{{{{{(1.0-0.2)}}^{2}}+{{{(1.0-0.3)}}^{2}}}}=1.06\text{m}
\displaystyle \tan \theta =\frac{{0.7}}{{0.8}}=\frac{7}{8}
\displaystyle \theta ={{\tan }^{{-1}}}\frac{7}{8}=41.2{}^\circ
The magnitude of E is
\displaystyle E=\frac{{kq}}{{{{r}^{2}}}}=\frac{{(9\times {{{10}}^{9}})\,(5\times {{{10}}^{{-6}}})}}{{{{{(1.06)}}^{2}}}}=4\times {{10}^{4}}\text{N}
Illustration
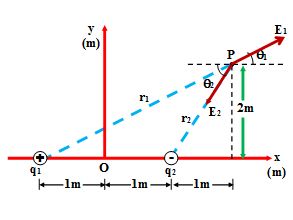
The figure shows a system of two charges. A point charge \displaystyle {{q}_{1}} = 20nC is at (-1,0) while \displaystyle {{q}_{2}} = -10nC is at (+1,0). Find the resultant field intensity at a point P with coordinates (2,2).

Solution
1.
The field vector \displaystyle {{E}_{1}} points away from the charge \displaystyle {{q}_{1}} while the vector \displaystyle {{E}_{2}} points toward the charge \displaystyle {{q}_{2}}.
2.
Let
The distance between P and \displaystyle {{q}_{1}}= \displaystyle {{r}_{1}}
and the distance between P and \displaystyle {{q}_{2}}= \displaystyle {{r}_{2}}
By distance formula
\displaystyle {{r}_{1}} = \sqrt{{{{{(2+1)}}^{2}}\,+{{2}^{2}}}}\,=\sqrt{{13}}=3.6\,m,
\displaystyle {{r}_{2}} = \sqrt{{{{1}^{2}}\,+{{2}^{2}}}}\,=\sqrt{5}\,=\,2.2\,m
The magnitude of the fields are
\displaystyle {{E}_{1}} = \frac{{k{{q}_{1}}}}{{r_{1}^{2}}}\,\,=\,\,\frac{{\left( {9\,\,\times \,{{{10}}^{9}}} \right)\,\,\left( {20\,\,\times \,{{{10}}^{{-9}}}} \right)}}{{{{{(3.6)}}^{2}}}} =\,13.8\,N{{C}^{{-1}}}
\displaystyle {{E}_{2}} = \displaystyle \frac{{k{{q}_{2}}}}{{r_{2}^{2}}}\,\,=\,\,\frac{{\left( {9\,\,\times \,{{{10}}^{9}}} \right)\,\,\left( {10\,\,\times \,{{{10}}^{{-9}}}} \right)}}{{{{{(2.2)}}^{2}}}} \displaystyle =\,18\,\,N{{C}^{{-1}}}
3.
The components of the resultant field are
\displaystyle {{E}_{x}}={{E}_{1}}\cos {{\theta }_{1}}-{{E}_{2}}\cos {{\theta }_{2}}
\displaystyle {{E}_{y}}={{E}_{1}}\cos {{\theta }_{1}}-{{E}_{2}}\cos {{\theta }_{2}}
were \displaystyle \cos {{\theta }_{1}} = \frac{3}{{3.6}} ; \displaystyle \cos {{\theta }_{2}}= \frac{1}{{2.2}}
and \displaystyle \sin {{\theta }_{1}} = \frac{2}{{3.6}} ; \displaystyle \sin {{\theta }_{2}} = \frac{2}{{2.2}}
Thus, Ex = (13.8) \frac{3}{{3.6}}\,-\,18\,\left( {\frac{1}{{2.2}}} \right)\,=\,3.3\,N/C
Ey = (13.8) \frac{2}{{3.6}} – 18 \left( {\frac{2}{{2.2}}} \right) = -8.7 NC–1
Finally, \displaystyle \overrightarrow{E}=3.3\hat{i}-8.7\hat{j}\,N{{C}^{{-1}}}
Illustration
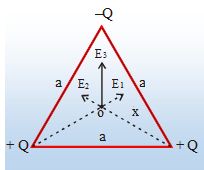
Three point charges are placed at the corners of an equilateral triangle as shown in figure. What is the value of electric field at the centre.
Solution
1st Method
By symmetry we find 2 observation
(1)
All {\overrightarrow{{E}_{1}}}, {\overrightarrow{{E}_{2}}}, {\overrightarrow{{E}_{3}}} have equal magnitude which is given by \frac {kq}{{{r}^{2}}} where r is the distance of point O from any charge and r = \frac{a}{\sqrt{3}}.
(2)
The x components of {\overrightarrow{{E}_{1}}}{ }and{ } {\overrightarrow{{E}_{2}}} being equal and opposite cancels with each other and hence the net electric field is given by the sum of y components of {\overrightarrow{{E}_{1}}}{ },{ } {\overrightarrow{{E}_{2}}} and {\overrightarrow{{E}_{3}}}.
Hence
{{E}_{net}}={{E}_{1}}sin\theta+{{E}_{2}}sin\theta+{{E}_{3}}
where \theta={{30}^{o}}
therefore {{E}_{net}}=\frac{Q}{{4\pi {{\in }_{0}}{{{\left( {\frac{a}{{\sqrt{3}}}} \right)}}^{2}}}}{ }sin{{30}^{o}} + \frac{Q}{{4\pi {{\in }_{0}}{{{\left( {\frac{a}{{\sqrt{3}}}} \right)}}^{2}}}}{ }sin{{30}^{o}} + \frac{Q}{{4\pi {{\in }_{0}}{{{\left( {\frac{a}{{\sqrt{3}}}} \right)}}^{2}}}}
so finally {{E}_{net}}= \frac{{6Q}}{{4\pi {{\in }_{0}}{{a}^{2}}}} along +ve y axis
2nd Method (By vectors)
Let us take origin at the centre and x and y-axis as shown in figure.
{\overrightarrow{{E}_{1}}}, {\overrightarrow{{E}_{2}}}, {\overrightarrow{{E}_{3}}} are the electric field due to +Q, +Q and – Q.
\displaystyle {{\vec{E}}_{1}}=\frac{Q}{{4\pi {{\in }_{0}}{{{\left( {\frac{a}{{\sqrt{3}}}} \right)}}^{20}}}}(\cos 30{}^\circ \hat{i}+\sin 30{}^\circ \hat{j})
\displaystyle {{\vec{E}}_{2}}=\frac{Q}{{4\pi {{\in }_{0}}{{{\left( {\frac{a}{{\sqrt{3}}}} \right)}}^{2}}}}(-\cos 30{}^\circ \hat{i}+\sin 30{}^\circ \hat{j})
\displaystyle {{\vec{E}}_{3}}=\frac{Q}{{4\pi {{\in }_{0}}{{{\left( {\frac{a}{{\sqrt{3}}}} \right)}}^{2}}}}(+\hat{j})
\displaystyle {{\vec{E}}_{{net}}}={{\vec{E}}_{1}}+{{\vec{E}}_{2}}+{{\vec{E}}_{3}}
\displaystyle =\frac{{6Q}}{{4\pi {{\in }_{0}}{{a}^{2}}}}\hat{j}
Practice Questions (Level-1)
Q.1
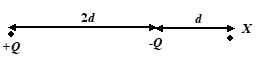
Figure shows a charge +Q a distance 2d from a charge –Q and a point X distance d from –Q. The field strength at X is numerically, using SI units
(a) \frac{Q}{{4\pi {{\varepsilon }_{o}}{{d}^{2}}}}
(b) \frac{Q}{{36\pi {{\varepsilon }_{o}}{{d}^{2}}}}
(c) \frac{{3Q}}{{4\pi {{\varepsilon }_{o}}{{d}^{2}}}}
(d) \frac{{2Q}}{{9\pi {{\varepsilon }_{o}}{{d}^{2}}}}
Ans .(d)
Q.2
A positively charged pendulum is oscillating in a uniform electric field as shown in the figure. Its time period as compared to that when it was uncharged
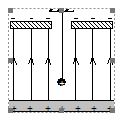
(a) will increase
(b) will decrease
(c) will not change
(d) will first increase then decrease
Ans. (a)
Q.3
An oil droplet of density \rho and radius r carries a charge q when placed in a electric field E, its moves upwards with a velocity \nu .If \rho is the density of air, \eta be the viscosity of the air, then which of the following forces is directed upwards ?
(a) 6 \pi \rho \eta r
(b) q E
(c) \frac{4}{3}\pi {{r}^{3}}(\rho -{{\rho }_{0}})g
(d) qE + 6 \pi \rho \eta \nu
Ans. (b)
Q.4
The diagram shows electric lines of force. If EA and EB are electric fields at A and B and distance AB = r, then
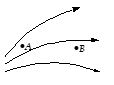
(a) \displaystyle {{E}_{A}}>{{E}_{B}}
(b) \displaystyle {{E}_{A}}={{E}_{B}}/r
(c) \displaystyle {{E}_{A}}<{{E}_{B}}
(d \displaystyle {{E}_{B}}={{E}_{A}}/r
Ans (a)
Q.5
Charges 2Q and –Q are placed as shown. The point at which electric field intensity is zero will be
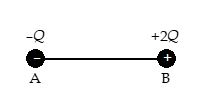
(a) somewhere between –Q and 2Q
(b) somewhere on the left of –Q
(c) somewhere on the right of 2Q
(d) somewhere on the right bisector of line joining –Q and 2Q
Ans. (b)
Q.6
Five point charges (+q each) are placed at the five vertices of a regular hexagon of side 2a. What is the magnitude of the net electric field at the centre of the hexagon?
(a) \frac{1}{{4\pi {{\varepsilon }_{o}}}}\frac{q}{{{{a}^{2}}}}
(b) \frac{q}{{16\pi {{\varepsilon }_{o}}{{a}^{2}}}}
(c) \frac{{\sqrt{2}\,q}}{{4\pi {{\varepsilon }_{o}}{{a}^{2}}}}
(d) \frac{{5q}}{{16\pi {{\varepsilon }_{o}}{{a}^{2}}}}
Ans . (b)
Q. 7
In a regular polygon of n sides, each corner is at a distance r from the centre. Identical charges of magnitude Q are placed at (n-1) corners. The field at the centre is
(a) k \frac{Q}{{{{r}^{2}}}}
(b) (n-1)k \frac{Q}{{{{r}^{2}}}}
(c) \frac{n}{{n-1}} k \frac{Q}{{{{r}^{2}}}}
(d) \frac{{n-1}}{n} k \frac{Q}{{{{r}^{2}}}}
Ans . (a)
Q.8
Charge Q is given a displacement aî+bĵ in an electric field \displaystyle {{E}_{1}}\hat{i}+{{E}_{2}}\hat{j}. The work done is
(a) \displaystyle Q\left( {{{E}_{1}}a+{{E}_{2}}b} \right)
(b) Q \sqrt{{(2{{E}_{1}}a)+(3{{E}_{2}}b)}}
(c) Q\left( {{{E}_{1}}+{{E}_{2}}} \right) \sqrt{{{{a}^{2}}+{{b}^{2}}}}
(d) Q \displaystyle \sqrt{{(2{{E}_{1}}b+{{E}_{2}}a)}}
Ans .(a)
Q.9
Which of the following is not true for a region with a uniform electric field?
(a) It can have free charges
(b) It may have uniformly distributed charge.
(c) It may contain dipoles.
(d) None of the above.
Ans. (d)
Q.10
A simple pendulum of time period T is suspended above a large horizontal metal sheet with uniformly distributed positive charge. If the bob is given some negative charge, its time period of oscillation will be
(a) >T
(b) <T
(c) T
(d) proportional to its amplitude.
Ans. (b)
Q. 11
The maximum electric field intensity on the axis of a uniformly charged ring of charge q and radius R will be :
(a) \displaystyle \frac{1}{{4\pi {{\varepsilon }_{0}}}}\,\,\frac{q}{{3\sqrt{3}{{R}^{2}}}}
(b) \displaystyle \frac{1}{{4\pi {{\varepsilon }_{0}}}}\,\,\frac{{2q}}{{3{{R}^{2}}}}
(c) \displaystyle \frac{1}{{4\pi {{\varepsilon }_{0}}}}\,\,\frac{{2q}}{{3\sqrt{3}{{R}^{2}}}}
(d) \displaystyle \frac{1}{{4\pi {{\varepsilon }_{0}}}}\,\,\frac{{3q}}{{2\sqrt{2}{{R}^{2}}}}
Ans. (c)
Q.12
Two particles A and B (B is right of A) having charges and , respectively, are held fixed with separation of 20 cm. Where should a third charged particle be placed so that it does not experience a net electric force.
(a) 5cm right of B
(b) 5cm left of A
(c) 20cm left of A
(d) 20cm right of B
Ans. (d)
Q.13
Two particles of masses in the ratio 1 : 2, with charges in the ratio 1 : 1, are placed at rest in a uniform electric field. They are released and allowed to move for the same time. The ratio of their kinetic energies will be finally
(a) 2 : 1
(b) 8 : 1
(c) 4 : 1
(d) 1 : 4
Ans. (a)
Q.14
An electron of mass {{m}_{e}}, initially at rest, moves through a certain distance under a uniform electric field in time {{t}_{e}}. A proton of mass {{m}_{p}} also initially at rest, takes time {{t}_{p}} to move through the same distance in the same electric field. Neglecting the effect of gravity, the ratio \frac {{t}_{e}}{{t}_{p}} is nearly equal to
(a) 1
(b) \displaystyle \sqrt{{{{m}_{p}}/{{m}_{e}}}}
(c) \displaystyle \sqrt{{{{m}_{e}}/{{m}_{p}}}}
(d) 1836
Ans. (c)
15.
Two point charges +8q and -2q are located at x = 0 and x = L respectively. The location of a point on the x-axis at which the net electric field due to these two point charges is zero, is
(a) 2 L
(b) \frac{L}{4}
(c) 8 L
(d) 4 L
Ans (a)
Practice Questions (Level-2)
Q.1
At what distance ‘x’ from the centre the intensity of electric field due to a ring of radius R and charge Q on its axis is maximum
(a) x = R
(b) x = R/2
(c) x = \sqrt{2}R
(d) x = \frac{R}{{\sqrt{2}}}
Ans. (d)
Q.2
A spring-block system undergoes vertical oscillation above a large horizontal metal sheet with uniform positive charge. The time period of the oscillation is T. If the block is given a charge Q, its time period of oscillation will be
(a) T
(b) more than T
(c) T if Q is positive
(d) less than T
Ans. (a)
Q.3
A large flat metal surface has a uniform charge density + \displaystyle \sigma . An electron of mass m and charge e leaves the surface at point A with speed u, and returns to it at point B. Disregard gravity. The maximum value of AB is
(a) \frac{{{{u}^{2}}m{{\varepsilon}_{0}}}}{{\sigma e}}
(b) \frac{{{{u}^{2}}e {{\varepsilon}_{0}}}}{{m\sigma }}
(c) \frac{{{{u}^{2}}e}}{{{{\varepsilon}_{0}}\sigma m}}
(d) \frac{{{{u}^{2}} \sigma e}}{{{{\varepsilon}_{0}}m}}
Ans. (a)
Q.4
An extremely long wire is uniformly charged. An electron is revolving about the wire and making 108 revolutions per second in an orbit of radius 2cm. Linear charge density of the wire is nearly :
(a) 50 nC
(b) 25 nC
(c) 62.5 nC
(d) 12.5 nC
Ans. (a)
Q.5
Four identical charges Q are fixed at the four corners of a square of side a. The electric field at a point P located symmetrically at a distance x from the center of the square is
(a) \displaystyle \frac{{2\sqrt{2}qx}}{{\pi {{\varepsilon }_{o}}{{{({{a}^{2}}+2{{x}^{2}})}}^{{3/2}}}}}
(b) \displaystyle \frac{Q}{{\sqrt{2}\pi {{\varepsilon }_{0}}({{a}^{2}+{{x}^{2}})}}}
(c) \displaystyle \frac{{2\sqrt{2}qx}}{{\pi {{\varepsilon }_{o}}{{{({2{a}^{2}}+{{x}^{2}})}}^{{3/2}}}}}
(d) \displaystyle \frac{{\sqrt{2}Q}}{{\pi {{\varepsilon }_{0}}{{a}^{2}}}}
Ans. (a)
Q.6
One of the two parallel metallic plates is uniformly charged with charge +q and the other one is charged with charge –q. In this case, the electric field between them is E. When the negatively charged plate is discharged then recharged with a positive charge 4q, the magnitude of electric field between the plates becomes
(a) 1.5 E
(b) 2.5 E
(c) 3 E
(d) 5 E
Ans..(a)
Q.7
Consider three identical metal spheres A(0,0), B(1,0) and C(2,0). Spheres A carries charge + 6q and sphere B carries charge – 2q Sphere C carries no charge. IF firstly spheres A and B are touched together through a wire and then A and C are touched through a wire . Choose the wrong statement
(a) Finally the force acting on B must be zero
(b) The final charge on A must be q
(c) The electric field at c must be zero
(d) The final elecric field at (1,2) must be non zero.
Ans. (c)
Q.8
Two positive point charges of magnitude Q each are separated by a distance “2a”. A test charge is located in a plane which is normal to the line joining these charges and midway between them. The locus of the points in this plane for which the force on the test charge has a maximum value is
(a) a circle of radius r=a/\sqrt{2}
(b) a circle of radius r=a
(c) a straight line
(d) an ellipse
Ans. (a)
Q.9
A particle of mass m and charge q is placed at rest in a uniform electric field E as shown and released. The kinetic energy it attains after moving a distance y is

(a) \frac{1}{2}qEy
(b) qEy
(c) qE2y
(d) \frac{1}{2}m(qEy)
Ans. (b)
Q.10
The electric field on two sides of a charged plate is shown in the figure. The charge density on the plate is given by
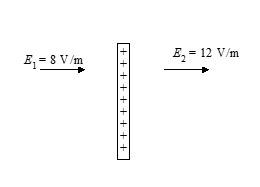
(a) 2 \displaystyle {{\varepsilon }_{o}}
(b) 4 \displaystyle {{\varepsilon }_{o}}
(c) 10 \displaystyle {{\varepsilon }_{o}}
(d) zero
Ans. (b)
Q.11
A thin glass rod is bent into a semicircle of radius R. A charge +Q is uniformly distributed along the upper half, and a charge –Q is uniformly distributed along the lower half. The electric field E at P, the center of the semicircle, is
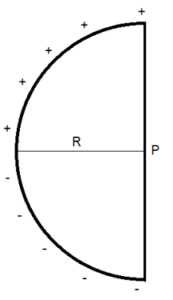
(a) \displaystyle \frac{Q}{{{{\pi }^{2}}{{\varepsilon }_{0}}{{r}^{2}}}}
(b) \displaystyle \frac{{2Q}}{{{{\pi }^{2}}{{\varepsilon }_{0}}{{r}^{2}}}}
(c) \displaystyle \frac{{4Q}}{{{{\pi }^{2}}{{\varepsilon }_{0}}{{r}^{2}}}}
(d) \displaystyle \frac{Q}{{4{{\pi }^{2}}{{\varepsilon }_{0}}{{r}^{2}}}}
Ans. (a)
Q.12
A block of mass m having charge q, is hinged by a spring of spring constant k in a vertical electrostatic field E. The spring extension in equilibrium will be
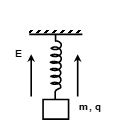
(a)\displaystyle\frac{{mg}}{k}
(b) \displaystyle \frac{{qE}}{k}
(c) \displaystyle \frac{{mg+qE}}{k}
(d) \displaystyle \frac{{mg-qE}}{k}
Ans : (d)
Q. 13
Two identical point charges are placed at a separation of l. P is a point on the line joining the charges, at a distance x from any one charge. The field at P is E. E is plotted against x for values of x from close to zero to slightly less than l. Which of the following best represents the resulting curve?
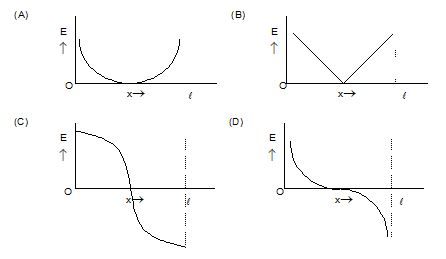
Ans. (d)
Q.14
Figure shows a uniformly charged hemispherical shell. The direction of electric field at point p, that is off-centre (but in the plane of the largest circle of the hemisphere), will be along
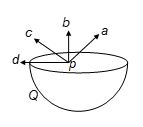
(a) pa
(b) pb
(c) pc
(d) pd
Ans. (b)
Q.15
Three charges + 2Q, – 3Q and –Q are arranged in a line as shown in the figure. The number of points along the line at which the net electric field is zero is
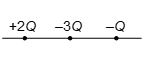
(a) 1
(b) 2
(c) 3
(d) More than 3
Ans. (b)
