Video Lecture
Measuring Instruments
Galvanometer
A galvanometer detects the presence of current in the branch where it is connected.
It is the basic instrumentused in making various meters, such
(i)
ammeter (measures current)
(ii)
voltmeter(measures voltage or potential difference)
(iii)
ohmeters(measures resistance)
(iv)
powermeter (measures power)
A galvanometer can detect a current as low as 10–9 A.
- In DC circuits, usually moving coiltype galvanometers are used. Its deflection is directly proportional to the current that passes through it, or
I∝ q or I = Kθ
where K is called galvanometer constant.
- The deflection per unit current is called current sensitivity of the galvanometer,
CS=\frac{\theta }{I}=\frac{1}{K}
Shunting a galvanometer decreases its sensitivity. (When a small resistance is connected in parallel with a large resistance, we say that the large resistance is shunted).
- The total resistance of the galvanometer between its two terminals is called galvanometer resistance and is represented by G.
- The total resistance of the galvanometer between its two terminals is called galvanometer resistance and is represented by G.
- The current required for full-scale deflection in a galvanometer is called full-scale deflection current and is represented by Ig.
Ammeter
An ammeter is an instrument which reads the current passing through it. The ammeter must be inserted into the branch so that the current to be measured passes through it. That is, an ammeter is connected in series with the element through which current is to measured.
- The reading of an ammeter is always lesser than the actual current in the circuit. If V is the potential difference across a resistance R, the true current is I = (V/R). However, when an ammeter of resistance r is used to measure it, the reading will be
{I}’=\frac{V}{{(R+r)}}
which is less than the true current I.
- Smaller the resistance of an ammeter, the more accurate will be its reading. An ammeter is said to be ideal if its resistance (r) is zero. However, ideal ammeter cannot be realized in practice.
Conversion of a Galvanometer into an Ammeter
To convert a galvanometer into an ammeter of a certain range, say Io, a small resistance S (called shunt) is connected in parallel with the galvanometer. The range means the upper limit of the quantity which can be measured by the instrument. The value of S is chosen such that the current passing through the galvanometer of resistance G becomes equal to its full-scale deflection value Ig. Thus, equating the potential difference across two parallel branches, we have
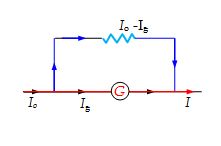
IgG = (Io – Ig)S
or S=\frac{{{{I}_{g}}}}{{\text{(}{{I}_{o}}\text{ }-\text{ }{{I}_{g}})}}G
Illustration
What is the value of shunt which passes 10% of the main current through a galvanometer of 99 ohms.
Solution
As the shunt is a small resistance S in parallel with a galvanometer (of resistance G)
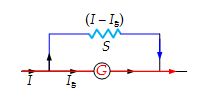
(I – Ig)S = Ig*G
or \displaystyle S=\frac{{{{I}_{g}}G}}{{(I-{{I}_{g}})}}
Here, G = 99 Ω and
Ig = (10/100) I = 0.1I
\displaystyle S=\frac{{0.1I\times 99}}{{(I-0.1\,I)}}=\frac{{0.1}}{{0.9}}\times 99 = 11 Ω
Voltmeter
A voltmeter is an instrument which reads the potential difference across its terminals. To measure the potential difference between any two points A and B in the circuit, the voltmeter terminals are connected to A and B without breaking the circuit.
- The reading of a voltmeter is always lesser than the true value. If a current Io is passing through a resistance R, the true value V = IoR. However, when a voltmeter having resistance r is connected across R, the current through R will become
{I}’=\frac{r}{{(R+r)}}{{I}_{o}}
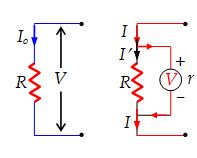
and so {V}’={I}’R=\frac{V}{{[1+(R/r)]}}
When the voltmeter is connected across R, its reading will also be V’ which is less than V.
- Greater the resistance of voltmeter, the more accurate will be its reading. A voltmeter is said to be idealif its resistance r is infinite. An ideal voltmeter draws no current from the circuit element for its operation.
Conversion of a Galvanometer into a Voltmeter
To convert a galvanometer into a voltmeter of certain range, say V, a high resistance R is connected in series with the galvanometer. The value of R is chosen such that the current passing through the galvanometer of resistance G becomes equal to its full-scale deflection value Ig. Thus, we should have
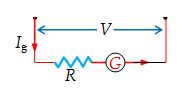
V = Ig(G + R)
or R=\frac{V}{{{{I}_{g}}}}-\text{ }G
Illustration
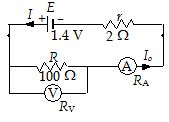
A battery of emf 1.4 V and internal resistance 2 Ω is connected to a resistor of 100 Ω. In order to measure the current through the resistance and the potential difference across its ends, an ammeter is connected in series with it and a voltmeter is connected across its ends. The resistance of the ammeter is 4/3 Ω and that of the voltmeter is 200 Ω. What are the readings of the two instruments ? What would be their reading if they were ideal instruments ?
Solution
Let RA and RV be the resistances of the ammeter and voltmeter respectively. Then the total resistance across the emf E is
Req = \displaystyle \frac{{R{{R}_{V}}}}{{R+{{R}_{V}}}}+{{R}_{A}}+r=\frac{{100\times 200}}{{100+200}}+\frac{4}{3}+2 = \displaystyle \frac{{210}}{3}Ω
Therefore, the current
{{I}_{o}}=\frac{E}{{{{R}_{{eq}}}}}=\frac{{1.4}}{{(210/3)}}= 0.02 A
This is the current through ammeter. Hence, the reading of ammeter is 0.02 A.
Reading of voltmeter = pd across its terminals
= {{I}_{o}}\left( {\frac{{R{{R}_{V}}}}{{R+{{R}_{V}}}}} \right)=0.02\left( {\frac{{100\times 200}}{{100+200}}} \right)= 1.33 V
If the ammeter and the voltmeter were ideal, RA = 0 and RV= ¥. Then,
The reading of ammeter = \displaystyle \frac{E}{{r+R}}=\frac{{1.4}}{{2+100}}= 0.0137 A
The reading of voltmeter = {{I}_{o}}R=\frac{{1.4}}{{102}}\times 100 = 1.37 V
Wheat-stone Bridge
It is an arrangement of four resistances used for measuring unknown resistance in terms of other three known resistances.
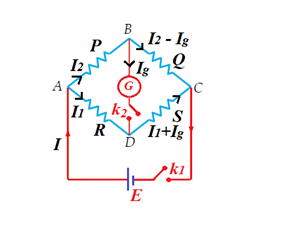
Construction
It consists of four resistances P, Q, R and S arranged in form of bridge. A source of emf E is connected between A and C and galvanometer is connected between B and D. Usually unknown resistance is put in place S.
Principle:
If there is no deflection in galvanometer on pressing key {{K}_{1}} and {{K}_{2}} , then Wheatstone Bridge is said to be balanced and ratio of P and Q is equal to the ratio of R and S
i.e., \frac{P}{Q}=\frac{R}{S}
Knowing, P, Q and R, the value of S can be calculated.
Proof:
Applying K.V.L in loop BDCB, we get
{{I}_{1}}.R-{{I}_{g}}G-{{I}_{2}}P=0 … (i)
Applying K.V.L in loop ADBA, we get
{{I}_{g}}G+({{I}_{1}}+{{I}_{g}})S-({{I}_{2}}-{{I}_{g}})Q=0 … (ii)
If bridge is balanced, then {{I}_{g}}=0
Hence, equation (i) and (ii) becomes
{{I}_{1}}.R={{I}_{2}}.P
and {{I}_{1}}.S={{I}_{2}}.Q
or \frac{P}{Q}=\frac{R}{S}
When this happens, the points B and D are at the same potential.
Also note that in the network, the cell arm and the galvanometer arm are interchangeable.
Illustration
An electrical circuit is shown in the figure. Calculate the potential difference across the resistor of 400 ohm, as will be measured by the voltmeter of resistance 400 ohm, either by applying Kirchhoff’s rules or otherwise.
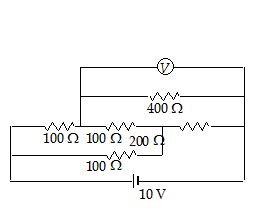
Solution
The equivalent resistance of the voltmeter and the 400Ω resistor in parallel is 200Ω . The circuit is a balanced Wheatstone bridge.
No current will flow through the 100 Ω-resistor connected between B and D.
Meter bridge: (Slide wire meter bridge)
It is used to measure unknown resistance and to compare unknown resistance of two conductors.
Principle:
It works on the principle of balanced wheatstone bridge i.e., \frac{P}{Q}=\frac{R}{S}
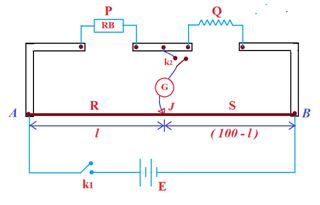
Working:
Close key {{k}_{1}} and then {{k}_{2}} , now move up Jockey (J) over the wire AB so that galvanometer shows no deflection.
If r is resistance per unit length of wire then resistance of portion l is R=l\,.\,r and resistance of portion (100-l) is S=(100-l)\,r . Therefore
\frac{R}{S}=\frac{l}{{(100-l)}} … (i)
and according to principle of wheatstone bridge
Hence, \frac{P}{Q}=\frac{R}{S}
or \frac{P}{Q}=\frac{l}{{(100-l)}} …(ii)
Hence Q=\frac{{(100-l)}}{l}\times P
If Q is nearly equal to P, balance point of the bridge will be near to middle of slide wire.
Illustration
Two unknown resistances X and Y are placed in the left and right gaps of a metre bridge. The null point in galvanometer is obtained at a distance of 80 cm from left. A resistance of 100 Ω is now connected in parallel across X. The null point is then found by shifting the sliding contact towards left by 20 cm. Calculate X and Y.
Solution
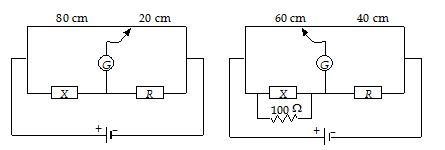
From first null point,
\displaystyle \frac{X}{Y}=\frac{{80}}{{20}} … (i)
From second null point,
\displaystyle \frac{{\left( {\frac{{100 X}}{{100+X}}} \right)}}{Y}=\frac{{60}}{{40}} …(ii)
From Eqn. (i) and (ii), we have
\displaystyle X=\frac{{500}}{3}\Omega \text{ }and\text{ }Y=\frac{{125}}{3}\Omega
