Lorem ipsum dolor sit amet, consectetur adipiscing elit. Ut elit tellus, luctus nec ullamcorper mattis, pulvinar dapibus leo.
Lorem ipsum dolor sit amet, consectetur adipiscing elit. Ut elit tellus, luctus nec ullamcorper mattis, pulvinar dapibus leo.
Q.1
Calculate net resistance between A and B :
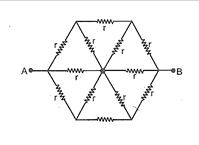
(a) \displaystyle \frac{{4r}}{5}
(b) \displaystyle \frac{{3r}}{5}
(c) \displaystyle \frac{{4r}}{3}
(d) None
Ans. (a)
Q.2
Calculate net resistance between A and B :
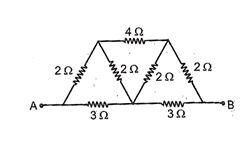
(a) 1 \displaystyle \Omega
(b) 2 \displaystyle \Omega
(c) 3 \displaystyle \Omega
(d) None
Ans. (c)
Q.3
Calculate net resistance between A and B :
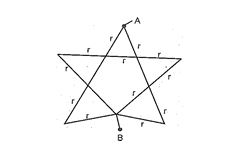
(a) \displaystyle \frac{{7r}}{3}
(b) \displaystyle \frac{{5r}}{6}
(c) \displaystyle \frac{{7r}}{2}
(d) \displaystyle \frac{{7r}}{6}
Ans. (d)
Q.4
A five-point regular star has been formed from a uniform wire. Calculate the equivalent resistance between points P and Q. Take sin =1/3 resistance of sections \displaystyle PQ\,=\,QR\,=\,RS\,=\,ST\,=\,TU\,=\,UV\,=\,VW\,=\,WX\,=\,XY\,=\,r
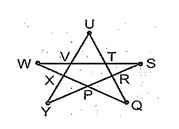
(a) r
(b) 2r/3
(c) 3r/5
(d) 2r
Ans. (c)
Q.5
Twelve wires of equal resistance R are connected to form a cube. The effective resistance between the diagonal ends of the figure will be :
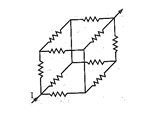
(a) (5/6)R
(b) (6/5) R
(c) 3R
(d) 12R
Ans. (a)
Q.6
Net resistance between A and B in the given network is :
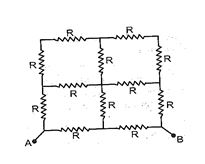
(a) \displaystyle \frac{{5R}}{7}
(b) \displaystyle \frac{{7R}}{6}
(c) \displaystyle \frac{{4R}}{5}
(d) \displaystyle \frac{{5R}}{4}
Ans. (d)
Q.7
Net resistance between A and B in the given network is :
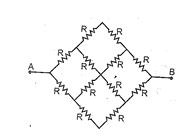
(a) \displaystyle \frac{{5R}}{7}
(b) \displaystyle \frac{{7R}}{6}
(c) \displaystyle \frac{{3R}}{2}
(d) \displaystyle \frac{{5R}}{4}
Ans. (c)
Q.8
The current I drawn from the 5 volt source will be :
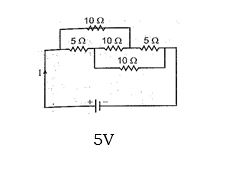
(a) 0.17 A
(b) 0.33 A
(c) 0.5 A
(d) 0.67 A
Ans.. (c)
Q.9
The given figure shows an infinite ladder network of resistances. The equivalent resistance between points A and B is :
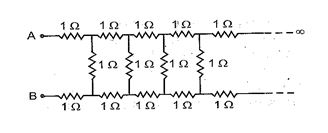
(a) Infinite
(b) 3.73 \displaystyle \Omega
(c) 2.73 \displaystyle \Omega
(d) 2/3 \displaystyle \Omega
Ans. (c)
Q.10
Find RAB, each individual resistance being R.
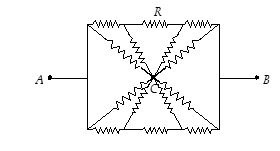
(a) 4R/5
(b) 7R/4
(c) 3R/2
(d) 5R/2
Ans. (a)
Q.11
Find RAB, each individual resistance being R.
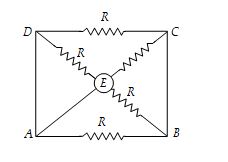
(a) R/5
(b) R/4
(c) R/2
(d) 5R/2
Ans. (b)
Q.1
Find the net resistance in each of the following symmetrical circuits
(Each edge of the cubes given in (b), (c),(d) and (e) has a resistance `r’ )
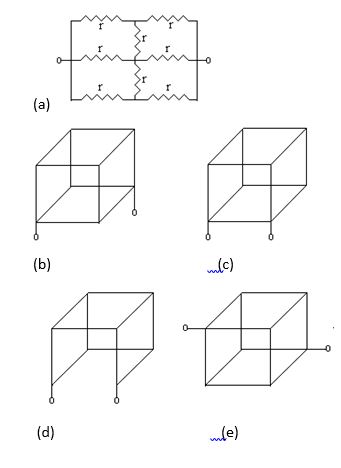
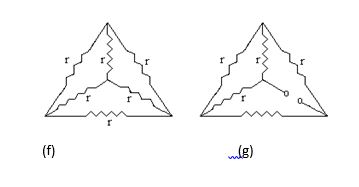
Q.2
A finite square grid each link having resistance r is fitted in a resistanceless conducting circular wire. Determine equivalent resistance between A and B.
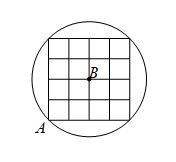
Ans : (21r)/40
Q.3
In the diagram shown, all the wires have resistance R. The equivalent resistance between the upper and lower dots shown in the diagram is
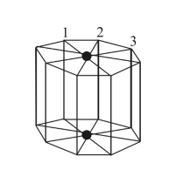
(a) R/8
(b) R
(c) 2R/5
(d) 3R/8
Ans. (d)
Q.4
In the infinite square grid resistance of each link is r. Determine the equivalent resistance between two junctions A and B. The link between A and B is missing.
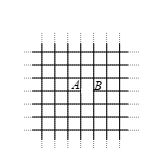
Ans. x = r
Get Full Access Of the Chapters
