Video Lecture
Theory For Notes Making
Gas
Gas is that state of matter in which the intermolecular forces are very weak and its molecule may fly apart in all directions. So the gas has no shape and size and can be contained in a vessel of any shape or size. It can expand indefinitely and uniformly to fill the available space. The gases can exert pressure on its surroundings.
Ideal Gas Equation
The equation which relates the pressure (P) volume (V) and temperature (T) of the given state of an ideal gas is known as ideal gas equation or equation of state.
For 1 mole of gas \frac{{PV}}{T}=R (constant) PV = RT
For n moles of gas the equation of gas becomes PV=nRT.
whereR = universal gas constant withDimension [M{{L}^{2}}{{T}^{{-2}}}{{\theta }^{{-1}}}]
Universal gas constant signifies the work done by (or on) a gas per mole per kelvin.
R=\frac{{PV}}{{nT}}=\frac{{\text{Pressure }\times \text{Volume}}}{{n\times \text{ Temperature}}} =\frac{{\text{Work done}}}{{\text{n }\times \text{ Temperature}}}
At S.T.P. the value of universal gas constant is same for all gases
R = 8.31\frac{J}{{mole\times kelvin}}=1.98\frac{{cal}}{{mole\times kelvin}} \tilde{-}\,2\frac{{cal}}{{mol\,\times kelvin}}
=0.8221\frac{{\,litre\times atm}}{{mole\times kelvin}}.
Real Gases
The gases actually found in nature are called real gases. They do not obeys gas Laws. For exactly one mole of an ideal gas \frac{{PV}}{{RT}}=1. Plotting the experimentally determined value of \frac{{PV}}{{RT}} for exactly one mole of various real gases as a function of pressure P, shows a deviation from identity.
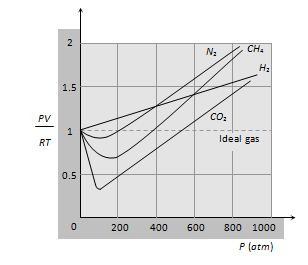
A real gas behaves as ideal gas most closely at low pressure and high temperature. Equation of state for real gaseswas given by Vander Waal’s with two correction in ideal gas equation. The itknow as Vander Waal’s gas equation.
(i) Volume correction :Due to finite size of molecule, a certain portion of volume of a gas is covered by the molecules themselves. Therefore the space available for the free motion of molecules of gas will be slightly less than the volume V of a gas. Hence the effective volume becomes (V – b).
(ii) Pressure correction : Due to intermolecular force in real gases, molecule do not exert that force on the wall which they would have exerted in the absence of intermolecular force. Therefore the observed pressure P of the gas will be less than that present in the absence of intermolecular force. Hence the effective pressure becomes \left( {P+\frac{a}{{{{V}^{2}}}}} \right).
Vander Waal’s gas equations
For 1 mole of gas \left( {P+\frac{a}{{{{V}^{2}}}}} \right)\,(V-b)=RT
For n moles of gas \left( {P+\frac{{a{{n}^{2}}}}{{{{V}^{2}}}}} \right)\ (V-nb)=n\,RT
Here a andb are constant called Vander Waal’s constant.
Dimension : [a] = [M{{L}^{5}}{{T}^{{-2}}}] and [b] = [L3]
Units :a = N x m4 and b = m3.
Assumption of Ideal Gases (or Kinetic Theory of Gases)
Kinetic theory of gases (KTG) relates the macroscopic properties of gases (such as pressure, temperature etc.) to the microscopic properties of the gas molecules (such as speed, momentum, kinetic energy of molecule etc.)
Actually in KTG a model of the molecular behavior was developed, which explains the behavior of an ideal gas. In this model few assumptions were made which are given below :
(1)
Every gas consists of extremely small particles known as molecules. The molecules of a given gas are all identical but are different than those of another gas.
(2)
The molecules of a gas are identical, spherical, rigid and perfectly elastic point masses.
(3)
Their size is negligible in comparison to intermolecular distance (10–9m)
(4)
The volume of molecules is negligible in comparison to the volume of gas. (The volume of molecules is only 0.014% of the volume of the gas).
(5)
Molecules of a gas keep on moving randomly in all possible direction with all possible velocities.
(6)
The speed of gas molecules lie between zero and infinity
(7)
The gas molecules keep on colliding among themselves as well as with the walls of containing vessel. These collisions are perfectly elastic.
(8)
The time spent in a collision between two molecules is negligible in comparison to time between two successive collisions.
(9)
No attractive or repulsive force acts between gas molecules. Hence there is no potential energy stored in the molecules.
(10)
Gravitational attraction among the molecules is ineffective due to extremely small masses and very high speed of molecules.
(11)
Molecules constantly collide with the walls of container due to which their momentum changes. The change in momentum is transferred to the walls of the container. Consequently pressure is exerted by gas molecules on the walls of container.
(12)
The density of gas is constant at all points of the container.
Calculation of pressure of an ideal gas
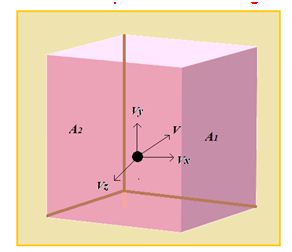
Consider an ideal gas enclosed in a cubical vessel of edge L as shown in figure also consider a molecule having velocity \displaystyle \vec{v} with components along the axes as \displaystyle {{v}_{x}},\,\,\,{{v}_{y}} and \displaystyle {{v}_{z}}.
When the molecule collides with face \displaystyle {{A}_{1}} , the x component of velocity is reversed whereas the y and z components remain unchanged.
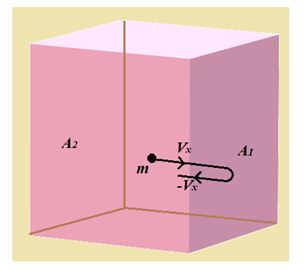
This follows from our assumption that the collision between molecule and wall is elastic.
The magnitude of the change in momentum of the molecule of mass ‘m’ after collision with the wall is therefore.
\displaystyle \Delta p\,=\,m{{v}_{x}}-\left( {-m{{v}_{x}}} \right)=2\,m{{v}_{x}}
Where, m is the mass of the molecule.
After rebounding from face \displaystyle {{A}_{1}}, the molecule travels towards face \displaystyle {{A}_{2}} and now gets rebound from face \displaystyle {{A}_{2}}. So, it travels between \displaystyle {{A}_{1}} and \displaystyle {{A}_{2}} with a constant x component of velocity equal to \displaystyle {{v}_{x}}. The total distance travelled by the molecule along x-direction between two successive collisions with face \displaystyle {{A}_{1}} is equal to 2L. Thus, the time between two successive collisions of this molecule with face \displaystyle {{A}_{1}} is \displaystyle \Delta t\,=\,2L/{{v}_{x}}
The force or the momentum imparted per unit time to the wall by this molecule given by
\displaystyle F\,=\,\frac{{\Delta p}}{{\Delta t}}\,=\,\frac{{2mv}}{{2L/{{v}_{x}}}}=\frac{{mv_{x}^{2}}}{L}, \displaystyle {{P}_{x}}\,=\, Pressure exerted by this molecule = \displaystyle \frac{{{{f}_{x}}}}{A}\,=\,\frac{{mv_{x}^{2}}}{{AL}}
Total pressure \displaystyle {{P}_{x}} by n molecules
\displaystyle {{P}_{x}}\,=\,\frac{m}{{Al}}\left[ {{{v}_{x}}_{1}^{2}+vx_{2}^{2}\,+\,……\,+\,vx_{n}^{2}} \right]
Similarly, \displaystyle {{P}_{y}}\,=\,\frac{m}{{Al}}\left[ {{{v}_{y}}_{1}^{2}+vy_{2}^{2}\,+\,……\,+\,vy_{n}^{2}} \right] & \displaystyle {{P}_{z}}\,=\,\frac{m}{{AL}}\left[ {{{v}_{z}}_{1}^{2}+vx_{2}^{2}\,+\,……\,+\,vz_{n}^{2}} \right]
So, average pressure \displaystyle P\,=\,\frac{{{{P}_{x}}+{{P}_{y}}+{{P}_{z}}}}{3}
\displaystyle \therefore \,P\,=\,\frac{m}{{3AL}}\,\left[ {\left( {{{v}_{x}}_{{_{{_{1}}}}}^{2}+{{v}_{y}}_{{_{{_{1}}}}}^{2}\,+\,\,{{v}_{z}}_{{_{{_{1}}}}}^{2}} \right)+\left( {{{v}_{x}}_{{_{2}}}^{2}+{{v}_{y}}_{{_{2}}}^{2}\,+\,\,{{v}_{z}}_{{_{2}}}^{2}} \right)+……\left( {{{v}_{x}}_{{_{n}}}^{2}+{{v}_{y}}_{{_{n}}}^{2}\,+\,\,{{v}_{z}}_{{_{n}}}^{2}} \right)} \right]
\displaystyle \therefore \,\,\,\,P\,=\,\frac{m}{{3\left( {vol} \right)}}\left[ {v_{1}^{2}\,+\,v_{2}^{2}+……….\,+\,v_{n}^{2}} \right]
Multiply and divide by n on RHS
\displaystyle P\,=\,\frac{{mn}}{{3\left( {vd} \right)}}\left( {\frac{{v_{1}^{2}+v_{2}^{2}…….+v_{n}^{2}}}{n}} \right),\,\, Where, \displaystyle \frac{{v_{1}^{2}+v_{2}^{2}…….+v_{n}^{2}}}{n}=v_{{rms}}^{2}
\displaystyle \therefore \,\,\,\,P\,=\,\frac{1}{3}\frac{{mnv_{{rms}}^{2}}}{{vol}} …(1)
Various Speeds of Gas Molecules
The motion of molecules in a gas is characterized by any of the following three speeds.
(1)
Root mean square speed :It is defined as the square root of mean of squares of the speed of different molecules
i.e. {{v}_{{rms}}}=\sqrt{{\frac{{v_{1}^{2}+v_{2}^{2}+v_{3}^{2}+v_{4}^{2}+….}}{N}}}=\sqrt{{\,\overline{{{{v}^{2}}}}}}
since therefore \displaystyle {{v}_{{rms}}}=\sqrt{{\frac{{3PV}}{{mn}}}}
We also know that for one mole PV=RTand number pf particles n=Na (Avogadro’s number)
therefore \displaystyle {{v}_{{rms}}}=\sqrt{{\frac{{3RT}}{{m{{N}_{a}}}}}} also we know that mNa = M (Molar mass)
hence finally we get \displaystyle {{v}_{{rms}}}=\sqrt{{\frac{{3RT}}{M}}}
(2)
Most probable speed :The particles of a gas have a range of speeds. This is defined as the speed which is possessed by maximum fraction of total number of molecules of the gas. e.g., if speeds of 10 molecules of a gas are 11, 22, 22, 16, 16, 16, 14, 15, 12, 20km/s, then the most probable speed is 16km/s, as maximum fraction of total molecules possess this speed.
Most probable speed {{v}_{{mp}}}=\sqrt{{\frac{{2RT}}{M}}}
(3)
Average speed :It is the arithmetic mean of the speeds of molecules in a gas at given temperature.
{{v}_{{av}}}=\frac{{{{v}_{1}}+{{v}_{2}}+{{v}_{3}}+{{v}_{4}}+…..}}{N}
and according to kinetic theory of gases
Average speed {{v}_{{av}}}=\sqrt{{\frac{8}{\pi }\frac{{RT}}{M}}}
Maxwell’s Law on the Distribution of Molecular Speeds
The vrms gives us a general idea of molecular speeds in a gas at a given temperature. This doesn’t mean that the speed of each molecule is vrms. Many of the molecules have speed less than vrms and many have speeds greater than vrms. Maxwell derived as equation given the distribution of molecules in different speed as follow
dN=4\pi N\,{{\left( {\frac{m}{{2\pi kT}}} \right)}^{{3/2}}}{{v}^{2}}{{e}^{{-\frac{{m{{v}^{2}}}}{{2kT}}}}}dv
wheredN = Number of molecules with speeds between v and v + dv.
Graph between \frac{{dN}}{{dv}} (number of molecules at a particular speed) and v (speed of these molecules) is shown below. From the graph it is seen that \frac{{dN}}{{dv}} is maximum at most probable speed.
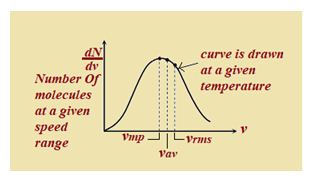
Area bonded by this curve with speed axis represents the number of molecules corresponds to that velocity range. This curve is asymmetric curve.
Kinetic energy of one particle : –
From equation \displaystyle \,\,P\,=\,\frac{1}{3}\frac{{mnv_{{rms}}^{2}}}{{vol}}
\displaystyle mv_{{rms}}^{2}\,=\,3\frac{{PV}}{n}\,\left[ {V\,=\,volume} \right]
\displaystyle \therefore \,\,\,\,\frac{1}{2}\,\,mv_{{rms}}^{2}\,=\,\frac{3}{2}\,\frac{{PV}}{n}
\displaystyle \therefore \,\,\,KE\,=\,\frac{3}{2}\,\frac{{PV}}{n}
For one mole
\displaystyle PV\,=\,RT\,\And \,n\,=\,{{N}_{a}}
\displaystyle \therefore \,\,\,KE\,=\,\frac{3}{2}\frac{{RT}}{{{{N}_{a}}}}\,=\,\frac{3}{2}\,KT
\displaystyle \frac{R}{{{{N}_{a}}}}\,=\,K\,=\, Boltzman constant = \displaystyle 1.3\,\,\times \,{{10}^{{-23}}}\,J/K
Degrees of Freedom
The number of degrees of freedom of a system is divined as the number of independent variables required to describe completely the position, orientation and configuration of the system.
A molecule free to move (translate) in space needs three coordinates to specify its location. We say that it has three translational degrees of freedom. If it is constrained to move (translate) in a plane, it needs two coordinates to specify its location and hence, it has two translational degrees of freedom. If it is constrained to move (translate) in a line, it needs one coordinate to specify its location and hence, it has one translational degree of freedom.
Molecules of monatomic gas like Argon, Helium, etc., have only translational degrees of freedom.
Molecules of diatomic gas like \displaystyle {{O}_{2}} , \displaystyle {{N}_{2}} , etc, have three translational and two rotational degrees of freedom. At very high temperature, in addition to translational and rotational, they have two vibrational degrees of freedom also.
Molecules of polyatomic gas have three translational and three rotational degrees of freedom and may have some vibrational degrees of freedom also.
here we have ignored the vibrational degrees of freedom. Accordingly, we conclude that the number of degrees of freedom are as given below
| No. of degrees of freedom (ignoring vibrational models) | ||
| Translational | Rotational | Total |
Monatomic | 3 | 0 | 3 |
Diatomic | 3 | 2 | 5 |
Polyatomic | 3 | 3 | 6 |
Law of Equipartition of Energy
According to this law, for any system in thermal equilibrium, the total energy is distributed equally amongst all the degrees of freedom with the average energy associated with each degrees of freedom equal to
\displaystyle \frac{1}{2}kT per molecule or \displaystyle \frac{1}{2}RT per mole.
Internal Energy of gas :
The sum of total kinetic energy of all types of motion of all the molecules of a gas is called the internal energy of gas. Here, if we consider only translatory motion then internal energy of a gas is given by total translational kinetic energy of all the molecules hence
\displaystyle U\,=\,n\,\times \,KE (n = total number of particles)
\displaystyle \therefore \,\,U\,=\,n\,.\,\frac{3}{2}\,kT Where, \displaystyle k\,=\,\frac{R}{{{{N}_{a}}}} \displaystyle \therefore \,\,\,U\,=\,\frac{3}{2}\,\,{{N}_{a}}\,\,\frac{R}{{{{N}_{a}}}}T
\displaystyle \frac{U}{{mole}}\,=\,\frac{3}{2}\,RT
Relation between Internal Energy and Degree of freedom
The internal energy of a system is defined as the total energy possessed by the system due to its molecular motion and molecular configuration. The energy due to the molecular motion is called internal kinetic energy and due to the molecular configuration is called internal potential energy. In case of an ideal gas, the intermolecular forces are zero and hence, the internal potential energy which is associated with intermolecular forces is also zero. Therefore, for an ideal gas, the internal energy is just the sum of kinetic energies associated with various random motions of its molecules. We shall denote internal energy by the symbol U and the change in internal energy of the system by \displaystyle \Delta U.
According to law of equal partition of energy if f is total degree of freedom then total KE of a molecule is given as \displaystyle KE=f\,\times \,\frac{1}{2}KT.
\displaystyle \therefore \,\,U\,=\,N\,\times \,KE\,=\,N\,\times \,f\,\times \,\frac{1}{2}\,\frac{R}{{{{N}_{a}}}}\,T\,=\,n\frac{1}{2}\,fRT …(2)
In terms of \displaystyle {{C}_{v}} , U is given as \displaystyle U\,=\,n{{C}_{v}}T …(3)
From (2) & (3)
\displaystyle n{{C}_{v}}T\,=\,n\frac{1}{2}fRT
\displaystyle {{C}_{v}}\,=\,\frac{1}{2}\,fR [When vibration is neglected]
Adiabatic Constant ( \displaystyle \gamma )
It is the ratio of specific heat at constant pressure to specific heat at constant volume and is denoted by the symbol \displaystyle \gamma .
\displaystyle \gamma =\frac{{{{C}_{p}}}}{{{{C}_{v}}}}
Its value is constant for a given gas.
Value of \displaystyle {{C}_{p}} and \displaystyle {{C}_{v}} in terms of \displaystyle \gamma and R
We know that
\displaystyle {{C}_{p}}-{{C}_{v}}\,=\,R and \displaystyle {{C}_{p}}-{{C}_{v}}\,=\,R.
Solving for \displaystyle {{C}_{p}} and \displaystyle {{C}_{v}}\, we obtain
\displaystyle {{C}_{v}}=\,\frac{R}{{\gamma -1}} and \displaystyle {{C}_{p}}=\,\frac{{\gamma R}}{{\gamma -1}}
If f is the number of degrees of freedom of a gas, then
\displaystyle \gamma \,=\,\frac{{{{C}_{p}}}}{{{{C}_{v}}}} \displaystyle \gamma \,=\,\frac{{{{C}_{p}}}}{{{{C}_{v}}}}=\frac{{\left[ {\left( {f\,+\,2} \right)\,/\,2} \right]R}}{{\left( {f/2} \right)R}}=\frac{{f+2}}{f}
Value of \displaystyle \gamma for various gases
As already discussed, the value of \displaystyle \gamma for various gases (ignoring vibrational models) is given below.
Gas | Degrees of freedom (Ignoring vibrational modes) | |||||
Translational | Rotational | Total | \displaystyle {{C}_{v}}=\frac{1}{2}fR | \displaystyle {{C}_{p}}={{C}_{v}}+R | \displaystyle \gamma =\frac{{{{C}_{p}}}}{{{{C}_{v}}}} | |
Monatomic | 3 | 0 | 3 | \displaystyle \frac{3}{2}R | \displaystyle \frac{5}{2}R | \displaystyle \frac{5}{3}=1.67 |
Diatomic | 3 | 2 | 5 | \displaystyle \frac{5}{3}R | \displaystyle \frac{7}{2}R | \displaystyle \frac{7}{5}=1.4 |
Polyatomic (Non-linear) | 3 | 3 | 6 | 3R | 4R | \displaystyle \frac{4}{3}=1.33 |
Mixture of Gases
For a mixture of gases, the number of degrees of freedom of a mixture if given by :
\displaystyle {{f}_{{mix}}}=\frac{{{{n}_{1}}{{f}_{1}}+{{n}_{2}}{{f}_{2}}+{{n}_{3}}{{f}_{3}}+….}}{{{{n}_{1}}+{{n}_{2}}+{{n}_{3}}+….}}
Molecular weight of the mixture is given by
\displaystyle {{M}_{{mix}}}=\frac{{{{n}_{1}}{{M}_{1}}+{{n}_{2}}{{M}_{2}}+{{n}_{3}}{{M}_{3}}+….}}{{{{n}_{1}}+{{n}_{2}}+{{n}_{3}}+….}}
Internal energy of the mixture is given by
\displaystyle {{U}_{{mix}}}={{U}_{1}}+{{U}_{2}}+{{U}_{3}}+…..
Pressure of the mixture is given by
\displaystyle {{P}_{{mix}}}={{p}_{1}}+{{p}_{2}}+{{p}_{3}}+…..
Where, p’s are partial pressure of the gases (Dalton’s law).
Specific heat at constant volume is given by
\displaystyle {{C}_{{{{v}_{{mix}}}}}}=\frac{{{{n}_{1}}{{C}_{{{{v}_{1}}}}}+{{n}_{2}}{{C}_{{{{v}_{2}}}}}+{{n}_{3}}{{C}_{{{{v}_{3}}}}}+…..}}{{{{n}_{1}}+{{n}_{2}}+{{n}_{3}}+….}}
Suppose different gases having number of moles \displaystyle {{n}_{1}},\,\,{{n}_{2}},\,\,{{n}_{3}},\,\,….. specific heat at constant volume
\displaystyle {{C}_{{{{v}_{1}}}}},\,\,{{C}_{{{{v}_{2}}}}},\,\,{{C}_{{{{v}_{3}}}}},\,\,…… and temperature \displaystyle {{T}_{1}},\,\,{{T}_{2}},\,\,{{T}_{3}},\,………. are mixed together, then we have
\displaystyle {{U}_{{mix}}}={{U}_{1}}+{{U}_{2}}+{{U}_{3}}+….
\displaystyle \Rightarrow \left( {{{n}_{1}}+{{n}_{2}}+…} \right){{C}_{{{{v}_{{mix}}}}}}T={{n}_{1}}{{C}_{{{{v}_{1}}}}}{{T}_{1}}+{{n}_{2}}{{C}_{{{{v}_{2}}}}}{{T}_{2}}+….
Hence, the final temperature of the mixture of gases is
\displaystyle T\,=\,\frac{{{{n}_{1}}{{C}_{{{{v}_{1}}}}}{{T}_{1}}+{{n}_{2}}{{C}_{{{{v}_{2}}}}}{{T}_{2}}+{{n}_{3}}{{C}_{{{{v}_{3}}}}}{{T}_{3}}\,+\,……..}}{{\left( {{{n}_{1}}+{{n}_{2}}+{{n}_{3}}+….} \right)\,{{C}_{{{{v}_{{mix}}}}}}}}s
Objective Assignment
Lorem ipsum dolor sit amet, consectetur adipiscing elit. Ut elit tellus, luctus nec ullamcorper mattis, pulvinar dapibus leo.
Subjective Assignment
Lorem ipsum dolor sit amet, consectetur adipiscing elit. Ut elit tellus, luctus nec ullamcorper mattis, pulvinar dapibus leo.
