Video Lecture
Theory For Notes Making
Heat transfer
Heat can be transferred from one place to another by three different methods, namely, conduction convection and radiation. Conduction usually takes place in solids, convection in liquids and gases, and no medium is required for radiation. Radiation is fastest and conduction is slowest.
Thermal conduction
This is the mode of heat transfer in which particles of the medium do not leave their position permanently i.e., they keep vibrating at their own places and transfer energy from one part of the medium to the other part.
Thermal conductivity
The ability of a material to conduct heat is measured by thermal conductivity of the material.
Consider a solid bar of thickness l and cross-sectional area A. Let the left side of the bar is at temperature T1 and right side at T2 where T1>T2.
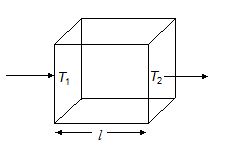
Sooner or later the temperature of each cross-section becomes constant with respect to time. This is known as steady state. If ΔQ amount of heat flows through a cross-section in time Δt under steady state conditions then the rate of heat transfer is given by
\frac{{\Delta Q}}{{\Delta t}}\,=\,\frac{{KA\,({{T}_{1}}-{{T}_{2}})}}{l}
whereK is a constant for the material of the rod and is called thermal conductivity of the material of the rod. It’s unit is J/s-m-K or Cal-s-m-K.
If the area of cross-section is not uniform or if the steady state conditions are not reached, then the equation can only be applied to a thin layer of material perpendicular to the heat flow. If A be the area of cross-section at a place, dx be a small thickness and dT be the temperature difference across the layer of thickness dx then the rate of heat transfer is
\frac{{dQ}}{{dt}}\,=\,-KA\,\frac{{dT}}{{dx}}
The negative sign indicates that \frac{{dT}}{{dx}} is negative along the direction of flow.
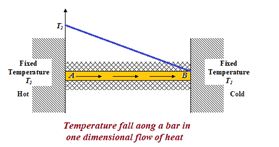
The diagram given below shows how the temperature falls linearly along the length of a bar when its ends are kept at different temperatures.
Thermal Resistance
In the equation \frac{{\Delta Q}}{{\Delta t}}\,=\,\,\frac{{{{T}_{1}}\,-\,{{T}_{2}}}}{{(\frac{l}{{KA}})}}
\frac{{\Delta Q}}{{\Delta t}} If we treat as thermal current and T1 – T2 as the temperature difference due to which the thermal current is flowing, then quantity \frac{l}{{KA}} can be treated as somewhat similar to electrical resistance (think i = \frac{V}{R}, Ohm’s law) and we call it thermal resistance. So thermal resistance of a body depends upon its length, area cross-section and thermal conductivity of the material of the body.
There exists an useful analog between thermal conductivity and electrical conductivity
Combination of Layers
Layers in series
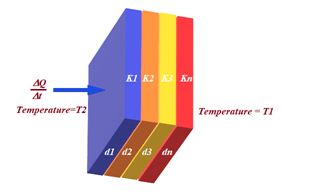
Let us consider a multiplayer medium consisted of layers having area of cross-section A each and width d1, d2, …dn as shown. The thermal conductivities of various layers are K1, K2 …, Kn. Remember in this case the same amount of heat flows through each layer.If a temperature difference of magnitude T2 – T1 is maintained between the near and far faces of the multiplayer (where T2 and T1 are the temperatures of the near and far faces respectively), then rate of heat flow is given by
\frac{{\Delta Q}}{{\Delta t}}\,=\,\frac{{A\,({{T}_{2}}-{{T}_{1}})}}{{\frac{{{{d}_{1}}}}{{{{k}_{1}}}}\,+\,\frac{{{{d}_{2}}}}{{{{k}_{2}}}}\,+\,…\,+\,\frac{{{{d}_{n}}}}{{{{k}_{n}}}}}}
Layers in parallel
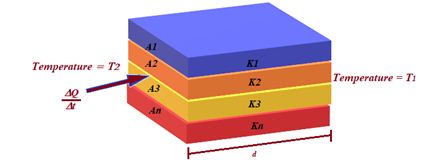
Now let us consider a multiplayer, this time having same width d but different areas of cross-section A1, A2, …An as shown. The thermal conductivities of the various layers are k1, k2, …kn. Remember in this case the rate of heat flow is different in different layers but the temperature difference is same across each layer. If a temperature difference of magnitude (T2 – T1) is maintained between the near and far faces of the multiplayer (where T2 and T1 are the temperatures of near and far faces respectively, then the total rate of heat flow is given by
\frac{{\Delta Q}}{{\Delta t}}\,\,=\,\,\frac{{({{T}_{2}}-{{T}_{1}})\,({{k}_{1}}{{A}_{1}}+{{k}_{2}}{{A}_{2}}+\,…\,+\,{{k}_{n}}{{A}_{n}})}}{d}
Detailed explanation of series and parallel combination can be found in video lectures
Illustration
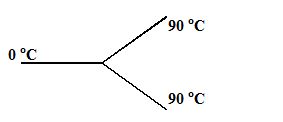
Three rods made of same material and having the same cross-section have been joined as shown in the figure. Each rod is of the same length. The left and right ends are kept at 0oC and 90oC respectively. The temperature of the junction of the three rods will be
(a) 45oC
(b) 60oC
(c) 30oC
(d) 20oC
Solution
Let θ be the temperature of the junction B.
Now, heat current in CB + heat current in DB
= heat current in BA (KCL)
\frac{{90-\theta }}{R}+\frac{{90-\theta }}{R}=\frac{{\theta -0}}{R}
θ = 60o
(b)
Illustration
Two identical conducting rods are first connected independently to two vessels, one containing water at 100oC and the other containing ice at 0oC. In the second case, the rods are joined end to end and connected to the same vessels. Let q1 and q2 g/s be the rate of melting of ice in the two cases respectively. The ratio q1/q2 is
(a)1/2
(b) 2/1
(c)4/1
(d) 1/4
Solution
The rate of the melting of the ice will be inversely proportional to the equivalent thermal resistance between the two vessels.
In the first case, equivalent thermal resistance is \frac{R}{2} and in the second case it is 2R. (whereR is the thermal resistance of the rod).
(c)
Convection
It is the mode of heat transfer by actual motion of matter. It is therefore possible only in fluids. Convection can be natural or forced. Gravity plays an important role in natural convection. Convection involves bulk transport of different parts of the liquid. We can see many examples of heat transfer by convection in our day to day life, right from the circulation of blood in our body to the intricacies of monsoon in India are all examples of convection.
Heat transfer through radiation
Radiation is the mode of heat transfer from one body to other even in the absence of any medium. Before discussing the laws governing radiation we have to understand following terms.
Black body: A black body may be defined as the one that completely absorbs the radiations of all wavelengths incident on it. The concept of black body is an idealised concept and in practice no substance behaves like black body.
Emissive power (e): The emissive power of a body is defined as the energy radiated per unit area per unit time, per unit solid angle perpendicular to the area for all possible wave length. So if energy radiated by the area ΔA of the surface in the solid angle ΔW in time Δt be ΔU the emissive power of the body is given by
E=\,\frac{{\Delta U}}{{(\Delta A)\,\,(\Delta W)\,\,(\Delta t)}}
The unit of emissive power is Joule/sec/m2 or watt/m2.
Absorptive power (a): Absorptive power of a body is defined as the fraction of the incident radiation that is absorbed by the body, in same time.
So, a = \frac{{\mathbf{Energy}\,\,\mathbf{absorbed}}}{{\mathbf{Energy}\,\mathbf{incident}}}
As the entire radiation incident a black body is absorbed, the absorptive power of black body is unity.
Kirchoff’s law of radiation
Kirchoff’s law states that ratio of the emissive power to the absorptive power for the radiation of a given wavelength is same for all bodies at the same temperature and is equal to the emissive power of a perfectly black body at that temperature. Kirchoff’s law in a way tells that a good emitter is a good absorber and a bad absorber is a bad emitter.
So, \frac{{e\,\,\mathbf{(body)}}}{{a\,\,\mathbf{(body)}}}= E (black body) = Constant (Depends of temperature)
Stefan’s law
The energy emitted per second per unit area of a black body is proportional to the fourth power of absolute temperature of the emitter, and is given by
E=\sigma {{T}^{4}} … (i)
wheres is a constant known as Stefan’s constant and its value is 5.67 × 10–8 J/m2-s-K4 For any other body
E=\in \,\sigma {{T}^{4}} … (ii)
Where \in is emissivity which is defined for a surface as the ratio of the emissive power of the surface to the emissive power of black body at the same temperature. The value of \in lies between 0 and 1, it is zero for perfectly reflecting surface and unity for black body.
Net Loss of Heat: The rate at, which a body radiates, is determined by the temperature of the body but the rate at which it absorbs energy by radiation depends on the temperature of surroundings.
So for a body at a temperature of T1 surrounded by walls at temperature T2 the net rate of loss of energy by radiation per unit area per second by radiation is given by
{{E}_{{net}}}=\in \sigma (T_{1}^{4}-T_{2}^{4})
This is also known as Stefan’s Boltzman law.
Newton’s law of cooling
For a small temperature difference between body and surrounding, the rate of cooling of the body is directly proportional to the temperature difference. This can be easily derived taking the value of T to be really close to temperature of surrounding To. Mathematically rate of fall of temperature with respect to time is given as
\displaystyle \frac{{dT}}{{dt}}=-ba(t-{{t}_{0}})
where b is a constant which depends on the nature of the surface involved and surrounding conditions, A is the surface area of the body and (T – T0) is the instantaneous temperature difference between the body and the surrounding. This is known as Newton’s law of cooling. The negative sign indicates that temperature decrease in time. This equation can be used in Celsius scale as well as in absolute scale because difference in temperature is same for absolute as well as for Celsius scale.
Spectral distribution of energy in a black body radiation
A black body emits radiations of different wavelength, however the energy content of radiations of different wavelength is not equal as shown in the graph given below. The relative intensities of different wavelengths depend upon the temperature of radiator
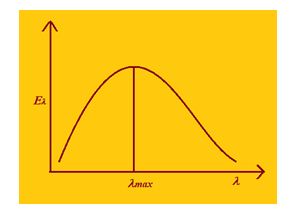
The following observations can be drawn from this diagram
(1)
For a given temperature the graph between energy and wavelength is a continuous arc spreading from a minimum to a maximum and has wavelength (lm) where the emission is maximum.
(2)
As the temperature increases the wavelength at which the emission is maximum, shifts to
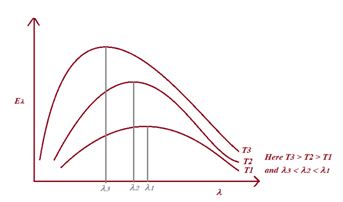
a lower values i.e., {{\lambda }_{m}}T=b where b is a constant having value 2.9 × 10–3 m-K, known as Wein’s constant. This is called Wein’s displacement law
(3)
As the temperature increases, the area enclosed by the curve also increases.
(4)
Area under the curve is proportional to T4 and hence verifies Stefan’s law.
Illustration
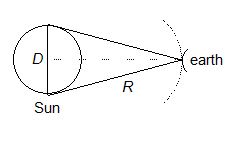
The earth receives solar radiation at a rate of 8.2 J/cm2 – minute. Assuming that the sun radiates like a blackbody. Calculate the surface temperature of the sun. The angle subtended by the sun on the earth is 0.53° and the Stefan constant s = 5.67 × 10-8 W/m2-K4
Solution:
Let the diameter of the sun be D and its distance from the earth be R. From the question,
\frac{D}{R}\,\approx \,\,0.53\,\times \,\frac{\pi }{{180}}= 9.25 × 10–3 … (i)
The radiation emitted by the surface of the sun per unit time is 4\pi \,{{\left( {\frac{D}{2}} \right)}^{2}}\,\sigma {{T}^{4}}\,=\,\pi {{D}^{2}}\sigma {{T}^{4}}.
At distance R, this radiation falls on an area 4pR2 in unit time. The radiation received at the earth’s surface per unit time per unit area is, therefore,
\frac{{\pi {{D}^{2}}\sigma {{T}^{4}}}}{{4\pi {{R}^{2}}}}\,=\,\frac{{\sigma {{T}^{4}}}}{4}\,{{\left[ {\frac{D}{R}} \right]}^{2}}
Thus, \frac{{\sigma {{T}^{4}}}}{4}\,{{\left[ {\frac{D}{R}} \right]}^{2}}\,=\,8.2J/m2 – minute
Or, \frac{1}{4}\,\times \,\left( {5.67\,\times \,{{{10}}^{{-8}}}\,\frac{W}{{{{m}^{2}}-{{K}^{4}}}}} \right)\,{{T}^{4}}\,\times \,{{(9.25\,\times \,{{10}^{{-3}}})}^{2}} = \frac{{8.2}}{{{{{10}}^{{-4}}}\,\times \,60\,}}\,\, \frac{W}{{{{m}^{2}}}}
or, T = 5794 K » 5800 K
Illustration
A copper sphere is suspended in a evacuated chamber maintained at 300 K. The sphere is maintained at constant temperature of 900 K by heating electrically. A total of 300 W electric power is needed to do this. When half of the surface of the copper sphere is completely blackened, 600 W is needed to maintain the same temperature of sphere. The emissivity of copper is
(a) 1/4
(b) 1/3
(c) 1/2
(d) 1
Solution
300=e\sigma A\left( {{{{900}}^{4}}-{{{300}}^{4}}} \right) … (i)
\displaystyle 600=\frac{{\sigma A}}{2}\left( {{{{900}}^{4}}-{{{300}}^{4}}} \right)+\frac{{e\sigma A}}{2}\left( {{{{900}}^{4}}-{{{300}}^{4}}} \right)… (ii)
e=\frac{1}{3}
(b)
Illustration
If the temperature of the sun is increased from T to 2T and its radius from R to 2R, then the ratio of the radiant energy received on earth to what it was previously will be
(a) 4
(b) 16
(c) 32
(d) 64
Solution
P\propto A{{T}^{4}} and A\propto {{r}^{2}}
P\propto {{r}^{2}}{{T}^{4}}
Now, T\prime =2T,\,\,r\prime =2r
Hence, P\prime =4\times 16P=64P
(d)
Illustration
Two spherical black bodies have maximum emission corresponding to wavelengths which are in the ratio of 2 : 3, and their radius 4 : 9. Then ratio of their emissive powers will be (assume same emissivity for body)
(a) 1 : 1
(b) \frac{{81}}{{16}}
(c) \frac{{16}}{{81}}
(d) \frac{8}{{27}}
\frac{{{{T}_{1}}}}{{{{T}_{2}}}}=\frac{{{{\lambda }_{2}}}}{{{{\lambda }_{1}}}}=\frac{3}{2}
\frac{{{{A}_{1}}}}{{{{A}_{2}}}}={{\left( {\frac{{{{r}_{1}}}}{{{{r}_{2}}}}} \right)}^{2}}=\frac{{16}}{{81}}
Solution
{{E}_{1}}=e\sigma A{{T}^{4}}
(a)
Objective Assignment

(a) Each sphere has the same internal energy
(b) There is no net transfer of thermal energy between the spheres
(c) Both spheres radiate electromagnetic energy at the same rate
(d) The larger sphere has a greater mean internal energy per atom then the smaller sphere.
Ans (b)
10.
A black metal foil is warmed by radiation from a small sphere at temperature T and at a distance d. The power received by the foil is P. If both the temperature and the distance are doubled, the power received by the foil will be
(a) 2 P
(b) 1 P
(c) 16 P
(d) 4 P
Ans (4)
11.
Water can be heated in cup of paper because
(a) paper is good conductor of heat
(b) paper has high specific heat
(c) paper is thin
(d) paper is good radiator of heat
Ans (c)
12.
A sphere, a cube and a disc all of the same material, quality and volume are heated to 600°C and left in air.Which of these will have the lowest rate of cooling?
(a) Sphere
(b) Cube
(c) Disc
(d) All will have same rate
Ans (a)
13.
Newton’s law of cooling is a special case of
(a) Stefan’s law
(b) Boltzmann’s low
(c) Wien’s law
(d) Planck’s law
Ans (a)
Subjective Assignment
Lorem ipsum dolor sit amet, consectetur adipiscing elit. Ut elit tellus, luctus nec ullamcorper mattis, pulvinar dapibus leo.
