Video Lectures
Theory For Making Notes
Introduction
An object launched with certain initial velocity and following a curved path under the gravitational field of earth alone is called projectile and its motion is called projectile motion. This definition includes the effect of air resistance, rotation and curvature of the earth and the variation of acceleration due to gravity of the earth.
Generally the projectile motion of body near earth surface is approximated by assuming body as a point particle moving under the field of earth having constant value of g and following a parabolic path in the absence of air resistance, rotation and curvature effect of earth.
Note that
The motion of projectile is two-dimensional motion contained in a plane having and initial velocity vector of projection. And the motion of projectile can be represented as superposition of the uniform horizontal motion and the uniform accelerated vertical motion.
Analysis of Projectile Motion
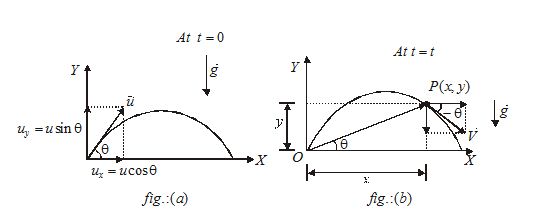
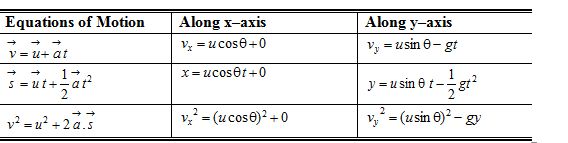
Consider a projectile is launched from the origin of rectangular coordinate system, having x-axis parallel to horizon and y-axis perpendicular to the ground, with initial velocity making an angle θ with the x-axis or with horizon as shown in figure (a). As discussed, the motion of projectile is uniform motion along x–axis and uniform accelerated motion, of acceleration \vec{g}=-g\hat{j}, along the y–axis, so the equations of motion of the projectile at an instant of time , as shown in figure (b), are given as
Time of flight (T)
It is time from point of projection to a point on the same horizontal level passing through the point of projection
Applying 2nd equation of motion along y axis
\displaystyle y=ut-\frac{1}{2}g{{t}^{2}}
At the landing point t = T, and y = 0.
\displaystyle 0=ut-\frac{1}{2}g{{t}^{2}} or
\displaystyle t=\frac{{2u\sin \theta }}{g} ——- (3)
Note : Time of flight is maximum at = 90o.
Equation of Trajectory
The path followed by a body is known as its trajectory. Mathematically it is defined as a relation between the x and y coordinates of a body whose graph represents the path of the body.
We know that the x coordinate of the projectile is given by
\displaystyle x=u\cos \theta .t hence \displaystyle t=\frac{x}{{u\cos \theta }}
and y coordinate is given by \displaystyle y=u\sin \theta t-\frac{1}{2}g{{t}^{2}}
substituting t in the equation of y coordinate we get
\displaystyle y=u\sin \theta.\frac{x}{{u\cos \theta }}-\frac{1}{2}g{{\left( {\frac{x}{{u\cos \theta }}} \right)}^{2}}
hence \displaystyle y=x\tan \theta -\frac{1}{2}\frac{{g{{x}^{2}}}}{{{{u}^{2}}{{{\cos }}^{2}}\theta }}
The above equation is the equation of trajectory of a projectile
This is an equation of a parabola of the type y=ax-b{{x}^{2}}.
Thus the trajectory of projectile is a parabola in the absence of air resistance, rotation and curvature effect of the earth.
Calculation Of Range & Maximum Height From Equation Of trajectory
Range (R):It is the horizontal distance covered by particle from the point of projection to the point of landing on the horizontal surface passing throught the point of projection
we know that \displaystyle x=u\cos \theta .t
For the landing point put \displaystyle t=\frac{{2u\sin \theta }}{g} and x=R.
hence \displaystyle R=u\cos \theta .\frac{{2u\sin \theta }}{g}
\displaystyle or\text{ }R=\frac{{{{u}^{2}}\sin 2\theta }}{g}
Alternate Method
At the point of landing the x coordinate is equal to R and y coordinate is equal to 0. Hence on substituting these coordinates in equation of trajectory we get
0=R\tan \theta -\frac{1}{2}\frac{{g{{R}^{2}}}}{{{{u}^{2}}{{{\cos }}^{2}}\theta }}
R=\frac{{2{{u}^{2}}\sin \theta \cos \theta }}{g}=\frac{{{{u}^{2}}\sin 2\theta }}{g}
Note that the range R is maximum when projectile is projected at an angle 45° .
{{R}_{{\max }}}=\frac{{{{u}^{2}}\sin (2\times 45{}^\circ )}}{g}=\frac{{{{u}^{2}}\sin 90{}^\circ }}{g}=\frac{{{{u}^{2}}}}{g}
III. Maximum Height (H):It is the highest distance of projectile above the ground in its trajectory.
The coordinates of the highest point are (R/2,H). On sbstituting these coordinates in equation of trajetory we can get the maximum height.
Alternatively we can also say yhat at the maximum height the verticle component of the velocity is zero thus when {S}_{y}=H then {{v}_{y}}=0. Now from the third equation of motion for y–axis, i.e.
\displaystyle v_{y}^{2}=u_{y}^{2}-2g{{S}_{y}} we get
0={{(u\sin \theta )}^{2}}-2gH
or H=\frac{{{{u}^{2}}{{{\sin }}^{2}}\theta }}{2g}
Important Points
1
In projectile motion, the time required to reach maximum height from the point of projection on horizontal ground is equal to the time required to return back on the ground i.e., the time of ascent is equal to the time of descent thus,
{{t}_{a}}={{t}_{d}}=\frac{T}{2}=\frac{{u\sin \theta }}{g}
2.
The velocity of projectile at the highest point has only horizontal component i.e., at the highest point
{{v}_{y}}=0 and {{v}_{x}}=u\cos \theta
Therefore, \overset{\to }{\mathop{v}}\,=u\cos \,\theta \,\hat{i}
3.
When the projectile strike the ground, then
{{v}_{x}}=u\cos \theta and {{v}_{y}}=u\sin \theta -g\left( {\frac{{2u\sin \theta }}{g}} \right)=-u\sin \theta

Therefore, \overset{\to }{\mathop{v}}\,=u\cos \theta \,\,\hat{i}-u\sin \theta \,\,\hat{j} and |\overset{\to }{\mathop{v}}\,|=u
Thus the projectile hit ground with the same speed with which it was projected and it makes same angle but below the horizon as shown in figure.
Relation between R, H and T
(i) R=4H\cot \theta
(ii) T=2\sqrt{{2gH}}
(iii) R=\frac{1}{2}g{{T}^{2}}\cot \theta
Properties of complimentary angles of projection
(i)
The horizontal ranges are equal for complementary angles of projections
Let {{\theta }_{1}} and {{\theta }_{2}} are the two angles of projection such that
{{\theta }_{1}}+{{\theta }_{2}}=90{}^\circ .
If R1 and R2 be the range at two different angles q1 an q2
then {{R}_{1}}=\frac{{{{u}^{2}}\sin 2{{\theta }_{1}}}}{g} and {{R}_{2}}=\frac{{{{u}^{2}}\sin 2{{\theta }_{2}}}}{g}
So {{R}_{1}}=\frac{{2{{u}^{2}}\sin {{\theta }_{1}}\cos {{\theta }_{1}}}}{g} and {{R}_{2}} =\frac{{2{{u}^{2}}\cos {{\theta }_{2}}\sin {{\theta }_{2}}}}{g}
If we put {{\theta }_{1}}=90{}^\circ -{{\theta }_{2}} in equation of R1 we find that
\displaystyle {{R}_{1}}=\frac{{2{{u}^{2}}\sin (90-{{\theta }_{2}})\cos (90-{{\theta }_{2}})}}{g}=\frac{{2{{u}^{2}}\cos {{\theta }_{2}}.\sin {{\theta }_{2}}}}{g}={{R}_{2}}
Hence {{R}_{1}}={{R}_{2}} if {{\theta }_{1}}+{{\theta }_{2}}=90{}^\circ
(ii)
The complementary angles are symmetrically located about an angle 45{}^\circ i.e., if
{{\theta }_{1}}=45{}^\circ +\alpha , then {{\theta }_{2}}=45{}^\circ -\alpha
(iii)
For complementary angles of projection, i.e., \theta and 90-\theta , the ratio of the maximum heights attained is equal to tan^{2}\theta and sum of these heights is equal to \frac{{{{u}^{2}}}}{{2g}}.
Let H1 and H2 are the maximum heights for \theta and 90-\theta angles of projection, then
{{H}_{1}}=\frac{{{{u}^{2}}{{{\sin }}^{2}}\theta }}{{2g}}
and {{H}_{2}}=\frac{{{{u}^{2}}{{{\sin }}^{2}}(90-\theta )}}{{2g}}=\frac{{{{u}^{2}}{{{\cos }}^{2}}\theta }}{{2g}}
Therefore \frac{{{{H}_{1}}}}{{{{H}_{2}}}}=\frac{{{{{\sin }}^{2}}\theta }}{{{{{\cos }}^{2}}\theta }}={{\tan }^{2}}\theta
and {{H}_{1}}+{{H}_{2}}=\frac{{{{u}^{2}}}}{{2g}}({{\sin }^{2}}\theta +{{\cos }^{2}}\theta )=\frac{{{{u}^{2}}}}{{2g}}
(iv)
For complimentary angles of projection, product of the times of flight is proportional to their common range.
Let {{T}_{1}} and {{T}_{2}} are the time of flight for angle of projections \theta and 90-\theta , then
{{T}_{1}}=\frac{{2u\sin \theta }}{g}and {{T}_{2}}=\frac{{2u\sin (90-\theta )}}{g}=\frac{{2u\cos \theta }}{g}
Therefore,
{{T}_{1}}\,\,{{.T}_{2}}=\left( {\frac{{2u\sin \theta }}{g}} \right)\left( {\frac{{2u\cos \theta }}{g}} \right) =\frac{2}{g}\left( {\frac{{{{u}^{2}}\sin 2\theta }}{g}} \right)=\frac{2R}{g}
Hence {{T}_{1}}\,{{.T}_{2}}\propto R
(v)
The equation of trajectory can be written in terms of range R as
y=x\tan \theta \left( {1-\frac{x}{R}} \right)
Effect of air resistance
In presence of air resistance, range, maximum height attained, time of ascent and flight reduces but time of decent and angle of landing increases.
Projectile Motion on an Inclined Plane
Suppose a projectile is thrown at an angle a to the horizontal with a speed u on a plane which makes an angle b to the horizontal. We wish to find the displacement of the projectile on the plane by . For this purpose, we choose a reference frame with x axis along the plane and y axis perpendicular to the plane as shown in the figure.
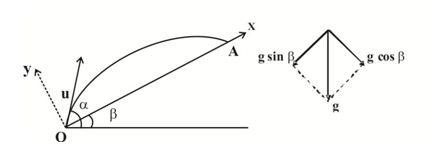
Now, we resolve the quantity along the plane and perpendicular to the plane i.e. along x axis and y axis,
ux= u cos (α – β)
uy= u sin (α – β)
ax= –g sin β
ay= –g cos β
Note that the y–component of the displacement is zero in traveling from O to A. Thus using
sy = uyt + \displaystyle \frac{1}{2}{{a}_{y}}{{t}^{2}}
we have
0 = u sin (α – β)t + \displaystyle \frac{1}{2} (-g cos b) t2
Hence t = \displaystyle \frac{{2u\sin \left( {\alpha -\beta } \right)}}{{g\cos \beta }} —-(1)
Next using sx = uxt + \displaystyle \frac{1}{2}axt2
OA = u cos (α – β) t + \displaystyle \frac{1}{2} (-g sin β) t2
Putting the value of t from equation (1), we have
OA = \displaystyle \frac{{2{{u}^{2}}\cos \alpha \sin \left( {\alpha -\beta } \right)}}{{g{{{\cos }}^{2}}\beta }}
or OA = \displaystyle \frac{{{{u}^{2}}}}{{g{{{\cos }}^{2}}\beta }} [sin(2α – β) – sinb] —–(2)
This is the required expression for range on an inclined plane. This range will be maximum when
sin (2α – β) = 1 or hence 2a – b = p/2
or \displaystyle \alpha =\frac{1}{2}\left( {\beta +\frac{\pi }{2}} \right). It is the angle of projection at which range becomes maximum on an inclined surface.
Let maximum value of range `OA’ is denoted by Rmax .
Rmax can be obtained by puting sin (2α – β) = 1 in equation (2) so we get
Rmax= \displaystyle \frac{{{{u}^{2}}}}{{g{{{\cos }}^{2}}\beta }} [1 – sinb]= \displaystyle \frac{{{{u}^{2}}\left( {1-\sin \beta } \right)}}{{g\left( {1-{{{\sin }}^{2}}\beta } \right)}}=\frac{{{{u}^{2}}}}{{g\left( {1+\sin \beta } \right)}}
Illustration
A ball is projected horizontally at 20 m/s from a cliff of height 45 m
(a) Find its time of flight
(b) Find its horizontal range R (the horizontal displacement from the point of firing).
Solution
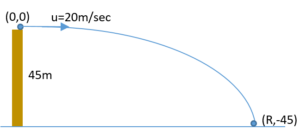
The origin is assumed to be at the point of projection on the cliff
Given:
ux= 20 m/s and uy= 0
(a)
Applying \displaystyle {{S}_{x}}={{u}_{x}}t and \displaystyle {{S}_{y}}={{u}_{y}}t+\frac{1}{2}{{a}_{y}}{{t}^{2}} from point of projection to landing point.
Putting values of ux , uy , and Sx = R , Sy = – 45
We get
R = 20 t … (i)
and -45 = – 5t2
or t = 3 s … (ii)
(b) To find the horizontal range we use the equation (i)
R = 20t = (20) (3) = 60 m
Illustration
Find the average velocity of a projectile between the instants it crosses one third the maximum height. It is projected with u making an angle q with the vertical.
(a) u cos q
(b) u
(c) u sin q
(d) u tan q

Solution
Note carefully the vertical velocities at the same height are in opposite direction and therefore their average sum = 0. It is horizontal velocity which is uniform and hence vav = u sin θ
Illustration
A person observes a bird on a tree 40 m high and at a distance of 60 m. With what velocity the person should throw an arrow at an angle of 45o so that it may hit the bird? g = 10 m/s2.
Solution
Let u be the initial velocity of the arrow and let it hit the bird after t.
The horizontal component of velocity u is u cos 45o = \frac{u}{{\sqrt{2}}}. Hence the horizontal distance traveled in t s is \frac{u}{{\sqrt{2}}}x t = 60 … (1)
The vertical distance traveled in time t s is 40 m, acceleration g = – 10 m/s2 and initial vertical velocity = u sin 45o = \frac{u}{{\sqrt{2}}}. Hence by the formula h = ut + \displaystyle \frac{1}{2}gt2, we have
40 = \displaystyle \frac{u}{{\sqrt{2}}}t-\frac{1}{2}\left( {10} \right){{t}^{2}} … (2)
By equation (1) and (2), we get
40 = 60 – \displaystyle \frac{1}{2}\left( {10} \right){{t}^{2}}
or t2 = \displaystyle \frac{{60-40}}{5}=\frac{{20}}{5}=4
t = 2 s
Substituting the value of t in equation (1), we get
\frac{u}{{\sqrt{2}}}´ 2 = 60
u = 30 \displaystyle \sqrt{2} m/s
Illustration
A bomb is fired from a cannon with a velocity of 1000 m/s making an angle of 30o with the horizontal (g = 10 m/s2).
(i) What is the time taken by the bomb to reach the highest point?
(ii) What is the total time of its motion ?
(iii) With what speed the bomb will hit the ground and what will be its direction of motion while hitting?
(iv) What is the maximum height attained by the bomb?
(v) At what distance from the cannon the bomb will hit the ground ?
Solution
(i) Let u be the initial velocity of the bomb and q its angle of projection. The time taken by the bomb to reach the highest point is given by
t = \displaystyle \frac{{u\sin {{\theta }}}}{g}=\frac{{1000\times \sin 30{}^\circ }}{{10}}=\frac{{1000\times \frac{1}{2}}}{{10}} = 50 s
(ii) The total time of its motion is, T = 2t = 2 x 50 = 100 s.
(iii) The bomb will hit the ground with the same speed with which it was fired. Hence its speed of hitting = 1000 m/s. Also, the angle of hitting with respect to the horizontal is 30o.
(iv) The maximum height attained by the bomb is
h = \displaystyle \frac{{{{u}^{2}}{{{\sin }}^{2}}{{\theta }}}}{{2g}}
= \displaystyle \frac{{{{{\left( {1000} \right)}}^{2}}\times {{{\sin }}^{2}}{{{30}}^{o}}}}{{2\times 10}}=\frac{{{{{\left( {1000} \right)}}^{2}}\times {{{\left( {0.5} \right)}}^{2}}}}{{2\times 10}} =1.25 x 104 m
(v) Horizontal range is
R = \displaystyle \frac{{{{u}^{2}}\sin 2{{\theta }}}}{g}=\frac{{{{{\left( {1000} \right)}}^{2}}\times \sin {{{60}}^{o}}}}{{10}} = 8.66 x 104 m
Illustration
A ball is thrown from the top of a tower with an initial velocity of 10 m/s at an angle of 30o with the horizontal. It hits the ground at a distance of 17.3 m from the base of the tower. Calculate the height of the tower. (g = 10 m/s2)
Solution
The angle of projection of the ball is qo (=30o) and the velocity of projection is u (= 10 m/s). Resolving u in horizontal and vertical components, we have horizontal component,
ux= u cos qo = 10 cos 30o = 8.65 m/s
and vertical component (upward), uy= u sin30o = 5 m/s.
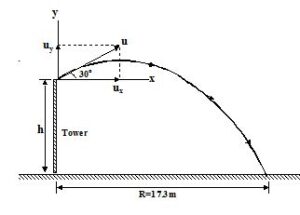
If the ball hits the ground after time t of projection, then the horizontal range is
R = ux X t = 8.65 t m
t = \displaystyle \frac{R}{{8.65}}=\frac{{17.3}}{{8.65}} = 2 s
If h be the height of the tower, then
h = uyt + \frac{1}{2}gt2
Here uy= 5 m/s , t = 2 s and g = -10m/s2
h = (5) x 2 + 1/2 x (-10) x (2)2 = 10 – 20 = – 10 m
The negative sign shows that the displacement is negative (downward).
Thus the height of the tower = 10 m
Illustration
A foot ball is kicked with a velocity of 20 m/s at an angle of 45° with the horizontal. (a) Find the time taken by the ball to strike the ground, (b) Find the maximum height it reaches. (c) How far away from the kick does it hit the ground? Take g = 10 m/s2.
Solution
(a) Take the origin at the point where the ball is kicked, vertically upward as the Y-axis and the horizontal in the plane of motion as the X-axis. The initial velocity has the components
\displaystyle {{u}_{x}}=(20\text{m/s})\cos 45{}^\circ =10\sqrt{2}\text{m/s}
& \displaystyle {{u}_{y}}=(20\text{m/s})\sin 45{}^\circ =10\sqrt{2}\text{m/s}
When the ball reaches the ground, y = 0.
Using \displaystyle y={{u}_{y}}t-\frac{1}{2}g{{t}^{2}}
\displaystyle 0=(10\sqrt{2}\text{m/s})t-\frac{1}{2}\times (10\,\text{m/}{{\text{s}}^{2}})\times {{t}^{2}}
or, \displaystyle t=2\sqrt{2}s=2.8s.
Thus, it takes 2.8s for the football to fall on the ground
(b) At the highest point Using the equation
\displaystyle v_{y}^{2}=u_{y}^{2}-2gy,
\displaystyle 0={{(10\sqrt{2}\text{m/s})}^{2}}-2\times (10\text{m/}{{\text{s}}^{2}})H
or, H = 10m.
Thus, the maximum height reached is 10 m.
(c) The horizontal distance traveled before falling to the ground is
x = uxt
x = \displaystyle (10\sqrt{2}\text{m/s})\,\,(2\sqrt{2}\text{s})=40\text{m}
Illustration
A ball is projected with velocity v0 and at an angle of projection a. After what time is the ball moving at right angles to the initial direction?

Solution
We choose x-axis along the initial velocity. If after time t the velocity v is perpendicular to initial direction, then vx must be zero after time t,
i.e. \displaystyle 0={{v}_{0}}-(g\sin \alpha )t
or \displaystyle t=\frac{{{{v}_{0}}}}{{g\sin \alpha }}
Practice Questions (Basic Level)
1.
Which of the following is the largest when the height attained by projectile is the greatest?
(a) Angle of projectile with the vertical (b) Range
(c) Time of light (d) None of these
Ans (c)
2.
A projectile is thrown to have the maximum possible horizontal range of 400 m. Taking the point of projection as the origin, the co-ordinate of the point where the velocity of the projectile is minimum are
(a) (400m, 200m) (b) (200m, 200m)
(c) (400m, 100m) (d) (200m, 100m)
Ans (d)
3.
In case of a projectile, what is the angle between its instantaneous velocity and acceleration at the highest point?
(a) 180° (b) 90° (c) 45° (d) 0°
Ans (b)
4.
The range of a projectile when fired at 75° with the horizontal is 0.5 km. What will be its range when fired at 45°?
(a) 2 km (b) 1.5 km (c) 1 km (d) 0.5 km
Ans (c)
5.
If a man wants to hit a target, he should aim his rifle in a direction:
(a) Lower than the target (b) Of the target
(c) Higher than the target (d) None of these
Ans (c)
6.
The x and y coordinates of a particle at any time t are given by x=7t+4{{t}^{2}} and y=5t where x and y are in metre and t in second. The acceleration of the particle at t=5s is
(a) \mathbf{40}\,\mathbf{m}{{\mathbf{s}}^{{-\mathbf{2}}}} (b) 20\,\mathbf{m}{{\mathbf{s}}^{{-\mathbf{2}}}} (c) \mathbf{8}\,\,\mathbf{m}{{\mathbf{s}}^{{-\mathbf{2}}}} (d) Zero
Ans (c)
7.
The coordinate of a moving particle at any instant of time t are x= at and y=b{{t}^{2}}. The trajectory of the particle is
(a) y=\frac{{{{b}^{2}}x}}{{{{a}^{2}}}} (b) y=\frac{{{{b}^{2}}{{x}^{2}}}}{{{{a}^{2}}}} (c) {{y}^{2}}=\frac{{{{b}^{2}}x}}{{{{a}^{2}}}} (d) y=\frac{{b{{x}^{2}}}}{{{{a}^{2}}}}
Ans (d)
8.
The equation of motion of a projectile is y=12x-\frac{3}{4}{{x}^{2}}. What is the range of the projectile?
(a) 3m (b) 4m (c) 12m (d) 16m
Ans (d)
9.
A passenger traveling in a train, which is moving with constant velocity, drops a stone from the window. To the passenger, the path of projectile will appear to be:
(a) An arc of a circle (b) An elliptical (c) A straight line (d) A parabola.
Ans (c)
10.
Two projectiles are fired at different angles with the same magnitude of velocity such that they have the same range. At what angles they might have been projected?
(a) 35° and 75° (b) 25° and 65° (c) 10° and 50° (d) None of these
Ans (b)
11.
Four projectiles are fired with the same speed at the angles 25°, 40°, 55° and 70° with the horizontal. The range of projectile will be largest for the one projected at an angle
(a) 70° (b) 40° (c) 55° (d) 25°
Ans (b)
12.
From the top of a tower, which is 19.6 m high, a ball is thrown horizontally. If the line joining the point of projection to the point where it hits the ground makes an angle of 45° with the horizontal, then the initial velocity of the ball is
(a) 9.8 m/s (b) 4.9 m/s (c) 14.7 m/s (d) 2.8 m/s
Ans (a)
13.
The pervious question, the magnitude of the velocity of ball when it collide with the ground is
(a)9.8m/s (b) 9.8\sqrt{3}\,\mathbf{m/s} (c) 19.6 m/s (d) 9.8\sqrt{5}\,\mathbf{m/s}
Ans (d)
14.
An aero-plane is moving horizontally with a velocity of magnitude u. It drops a packet when it is at a height h. The time taken by the packet to reach the ground is
(a) \sqrt{{\frac{{2u}}{g}}} (b) \sqrt{{\frac{{2g}}{h}}} (c) \sqrt{{\frac{u}{{2g}}}} (d) \sqrt{{\frac{{2h}}{g}}}
Ans (d)
Practice Questions (JEE Main Level)
1.
If projectile is thrown in a viscous medium offering resistance equal to one-tenth of the acceleration due to gravity, the time of flight of projectile will
(a) increase by 1% (b) decrease by 1%
(c) increase by 2% (d) decrease by 2%
Ans (c)
2.
A ball thrown by one player reaches the other in 2 second. The maximum height attained by the ball above the point of projection is about
(a) 10 m (b) 7.5 m (c) 5 m (d) 2.5 m
Ans (c)
3.
Two stones are projected with the same speed but making different angles with the horizontal. Their ranges are equal. If the angle of projection of one is 60o and its maximum height is y1, then the maximum height of the other is
(a) {{y}_{1}}/2 (b) {{y}_{1}}/3 (c) 3{{y}_{1}} (d) 2{{y}_{1}}
Ans (b)
4.
Two bullets are fired at different angles with the same speed to have the same horizontal range . The ratio of their times of flight is
(a) 1:\tan \theta (b) {{\tan }^{2}}\theta :1 (c) 1:1 (d) \tan \theta :1
Ans (d)
5.
If a particle moves according to the equation y=x-\frac{{{{x}^{2}}}}{2}, then the maximum height reached by the particle is
(a) 1 m (b) 1/3 m (c) 1/2 m (d) 1/4 m
Ans (c)
6.
A particle is projected at an angle of 30° with the horizon. The time corresponding to height h while ascending and while descending are t1and t2 respectively. The speed of projection of particle is
(a) g({{t}_{1}}+{{t}_{2}}) (b) \frac{{g({{t}_{1}}+{{t}_{2}})}}{2} (c) g{{t}_{1}} (d) g{{t}_{2}}
Ans (a)
7.
Two parallel buildings are x distance apart. A boy throws a ball horizontally with 10 m/s from one of the building which fall 10 m below in the opposite building. Then the value of x is
(a) 20 m (b) 10 m (c) 10\sqrt{2}\,\,\mathbf{m} (d) 10\sqrt{3}\,\,\mathbf{m}
Ans (c)
8.
Two particles A and B are projected from the ground toward each other with speeds 10m/s and 6\sqrt{2}\,\mathbf{m/s} respectively. They are projected at angles 37° and 45°, respectively, with horizontal direction from two points separated by a distance of 9.8 m. How much time will they take to collide?
(a) 0.7s (b) 1.2s (c) 1.4s (d) 1.5s
Ans (a)
9.
In previous question, at what height from the ground both particles will collide?
(a) 151 cm (b) 430 cm (c) 22.5 cm (d) 180 cm
Ans.(d)
10.
A projectile is required to hit a target whose coordinates relative to horizontal and vertical axes through the point of projection are (\alpha ,\beta ). If the gun velocity is \sqrt{{2g\alpha }}, and the angle of projection is q where tanq=2. Then it is impossible to hit the target if
(a) \beta < \frac{3}{4}\alpha
(b) \beta \ge \frac{4}{3}\alpha
(c) \beta >\frac{3}{4}\alpha
(d) \beta \ge \frac{1}{4}\alpha
Ans (a)
11.
A ball rolls from the top of a stair case with a horizontal velocity . The steps are h in height and b in wide. The ball will hit the edge of the nth step if
(a) n=\frac{{2h{{u}^{2}}}}{{gb}}
(b) n=\frac{{h{{u}^{2}}}}{{g{{b}^{2}}}}
(c) n=\frac{{2hu}}{{g{{b}^{2}}}}
(d) n=\frac{{2h{{u}^{2}}}}{{g{{b}^{2}}}}
Ans (d)
12.
A number of bullets are fired in all possible directions with the same initial velocities each of magnitude u. The maximum area of the ground covered by bullets is
(a) \pi {{\left( {\frac{{{{u}^{2}}}}{g}} \right)}^{2}}
(b) \pi {{\left( {\frac{{{{u}^{2}}}}{{2g}}} \right)}^{2}}
(c) \pi {{\left( {\frac{u}{g}} \right)}^{2}}
(d) \pi {{\left( {\frac{u}{{2g}}} \right)}^{2}}
Ans (a)
13.
A particle is moving in x-y plane. At certain instant of time, the components of its velocity and acceleration are as follows {{v}_{x}}=3\,\mathbf{m/s}, {{v}_{y}}=4\,\mathbf{m/s}, {{a}_{x}}=2\,\mathbf{m/}{{\mathbf{s}}^{\mathbf{2}}} and {{a}_{y}}=1\,\mathbf{m/}{{\mathbf{s}}^{\mathbf{2}}}. The rate of change of speed at this moment is
(a) \sqrt{{10}}\,\mathbf{m/}{{\mathbf{s}}^{\mathbf{2}}}
(b) 4\,\mathbf{m/}{{\mathbf{s}}^{\mathbf{2}}}
(c) \sqrt{5}\,\mathbf{m/}{{\mathbf{s}}^{\mathbf{2}}}
(d) 2\,\mathbf{m/}{{\mathbf{s}}^{\mathbf{2}}}
Ans. (d)
14.
If the maximum height attained by a projectile is increased by 1% keeping angle of projection constant, the percentage increase in range is
(a) 1%
(b) 2%
(c) 3%
(d) 4%
Ans. (a)
15.
The vertical height of the projectile at time t is given by y=4t-{{t}^{2}}, and the horizontal distance covered is given by x=3t. What is its angle of projection with the horizontal?
(a) {{\tan }^{{-1}}}\frac{3}{5}
(b) {{\tan }^{{-1}}}\frac{4}{5}
(c) {{\tan }^{{-1}}}\frac{4}{3}
(d) {{\tan }^{{-1}}}\frac{4}{3}
Ans (c)
16.
A gun shoots bullets that leave the muzzle at 250 m/s. If the bullet is to hit a target 100 m away at the level of the muzzle, the gun must be aimed at a point above the target. How far above the target is this point.
(a) 100 cm
(b) 80 cm
(c) 75 cm
(d) 50 cm
Ans (b)
17.
A particle is projected at the angle (45° – q) and another at (45° + q). They land at the level from which they were fired. Find the difference in their times of flight if the speed of projection in each case is v0.
(a) 8\sqrt{2}\frac{{{{v}_{o}}\sin \theta }}{g}
(b) 5\sqrt{2}\frac{{{{v}_{o}}\sin \theta }}{g}
(c) 2\sqrt{2}\frac{{{{v}_{o}}\sin \theta }}{g}
(d) 3\sqrt{3}\frac{{{{v}_{o}}\sin \theta }}{g}
Ans (c)
18.
For a given horizontal range, less than the maximum, there are two possible projection angles, \displaystyle \theta 1 and \displaystyle \theta 2. If \displaystyle \theta 1 < \displaystyle \theta 2 , then the relation between the time of flights and the range is
(a) \displaystyle \frac{R}{{{{t}_{1}}}}=\frac{{2g}}{{{{t}_{2}}}}
(b) \displaystyle \frac{R}{{{{t}_{1}}}}=\frac{g}{{2{{t}_{2}}}}
(c) \displaystyle \frac{R}{{{{t}_{1}}}}=\frac{{g{{t}_{2}}}}{3}
(d) \displaystyle \frac{R}{{{{t}_{2}}}}=\frac{{g{{t}_{1}}}}{2}
Ans (d)
Practice Questions (JEE Advance Level)
1.
Velocity and acceleration of a particle at some instant of time are \vec{v}=(2\hat{i}-\hat{j}+2\hat{k})m/s and \vec{a}=(\hat{i}+6\hat{j}-\hat{k})m/s2. Then, the speed of the particle is
(a) increasing at the rate of 2m/s2 (b)decreasing at the rate of 2m/s2
(c) increasing at the rate of 4 m/s2 (d) decreasing at the rate of 4 m/s2
Ans (b)
2.
The acceleration of a particle is given by \vec{a}=\left[ {2\hat{i}+6t\hat{j}+\frac{{2{{\pi }^{2}}}}{9}\cos \frac{{\pi t}}{3}\hat{k}} \right]ms–2.
At t = 0, \vec{r}= 0 and \vec{v}=(2\hat{i}+\hat{j})m/s. The position vector at t = 2 s will be
(a) \left( {8\hat{i}+10\hat{j}+\hat{k}} \right)m (b) \left( {8\hat{i}+10\hat{j}+3\hat{k}} \right)m
(c) \left( {3\hat{i}+8\hat{j}+10\hat{k}} \right)m (d) \left( {10\hat{i}+3\hat{j}+8\hat{k}} \right)m
Ans (b)
3.
A particle of mass m moves on the x-axis under the influence of a force of attraction towards the origin O given by F=\frac{k}{{{{x}^{2}}}}\hat{i}. If the particle starts from rest at x = a, the speed it will attain to reach at distance x from the origin O will be
(a) \sqrt{{\frac{{2k}}{m}}}{{\left[ {\frac{{x-a}}{{ax}}} \right]}^{{\frac{1}{2}}}}
(b) \sqrt{{\frac{{2k}}{m}}}{{\left[ {\frac{{x+k}}{{ax}}} \right]}^{{\frac{1}{2}}}}
(c) \sqrt{{\frac{k}{m}}}\left[ {\frac{{ax}}{{x-a}}} \right]
(d) \sqrt{{\frac{m}{{2k}}}}{{\left[ {\frac{{a-x}}{{ax}}} \right]}^{{\frac{1}{2}}}}
Ans (a)
4.
5.
The velocities in x and y direction of a particle moving in xy plane is given by {{v}_{x}}={{a}_{0}}t;\,\,{{v}_{y}}=\lambda x where {{a}_{0}} and l are some constants, t is time and x is the displacement in x direction at any time t. The trajectory of the particle will be
(a) {{y}^{3}}=\frac{{2{{\lambda }^{2}}}}{{9{{a}_{0}}}}{{x}^{2}}
(b) {{y}^{3}}=\frac{{9{{\lambda }^{2}}}}{{2{{a}_{0}}}}{{x}^{2}}
(c) {{y}^{2}}=\frac{{9{{\lambda }^{2}}}}{{2a_{0}^{2}}}{{x}^{2}}
(d) {{y}^{2}}=\frac{{2{{\lambda }^{2}}}}{{9{{a}_{0}}}}{{x}^{3}}
Ans (d)
6.
A particle moves in the x-y plane with velocity \vec{v}={{k}_{1}}\hat{i}+{{k}_{2}}x\hat{j}, where \hat{i}and \hat{j}are the unit vectors along the x and y axes and andare constants. At the initial moment, the particle was located at the point x = y = 0. The equation to the particle’s trajectory is
(a) y=\frac{{{{k}_{1}}}}{{2{{k}_{2}}}}{{x}^{2}}
(b) y=\frac{{{{k}_{2}}}}{{2{{k}_{1}}}}{{x}^{2}}
(c) y=\frac{{2{{k}_{1}}}}{{{{k}_{2}}}}{{x}^{2}}
(d) y=\frac{{2{{k}_{2}}}}{{{{k}_{1}}}}{{x}^{2}}
Ans (b)
7.
A ball is projected on a smooth inclined plane in a direction perpendicular to line of greatest slope with velocity of 8m/s. Find it’s speed (in m/s) after 1 sec.
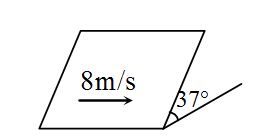
(a) 10 m/s
(b) 8 m/s
(c) 6 m/s
(d) 12 m/s
8.
Two particles are moving along two long straight lines, in the same plane, with the same speed = 20 cm/s. The angle between the two lines is 60°, and their intersection point is O. At a certain moment, the two particles are located at distances 3m and 4m from O, and are moving towards O. Find the shortest distance (in mm) between them subsequently. ( \sqrt{3}=1.73)
(a) 856mm
(b) 865mm
(c) 826mm
(d) 860.5mm
Ans (b)
9.
A train carriage moves along the X-axis with a uniform acceleration. An observer A in the train sets a ball in motion on frictionless floor of the carriage with a velocity relative to the carriage. The direction of ball makes an angle q with the X-axis. Let B be an observer standing on the ground outside the train. The subsequent path of the ball will be
(a) a straight line with respect to observer A
(b) parabolic with respect to observer B
(c) parabolic with respect to observer A
(d) none of these
Ans (c)
10.
A particle is released from a height of H = 400 m. Due to the wind the particle gathers the horizontal velocity {{v}_{x}}=ay where a=\surd 5 sec–1 and y is the vertical displacement of the particle from point of release. Find the horizontal drift (in m) of the particle when it strikes the ground.
(a) 2550m
(b)2670m
(c)2760m
(d) 1560m
Ans (b)
11.
Three particles A, B and C are thrown simultaneously with speeds {{v}_{A}},\,\,{{v}_{B}} and {{v}_{C}} with A horizontally, B at an angle of 60° with the horizontal and C vertically in such a manner that they collide simultaneously at H, the highest point of the parabolic path of B. If the acceleration due to gravity is g, then the ratio of the speeds {{v}_{A}}:{{v}_{B}}:{{v}_{C}} is
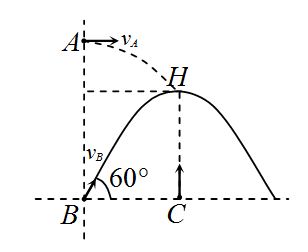
(a) 1 : 1 : 1
(b) 1 : 2 : \displaystyle \sqrt{{}}3
(c) \displaystyle \sqrt{{}}3 : 1 : 1
(d) \displaystyle \sqrt{{}}3 : 2 : 1
Ans (b)
12.
A golfer standing on level ground hits a ball with a velocity of u = 52 m/s at an angle a above the horizontal. If tan a = 5/12, then the time for which the ball is at least 15m above the ground (i.e. between A and B) will be (take g = 10 m/s2)
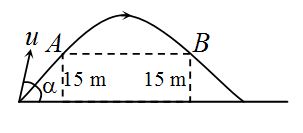
(a) 1 sec (b) 2 sec (c) 3 sec (d) 4 sec
Ans (b)
13.
A boy throws a ball upwards with a velocity 12 m/sec at a angle q with the vertical. The wind imparts a horizontal acceleration of 0.4 m/s2. If for angle q the ball thrown returns to the point of release, then find cot q.
(a)25 (b)35 (C)15 (d)27.5
Ans (a)
14.
A man can throw a stone with a speed of 10 m/s. The maximum horizontal distance to which he can throw the stone in a room of height 2m is \sqrt{x}. Find the value of x,
(a) 96 (b)86 (c) 75 (d)100
Ans (a)
15.
A particle is projected with a speed V from a point O making an angle of 30° with the vertical. At the same instant, a second particle is thrown vertically upwards from a point A. The two particles reach H, the highest point on the parabolic path of first particle simultaneously. The ratio \frac{V}{v} is
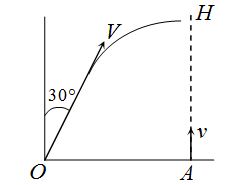
(a) 3\sqrt{2} (b) 2\sqrt{3} (c) \frac{2}{{\sqrt{3}}} (d) \frac{{\sqrt{3}}}{2}
Ans (c)
16.
A gun kept on a straight horizontal road, is used to hit a car, tavelling along the same road away from the gun with the uniform speed of 90 km/h. The car is at a distance of 600 m from the gun when shell is fired from the gun at an angle of 30° with the horizontal. Then the distance (in m) from the initial position of the car at which the shell will hit is close to
(a)150m
(b)125m
(c)250m
(d)225m
Ans (c)
17.
A gun is situated on an inclined plane. The maximum range up the inclined plane and down the inclined plane are 250 m and 750 m respectively. Find the angle of inclination (in degree) of the inclined plane
(a) 30o
(b) 40o
(c) 50o
(d) 80o
Ans (a)
18.
In the figure shown, the two projectiles are fired simultaneously. Find the minimum distance (in m) between them during their flight?
(a)10m
(b)5m
(c)20m
(d)12.5m

Ans (a)
Comprehension Based Question 19 and 20
Two guns, situated on the top of a hill of height 10 m, fire one shot each with the same speed 5 \sqrt{3} m/s at some interval of time. One gun fires horizontally and other fires upward at an angle of 60o with the horizontal. The shots collide in air at a point P. Find
19.
The time-interval between the firings
(a) 1 s
(b) 2 s
(c) 5 s
(d) 10 s
Ans (a)
20.
The coordinates of the point P.
Take the origin of the coordinate system at the foot of the hill right below the muzzles and consider the trajectories in the X-Y plane. (g = 10 m/s2).
(a) \left( {5\sqrt{3},\,\,5} \right)
(b) \left( {5\sqrt{3},\,\,3} \right)
(c) \left( {3\sqrt{5},\,\,3} \right)
(d) \left( {5\sqrt{7},\,\,10} \right)
Ans (a)
21.
Two bodies were thrown simultaneously from the same point; one straight up, and the other, at an angle of q = 30° to the horizontal. The initial velocity of each body is equal to
vo= 25 m/s. Neglecting the air drag, find the distance between the bodies t = 2 s later.
(a) 30m
(b) 10m
(c) 50m
(d) 15m
Ans (c)
22.
A cannon fires successively two shells with the same speed of 250 m/s; the first at an angle 60o and the second at 45o to the horizontal. The shells collide in mid air. Find the time interval between the firings. The trajectories of the shells lie in the same plane.
(a) 10s
(b) 5s
(c)11s
(d)20s
Ans (c)
Comprehension Based Question 23 and 24
A basketball player throws the ball with initial velocity vo at an angle q above the horizontal to the hoop which is located a horizontal distance L and at a height h above the point of release, as shown in figure.
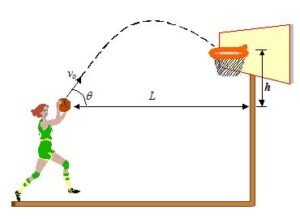
23.
The initial speed of projection must be
(a) v_{o}=\sqrt{\frac{{gL}}{{2{{{\cos }}^{2}}\theta (\tan \theta \,-\,h)}}}
(b) v_{o}=\sqrt{\frac{{g}}{{{{{\cos }}^{2}}\theta (\tan \theta \,-\,h/L)}}}
(c) v_{o}=\sqrt{\frac{{g}}{{2{{{\cos }}^{2}}\theta (\tan \theta \,-\,h/L)}}}
(d) v_{o}=\sqrt{\frac{{gL}}{{2{{{\cos }}^{2}}\theta (\tan \theta \,-\,h/L)}}}
Ans (d)
24.
The angle a to the horizontal at which it reaches the hoop is given by
(a) tan a = h/L+ tan q
(b) tan a = 2h/L+ tan q
(c) tan a = 2h/L– tan q
(d) tan a = h/L– tan q
Ans (c)
25.
A girl stands 4 m from a vertical wall and throws a ball. The ball leaves the girl’s hand at 2 m above the ground with initial velocity v= 10 \hat{i} + 10 \hat{j} m/s. When the ball hits the wall its horizontal component of velocity is reversed and its vertical component remains unchanged. Where does the ball hit the ground ?
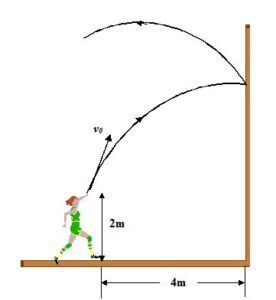
(a) 20 m behind the girl
(b) 13.8 m behind the girl
(c) 15.5 m behind the girl
(d) 10.6 m behind the girl
Ans (b)
Comprehension Based Question 26 and 27
On a frictionless horizontal surface, assumed to be the x – y plane, a small trolley A is moving along a straight line parallel to the y-axis (see figure) with a constant velocity of ( \sqrt{3}– 1)m/s. At a particular instant, when the line OA makes an angle of 45° with the x-axis, a ball is thrown along the surface from the origin O. Its velocity makes an angle f with the x-axis and it hits the trolley.
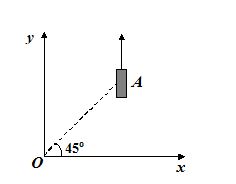
26.
The motion of the ball is observed from the frame of the trolley. Calculate the angle q made by the velocity vector of the ball with the x-axis in this frame.
(a) 55O with the x-axis
(b) 75O with the x-axis
(c) 45O with the x-axis
(d) 65O with the x-axis
Ans (c)
27.
Find the speed of the ball with respect to the surface. if f = 4q/3.
(a) 15 m/s
(b) 10 m/s
(c) 6 m/s
(d) 2 m/s
Ans (d)
Comprehension Based Question 28 and 29
A balloon starts rising from the surface of the earth. The vertical component of the balloon’s velocity is vo. Due to the wind the balloon gathers the horizontal velocity vx= ay, where a is a constant and y is the height from the surface of the earth. Find
28.
The equation of trajectory of the balloon.
(a) y2 = \frac{{2{{v}_{o}}}}{a}x
(b) y2 = \frac{{8{{v}_{o}}}}{a}y
(c)y2 = \frac{{4{{v}_{o}}}}{a}x
(d) y2= \frac{{7{{v}_{o}}}}{b}y
Ans (a)
29.
The tangential, normal and total acceleration of the balloon as function of y.
(a) at= \frac{{{{b}^{2}}y{{v}_{o}}}}{{\sqrt{{{{{\left( {ax} \right)}}^{2}}+v_{o}^{2}}}}} ; an = \frac{{av_{o}^{2}}}{{\sqrt{{{{{\left( {ay} \right)}}^{2}}+v_{o}^{2}}}}} ; atotal = a
(b) at= \frac{{{{a}^{3}}y{{v}_{o}}}}{{\sqrt{{{{{\left( {ay} \right)}}^{3}}+v_{o}^{3}}}}} ; an = \frac{{av_{o}^{2}}}{{\sqrt{{{{{\left( {ay} \right)}}^{2}}+v_{o}^{2}}}}} ; atotal = avo
(c) at= \frac{{{{a}^{2}}y{{v}_{o}}}}{{\sqrt{{{{{\left( {ay} \right)}}^{2}}+v_{o}^{2}}}}} ; an = \frac{{av_{o}^{2}}}{{\sqrt{{{{{\left( {ay} \right)}}^{2}}+v_{o}^{2}}}}} ; atotal = avo
(d) at= \frac{{{{a}^{4}}y{{v}_{o}}}}{{\sqrt{{{{{\left( {ay} \right)}}^{4}}+v_{o}^{4}}}}} ; an = \frac{{av_{o}^{2}}}{{\sqrt{{{{{\left( {ay} \right)}}^{2}}+v_{o}^{2}}}}} ; atotal = 2avo
Ans (C)